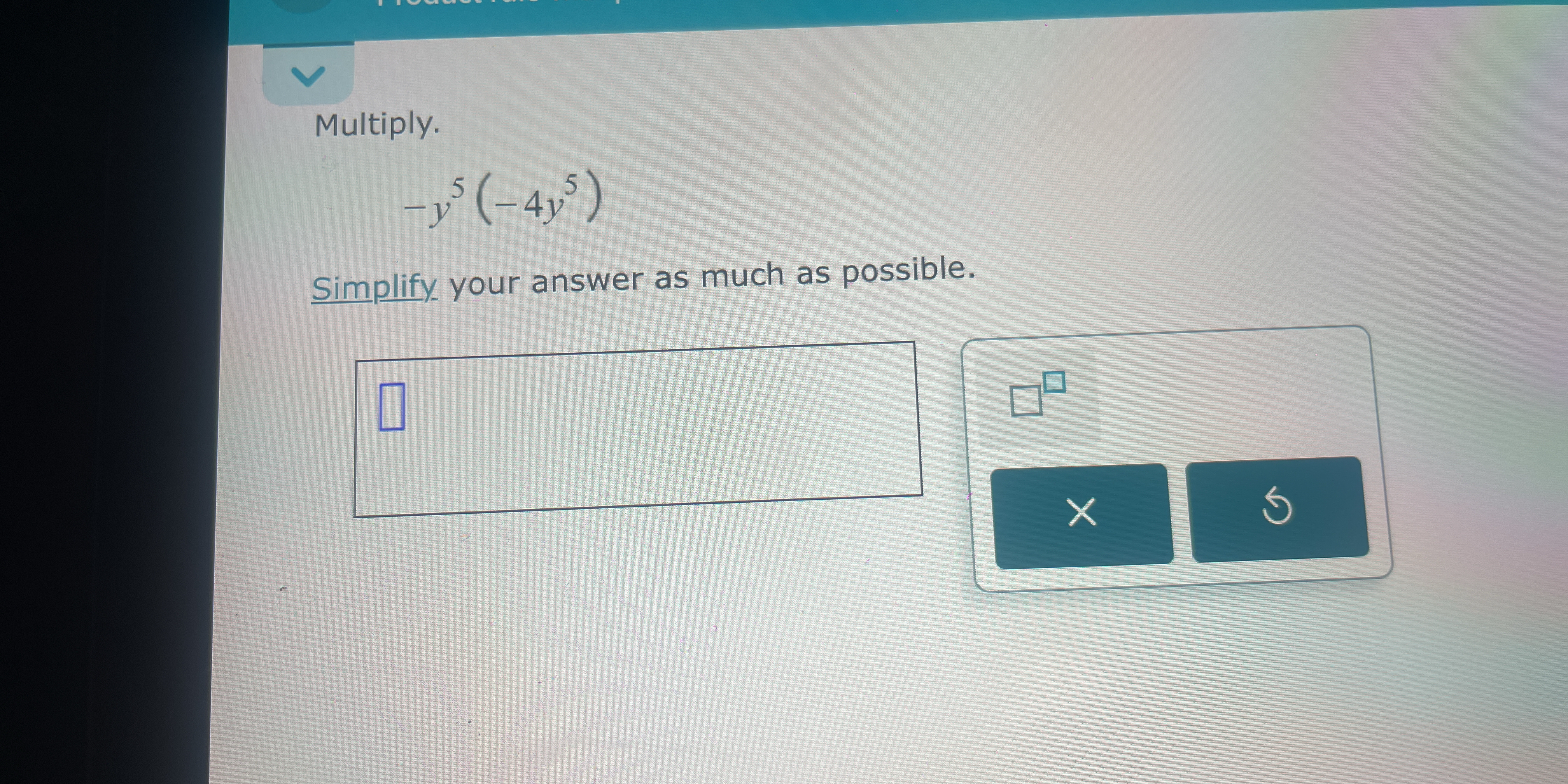
Multiply -y^5 by (4y^5) and simplify your answer as much as possible.
Understand the Problem
The question is asking to multiply the expression -y^5 by (4y^5) and simplify the result as much as possible.
Answer
The simplified expression is $-4y^{10}$.
Answer for screen readers
The simplified expression is $-4y^{10}$.
Steps to Solve
-
Identify the expression to multiply We have the expression $-y^5$ to be multiplied by $(4y^5)$.
-
Multiply the coefficients The coefficients are -1 (from $-y^5$) and 4 (from $4y^5$). Thus, we compute: $$ -1 \times 4 = -4 $$
-
Multiply the variable parts Next, we multiply $y^5$ by $y^5$. When multiplying terms with the same base, we add the exponents: $$ y^5 \times y^5 = y^{5+5} = y^{10} $$
-
Combine the results Now we combine the results of the coefficients and the variable parts: $$ -4 \times y^{10} = -4y^{10} $$
The simplified expression is $-4y^{10}$.
More Information
Multiplying expressions with exponents follows two main rules: multiply the coefficients and then add the exponents of like bases. This process helps in simplifying algebraic expressions efficiently.
Tips
- Forgetting to add the exponents when multiplying like bases.
- Miscalculating the multiplication of coefficients. Always double-check your arithmetic.
AI-generated content may contain errors. Please verify critical information