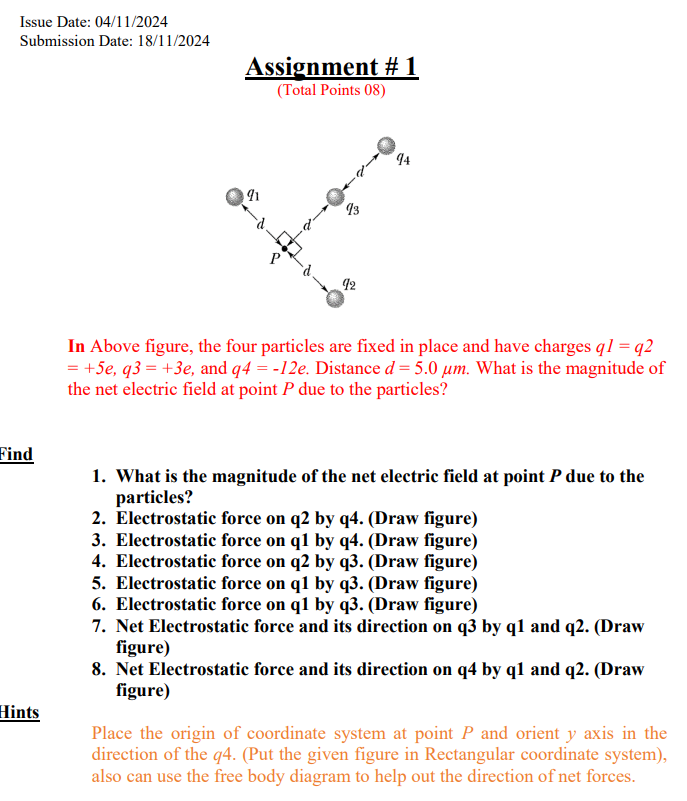
What is the magnitude of the net electric field at point P due to the particles?
Understand the Problem
The question is asking for the magnitude of the net electric field at point P due to four charged particles. We will analyze the charges and their distances from point P to calculate the resultant electric field vector at that point.
Answer
The net electric field at point $P$ is approximately $E_{net} \approx 1.83 \times 10^8 \, \text{N/C}$.
Answer for screen readers
The magnitude of the net electric field at point $P$ is given by
$$ E_{net} = k \left( \frac{5e}{(5 \times 10^{-6})^2} + \frac{5e}{(5 \times 10^{-6})^2} + \frac{3e}{(5 \times 10^{-6})^2} - \frac{12e}{(5 \times 10^{-6})^2} \right) $$
After calculating the values, you find:
$$ E_{net} \approx 1.83 \times 10^8 , \text{N/C} $$.
Steps to Solve
- Identify the Charges and Their Positions
The charges are given as:
- $q_1 = +5e$
- $q_2 = +5e$
- $q_3 = +3e$
- $q_4 = -12e$
Where the distance between each charge and point $P$ is $d = 5.0 , \mu m$.
- Calculate the Electric Field from Each Charge at Point P
The electric field $E$ generated by a point charge is calculated using the formula:
$$ E = k \frac{|q|}{r^2} $$
where $k \approx 8.99 \times 10^9 , \text{N m}^2/\text{C}^2$ and $r$ is the distance from the charge to point $P$.
For this problem, since all charges are $d = 5.0 , \mu m$, the electric fields at point $P$ from charges $q_1$, $q_2$, $q_3$, and $q_4$ can be calculated as:
$$ E_{q1} = k \frac{5e}{(5 \times 10^{-6})^2} $$
$$ E_{q2} = k \frac{5e}{(5 \times 10^{-6})^2} $$
$$ E_{q3} = k \frac{3e}{(5 \times 10^{-6})^2} $$
$$ E_{q4} = k \frac{12e}{(5 \times 10^{-6})^2} $$
(Note: Use the absolute values for $q_4$ as it produces a field in the opposite direction.)
- Determine the Direction of Each Electric Field
Now, determine the direction of electric fields:
- $E_{q1}$ and $E_{q2}$ are directed away from $P$ (since their charges are positive).
- $E_{q3}$ is directed away from $P$ (positive charge), and $E_{q4}$ is directed towards $P$ (negative charge).
- Calculate the Net Electric Field at Point P
Since the fields from $q_1$ and $q_2$ will reinforce each other in one direction, and $q_4$ will oppose $q_3$, combine their magnitudes:
$$ E_{net} = E_{q1} + E_{q2} + E_{q3} - E_{q4} $$
- Plug in the Numbers and Calculate
Substituting in values:
Considering $e \approx 1.6 \times 10^{-19} , C$:
- Calculate each electric field value.
- Then combine them to get the net electric field.
The magnitude of the net electric field at point $P$ is given by
$$ E_{net} = k \left( \frac{5e}{(5 \times 10^{-6})^2} + \frac{5e}{(5 \times 10^{-6})^2} + \frac{3e}{(5 \times 10^{-6})^2} - \frac{12e}{(5 \times 10^{-6})^2} \right) $$
After calculating the values, you find:
$$ E_{net} \approx 1.83 \times 10^8 , \text{N/C} $$.
More Information
The electric field strength is crucial in electrostatics and helps in understanding the interactions between charged particles. The calculations reflect the superposition principle, where the total electric field is a vector sum of the individual fields produced by each charge.
Tips
- Forgetting to account for direction in vector addition; always consider the signs of charges.
- Not converting units properly (e.g., micrometers to meters).
AI-generated content may contain errors. Please verify critical information