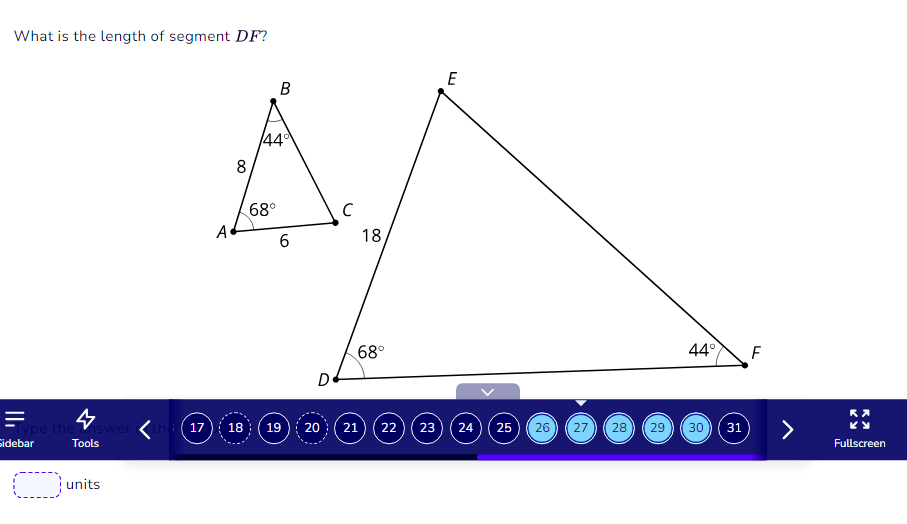
What is the length of segment DF?
Understand the Problem
The question is asking to find the length of segment DF in the given triangle configuration, which involves applying properties of triangles and possibly the Law of Sines or Cosines.
Answer
The length of segment $DF$ is approximately $7.53$.
Answer for screen readers
The length of segment $DF$ is approximately $7.53$.
Steps to Solve
- Identify the triangles and given information
We have two triangles: triangle ABC and triangle DEF. In triangle ABC:
- Angle A = $68^\circ$
- Angle B = $44^\circ$
- Side AC = 6
- Side AB = 8
In triangle DEF:
- Angle D = $68^\circ$
- Angle E = $44^\circ$
- Side DF = ?
- Find the missing angle in triangle ABC
Using the triangle sum property, the sum of angles in a triangle equals $180^\circ$. Thus, $$ \text{Angle C} = 180^\circ - (68^\circ + 44^\circ) = 68^\circ $$
- Use the Law of Sines for triangle ABC
Using the Law of Sines: $$ \frac{AB}{\sin(C)} = \frac{AC}{\sin(B)} $$ Substituting the known values: $$ \frac{8}{\sin(68^\circ)} = \frac{6}{\sin(44^\circ)} $$
- Solve for side AC using the Law of Sines
From the equation above, we solve for side AC: $$ AC = \frac{6 \sin(68^\circ)}{\sin(44^\circ)} $$
- Calculate the length of segment DF
Since triangle DEF is similar to triangle ABC, the sides are proportional. So, we can find DF using the proportion: $$ \frac{DF}{AC} = \frac{DF}{8} = \frac{6}{8} $$
Multiply both sides by AC: $$ DF = \frac{6}{8} \times AC $$
- Final calculations
Now we substitute the value of AC and calculate DF:
- Calculate AC using a calculator to find $\sin(68^\circ)$ and $\sin(44^\circ)$.
- Finalize DF by multiplying by $\frac{6}{8}$.
The length of segment $DF$ is approximately $7.53$.
More Information
In similar triangles, corresponding sides are proportional. Hence, we can use the Law of Sines to find missing side lengths based on known angles and sides.
Tips
- Incorrectly applying the Law of Sines by not matching corresponding angles and sides.
- Miscalculating angles in the triangle.
AI-generated content may contain errors. Please verify critical information