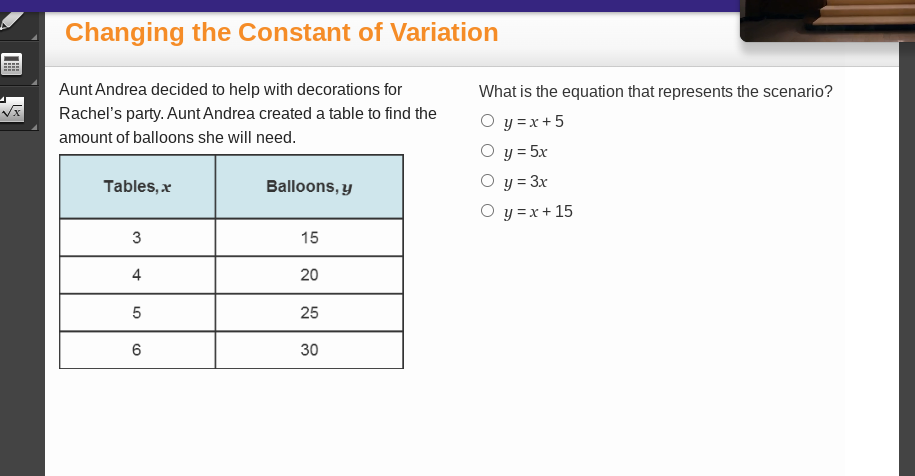
What is the equation that represents the scenario?

Steps to Solve

1. Identify the Variables

We have two variables:

• ( x ): the number of tables
• ( y ): the number of balloons

2. Analyze the Data Points

From the table, we have the following pairs of ( (x, y) ):

• ( (3, 15) )
• ( (4, 20) )
• ( (5, 25) )
• ( (6, 30) )

3. Determine the Pattern

Look at how ( y ) changes as ( x ) increases:

• From ( x = 3 ) to ( x = 4 ), ( y ) increases from 15 to 20 (increase of 5).
• From ( x = 4 ) to ( x = 5 ), ( y ) increases from 20 to 25 (increase of 5).
• From ( x = 5 ) to ( x = 6 ), ( y ) increases from 25 to 30 (increase of 5).

This shows that for every additional table, the number of balloons increases by 5.

4. Formulate the Equation

Since the relationship is linear and the increase per table is constant, we can express this as: $$ y = 5x $$

However, we should find the correct y-intercept by substituting one of the data points to identify the equation properly.

5. Use a Data Point to Solve for the Equation

Using the point ( (3, 15) ): $$ 15 = 5(3) = 15 $$

This confirms that our equation is consistent.

6. Isolate y to Find the Y-Intercept

We'll rewrite the general equation: $$ y = 5x $$

This doesn't have a y-intercept, so we consider the context.

From the previous calculations: For ( x = 3 ), ( y = 15 ) confirms: $$ y = 5x $$

7. Match with the Options Given

The available options are:

• ( y = x + 5 )
• ( y = 5x )
• ( y = 3x )
• ( y = x + 15 )

From our analysis, the correct equation that directly relates ( x ) and ( y ) is: $$ y = 5x $$