What is the derivative of y = f(x) at the point x = x0, and how is it defined as a limit?

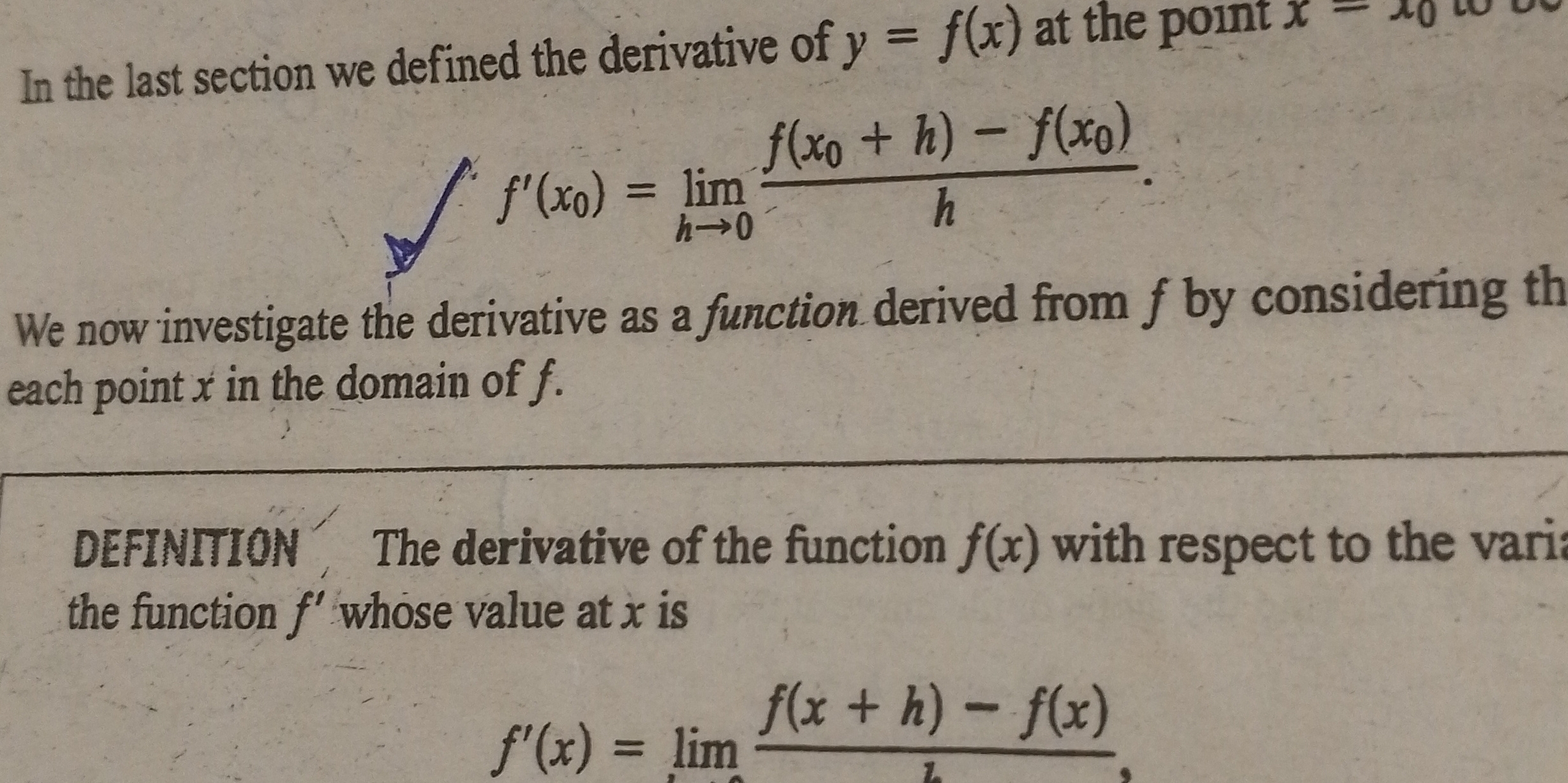
Understand the Problem

The question relates to the definition of the derivative of a function and how it is expressed as a limit. It addresses the concept of investigating the derivative as a function itself, derived from the original function.

Answer

The derivative of the function is given by $f'(x) = \lim_{h \to 0} \frac{f(x + h) - f(x)}{h}$.

Steps to Solve

Understanding the Derivative Definition The derivative of a function $f(x)$ at a specific point $x = x_0$ is defined as: $$ f'(x_0) = \lim_{h \to 0} \frac{f(x_0 + h) - f(x_0)}{h} $$ This expression represents the slope of the tangent line to the function at that point.
Generalizing the Concept We extend the derivative definition to a function $f'(x)$ that gives the slope at any point $x$ in the domain of $f$. Thus, we have: $$ f'(x) = \lim_{h \to 0} \frac{f(x + h) - f(x)}{h} $$ This means we can compute the derivative at any point along the curve of $f$.
Expression for $f'(x)$ This leads to a general expression for the derivative of the function $f(x)$, where in this definition we replace $x_0$ with $x$, capturing the behavior of the function around $x$ itself.

The derivative of the function $f(x)$ with respect to $x$ is given by: $$ f'(x) = \lim_{h \to 0} \frac{f(x + h) - f(x)}{h} $$

More Information

This expression signifies how quickly the function $f(x)$ is changing at any given point $x$ in its domain. Understanding derivatives is crucial in calculus as they help us analyze rates of change and motion.

Tips

Confusing the point of evaluation when computing derivatives. Always ensure you're substituting the correct point when evaluating $f(x_0)$ versus $f(x)$.
Omitting the limit process. The derivative definition inherently relies on taking the limit as $h$ approaches 0.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!