What is Thales's theorem in geometry?
Understand the Problem
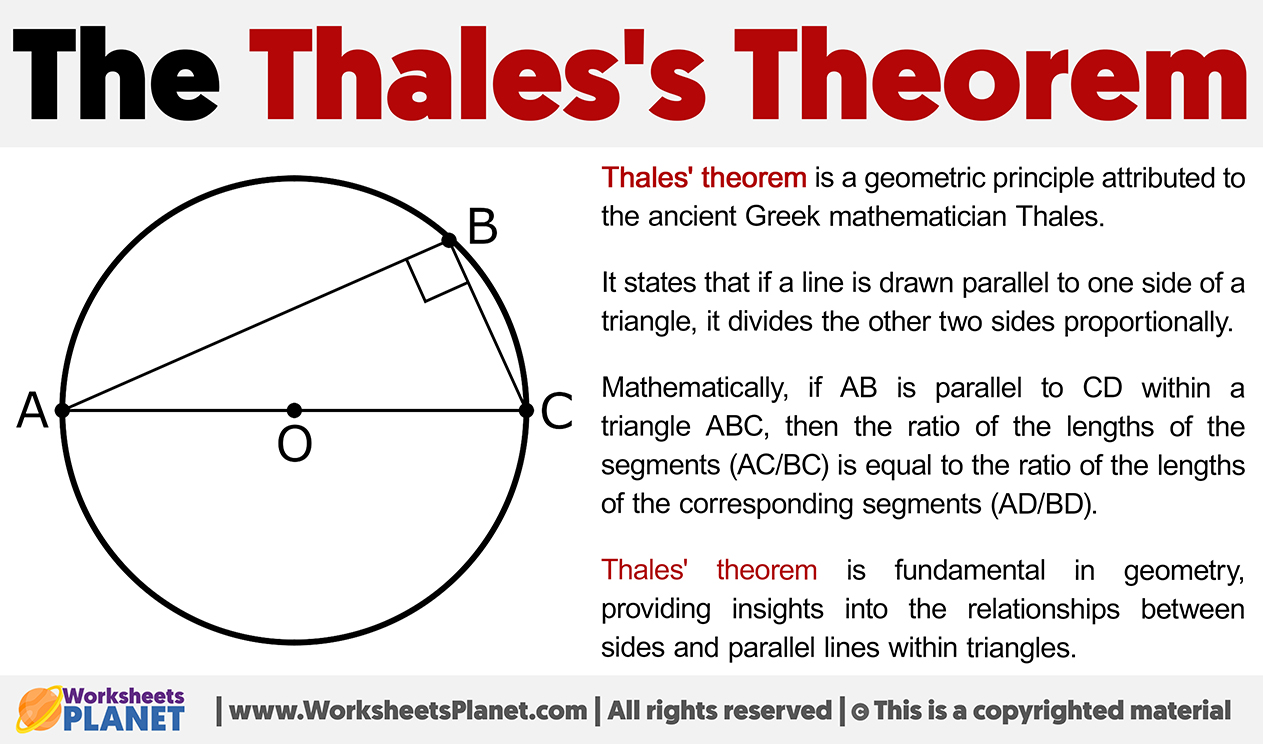
The question is explaining Thales's theorem, which is a fundamental concept in geometry, focusing on the proportionality of segments created by a line drawn parallel to one side of a triangle.
Answer
$AD = 2$
Answer for screen readers
The value of $AD$ is $2$.
Steps to Solve
-
Understanding Thales's Theorem Thales's Theorem states that if a line is drawn parallel to one side of a triangle, it divides the other two sides proportionally. If we have triangle $ABC$ and a line $AB$ drawn parallel to side $AC$, then the segments formed on the opposite side are proportional.
-
Identifying Proportional Segments Let’s denote the segments formed by the parallel lines: if $AB \parallel CD$, then we have segments $AC$, $BC$, $AD$, and $BD$. The theorem tells us that: $$ \frac{AC}{BC} = \frac{AD}{BD} $$
-
Setting Up a Problem Example For instance, if we know the lengths of $AC = 3$, $BC = 6$, and we need to find $AD$ when $BD = 4$, we can set up the proportion based on the theorem.
-
Applying the Proportion Using our proportions, we substitute the known values: $$ \frac{3}{6} = \frac{AD}{4} $$ Now, we can cross-multiply to solve for $AD$: $$ 3 \cdot 4 = 6 \cdot AD $$
-
Solving for the Unknown This simplifies to: $$ 12 = 6 \cdot AD $$ Dividing both sides by 6 gives: $$ AD = 2 $$
-
Conclusion Thus, by applying Thales's Theorem, we have determined that $AD = 2$.
The value of $AD$ is $2$.
More Information
Thales's Theorem is widely used in geometry, particularly in problems involving triangles and parallel lines. It showcases how geometric properties can lead to proportional relationships, making it an essential theorem for both basic and advanced geometric studies.
Tips
- Forgetting that the segments must be proportional based on the parallel lines can lead to incorrect calculations.
- Confusing the segments' labels, which can result in setting up the wrong proportions.
AI-generated content may contain errors. Please verify critical information