What are the assessment topics related to mathematics?
Understand the Problem
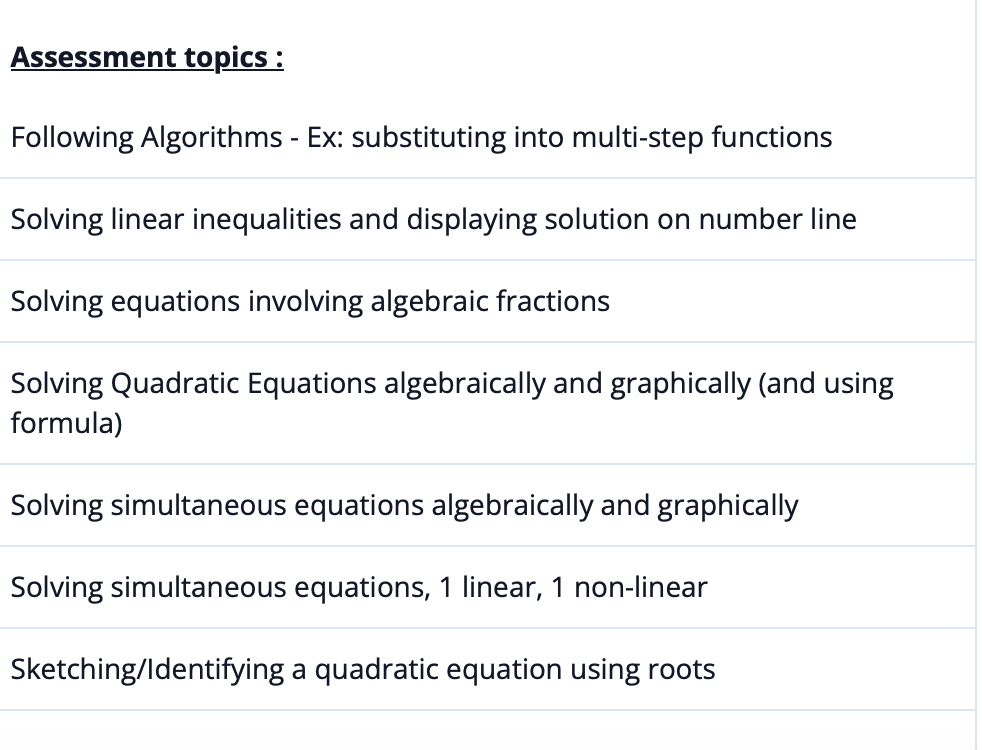
The image contains a list of assessment topics related to mathematical concepts. It includes algorithms, linear inequalities, algebraic fractions, quadratic equations, simultaneous equations, and sketching quadratic equations. The question likely pertains to understanding or explaining these assessment topics.
Answer
The assessment topics include algorithms, solving linear inequalities, algebraic fractions, quadratic equations, simultaneous equations, and sketching quadratics using roots.
Answer for screen readers
The assessment topics cover a variety of mathematical concepts including algorithms, inequalities, algebraic fractions, and different methods for solving quadratic and simultaneous equations. These skills are foundational for grasping more complex mathematical principles.
Steps to Solve
-
Understanding Algorithms in Mathematics
Begin by identifying and reviewing algorithms for substituting values into multi-step functions. This usually involves evaluating expressions step by step.
-
Solving Linear Inequalities
To solve linear inequalities, isolate the variable on one side of the inequality. For example, if you have $2x + 3 < 7$, subtract 3 from both sides to get $2x < 4$, and then divide by 2 to find $x < 2$. Displaying the solution on a number line involves marking the value where the inequality holds true.
-
Working with Algebraic Fractions
When solving equations involving algebraic fractions, ensure you have a common denominator. For example, to solve $\frac{1}{x} + \frac{2}{x} = 3$, combine to get $\frac{3}{x} = 3$. Cross-multiply to solve for $x$.
-
Solving Quadratic Equations
Quadratic equations can be solved using the quadratic formula, $x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$, or by factoring. Graphically, plot the equation and identify the x-intercepts.
-
Simultaneous Equations
Simultaneous equations can be solved either algebraically (e.g., substitution or elimination methods) or graphically by finding points where the lines intersect.
-
Sketching Quadratic Equations
To sketch or identify a quadratic equation using its roots, use the roots (x-intercepts) to plot on a coordinate plane. For the equation $y = a(x - r_1)(x - r_2)$, where $r_1$ and $r_2$ are the roots.
The assessment topics cover a variety of mathematical concepts including algorithms, inequalities, algebraic fractions, and different methods for solving quadratic and simultaneous equations. These skills are foundational for grasping more complex mathematical principles.
More Information
Understanding these topics not only helps in performing mathematical calculations but also in developing critical thinking and problem-solving skills. Each concept builds on others, forming a comprehensive mathematical foundation.
Tips
- Failing to properly isolate variables when solving linear inequalities.
- Forgetting to find common denominators when working with algebraic fractions.
- Misapplying the quadratic formula or neglecting to check if the discriminant is positive, negative, or zero.
AI-generated content may contain errors. Please verify critical information