Use the information in the diagram to determine the height of the tree.
Understand the Problem
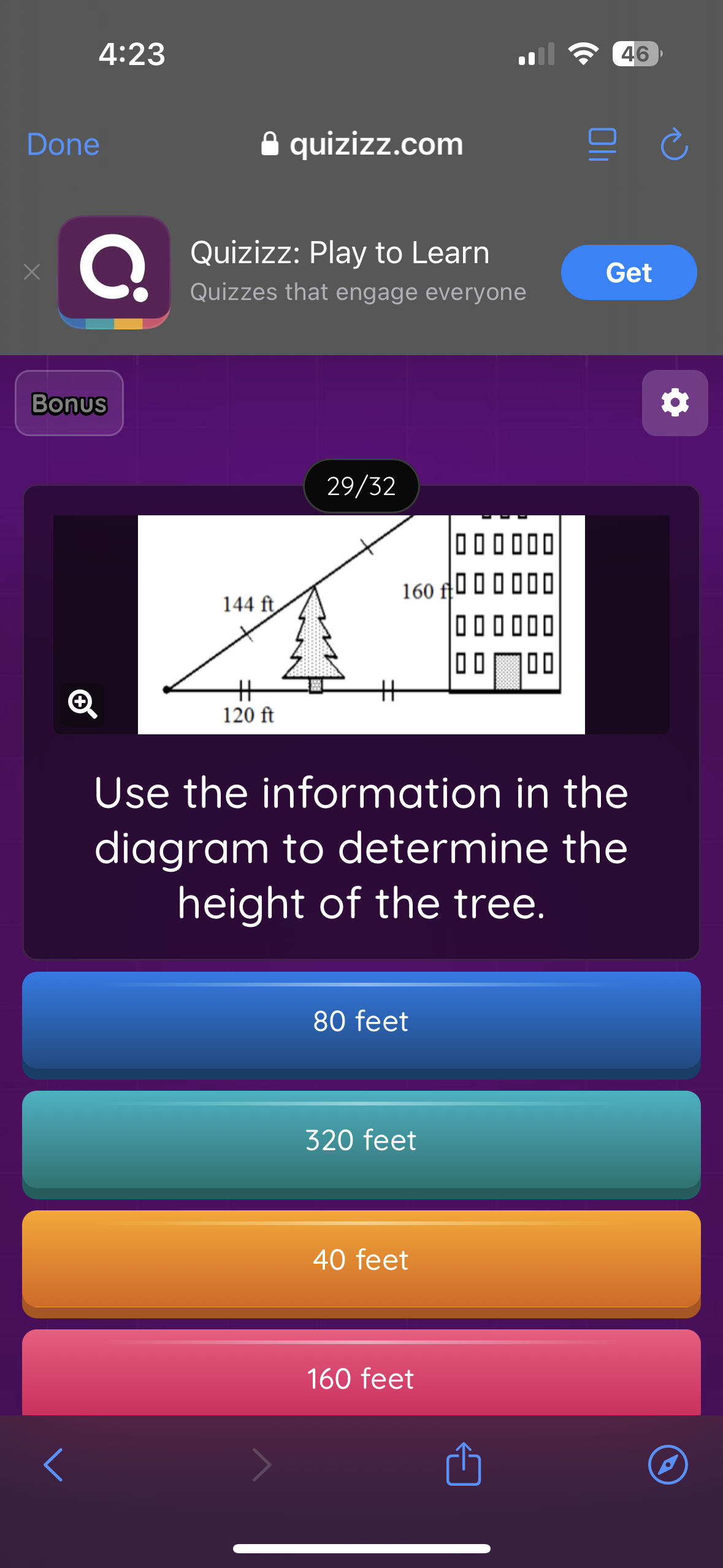
The question is asking to determine the height of a tree using given geometric information from the diagram, including certain distances indicated in feet. It involves applying concepts from geometry, likely involving right triangles.
Answer
The height of the tree is approximately $80$ feet.
Answer for screen readers
The height of the tree is approximately $80$ feet.
Steps to Solve
-
Identify the Right Triangle The diagram forms a right triangle with the tree height as one leg, the distance from the base of the tree to the building as the other leg, and the line from the top of the tree to the observer as the hypotenuse.
-
Label the Known Distances From the diagram:
- The distance from the tree to the building (horizontal leg) is given as 120 ft.
- The distance from the observer to the top of the tree (hypotenuse) is 144 ft.
- The vertical height of the building does not factor into the calculation for the tree height.
-
Apply the Pythagorean Theorem Using the Pythagorean theorem: $$ a^2 + b^2 = c^2 $$ Let ( a ) be the height of the tree, ( b = 120 ) ft, and ( c = 144 ) ft.
-
Set Up the Equation Substituting the known values into the equation: $$ a^2 + 120^2 = 144^2 $$
-
Calculate the Squares Calculate ( 120^2 ) and ( 144^2 ): $$ 120^2 = 14400 $$ $$ 144^2 = 20736 $$
-
Solve for ( a^2 ) Now, substituting the values: $$ a^2 + 14400 = 20736 $$ Rearranging gives: $$ a^2 = 20736 - 14400 $$
-
Calculate ( a^2 ) Perform the subtraction: $$ a^2 = 6336 $$
-
Find ( a ) Take the square root to find ( a ): $$ a = \sqrt{6336} $$
-
Calculate the Height Using a calculator: $$ a \approx 79.6 \text{ feet} $$
The height of the tree is approximately $80$ feet.
More Information
The height of the tree is found using the Pythagorean theorem, which relates the sides of a right triangle. In this case, the calculations provide the height of the tree based on the distances given in the diagram.
Tips
- Forgetting to use the right triangle relationship: Ensure you're correctly identifying the triangle formed and using the appropriate sides.
- Not squaring the values correctly: Pay attention to the arithmetic involved in squaring the lengths.
- Misinterpreting the diagram information: All given lengths should be correctly attributed to either the height, base, or hypotenuse of the triangle.
AI-generated content may contain errors. Please verify critical information