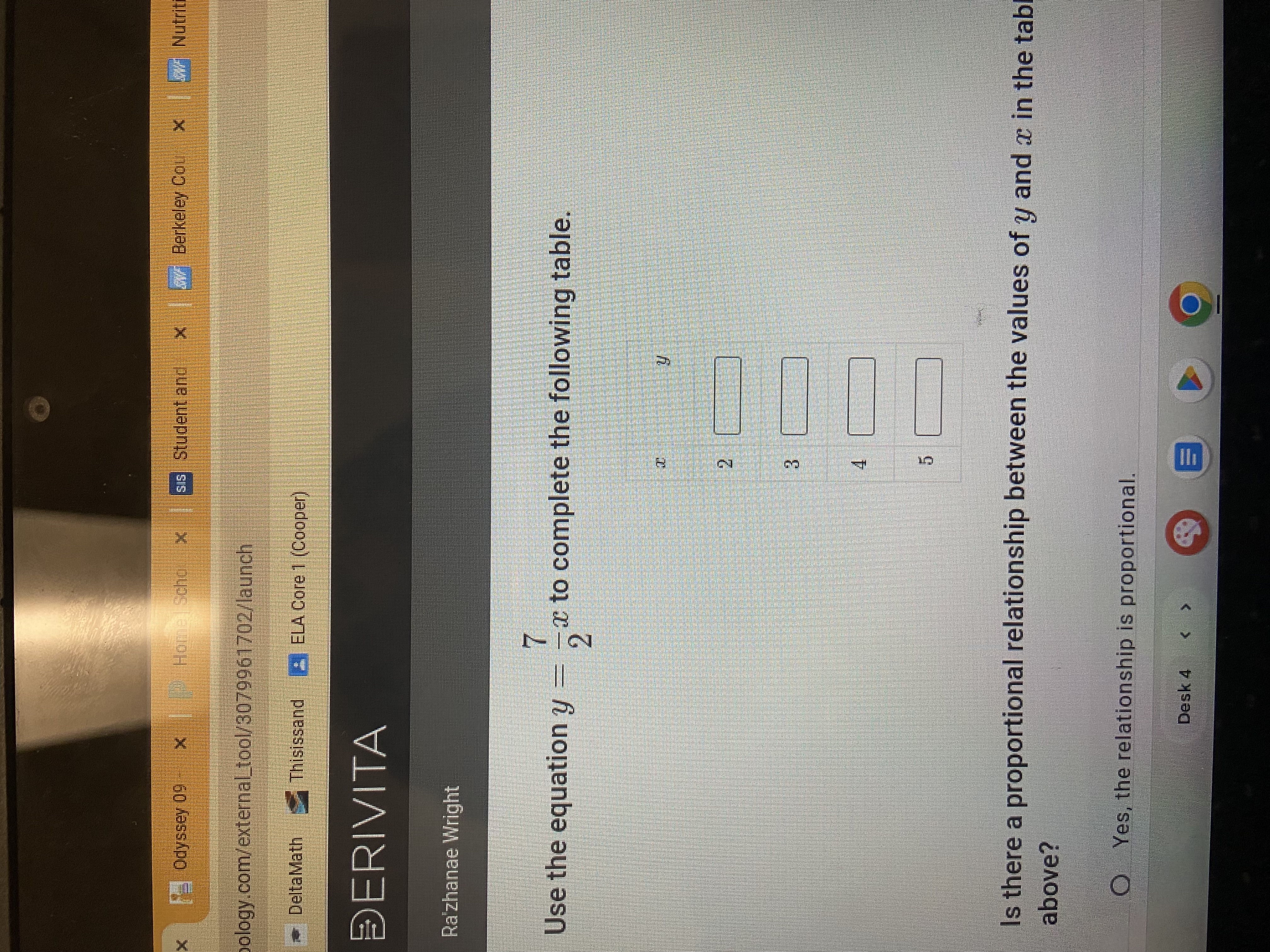
Use the equation y = 7/2 x to complete the following table. Is there a proportional relationship between the values of y and x in the table above?
Understand the Problem
The question is asking to use the equation y = (7/2)x to complete the table with specific x values and determine if there is a proportional relationship between y and x.
Answer
The table is completed as follows: | x | y | |-----|-------| | 2 | 7 | | 3 | 10.5 | | 4 | 14 | | 5 | 17.5 | Yes, the relationship is proportional.
Answer for screen readers
The completed table is:
| x | y |
|---|---|
| 2 | 7 |
| 3 | 10.5 |
| 4 | 14 |
| 5 | 17.5 |
Yes, the relationship is proportional.
Steps to Solve
- Calculate Values of y for Each x
To complete the table, substitute the given values of $x$ into the equation $y = \frac{7}{2}x$.
-
For $x = 2$:
$$ y = \frac{7}{2} \cdot 2 = 7 $$ -
For $x = 3$:
$$ y = \frac{7}{2} \cdot 3 = \frac{21}{2} = 10.5 $$ -
For $x = 4$:
$$ y = \frac{7}{2} \cdot 4 = 14 $$ -
For $x = 5$:
$$ y = \frac{7}{2} \cdot 5 = \frac{35}{2} = 17.5 $$
- Complete the Table
Fill in the table with the calculated values:
| x | y |
|---|---|
| 2 | 7 |
| 3 | 10.5 |
| 4 | 14 |
| 5 | 17.5 |
- Determine the Proportional Relationship
To check if there is a proportional relationship between $y$ and $x$, we need to see if the ratio $\frac{y}{x}$ remains constant.
-
Calculate the ratios:
-
For $x = 2, y = 7$:
$$ \frac{y}{x} = \frac{7}{2} = 3.5 $$ -
For $x = 3, y = 10.5:
$$ \frac{y}{x} = \frac{10.5}{3} = 3.5 $$ -
For $x = 4, y = 14:
$$ \frac{y}{x} = \frac{14}{4} = 3.5 $$ -
For $x = 5, y = 17.5:
$$ \frac{y}{x} = \frac{17.5}{5} = 3.5 $$
-
Since the ratios are all equal, there is a proportional relationship.
The completed table is:
| x | y |
|---|---|
| 2 | 7 |
| 3 | 10.5 |
| 4 | 14 |
| 5 | 17.5 |
Yes, the relationship is proportional.
More Information
The relationship $y = \frac{7}{2}x$ indicates that $y$ increases linearly as $x$ increases. The constant ratio $\frac{y}{x} = 3.5$ confirms proportionality. In proportion, as $x$ doubles, $y$ also doubles.
Tips
- Forgetting to multiply correctly when substituting $x$ into the equation.
- Miscalculating the ratios when checking for proportionality. Always simplify the ratios carefully.