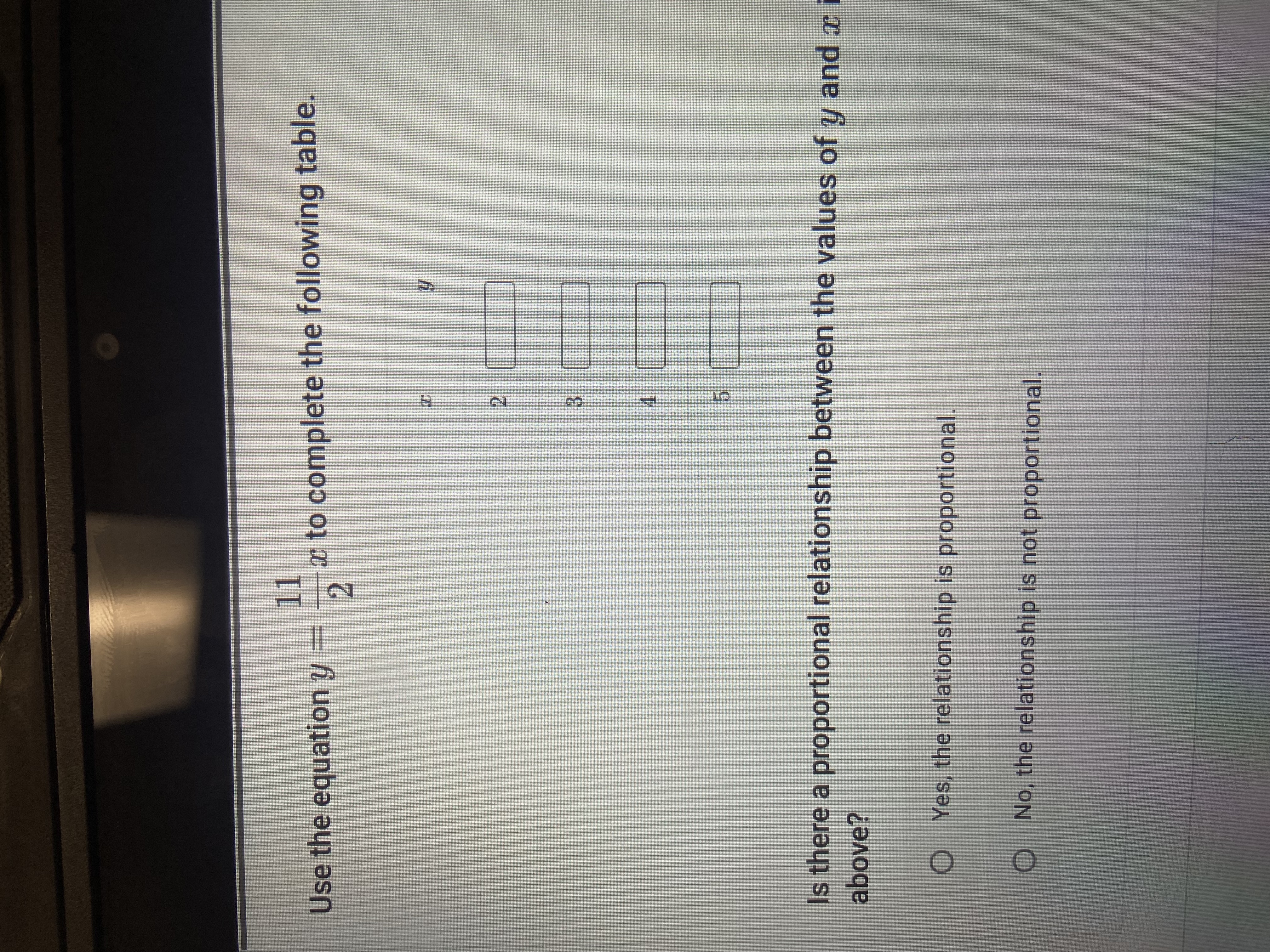
Use the equation y = (11/2)x to complete the following table. Is there a proportional relationship between the values of y and x?
Understand the Problem
The question is asking to use the equation y = (11/2)x to calculate the values of y for given x values (2 through 5) and then determine if there is a proportional relationship between y and x based on the equation provided.
Answer
Yes, the relationship is proportional.
Answer for screen readers
The completed table of values is:
| x | y |
|---|---|
| 2 | 11 |
| 3 | 16.5 |
| 4 | 22 |
| 5 | 27.5 |
Yes, the relationship is proportional.
Steps to Solve
-
Calculate $y$ for $x = 2$
Substitute $x = 2$ into the equation $y = \frac{11}{2}x$.
$$ y = \frac{11}{2} \cdot 2 = 11 $$
-
Calculate $y$ for $x = 3$
Now, substitute $x = 3$ into the equation.
$$ y = \frac{11}{2} \cdot 3 = \frac{33}{2} = 16.5 $$
-
Calculate $y$ for $x = 4$
Next, substitute $x = 4$ into the equation.
$$ y = \frac{11}{2} \cdot 4 = 22 $$
-
Calculate $y$ for $x = 5$
Finally, substitute $x = 5$ into the equation.
$$ y = \frac{11}{2} \cdot 5 = \frac{55}{2} = 27.5 $$
-
Evaluate the proportional relationship
A proportional relationship implies that the ratio of $y$ to $x$ is constant. Let's check the ratios:
- For $x = 2, y = 11$, ratio $= \frac{11}{2} = 5.5$
- For $x = 3, y = 16.5$, ratio $= \frac{16.5}{3} = 5.5$
- For $x = 4, y = 22$, ratio $= \frac{22}{4} = 5.5$
- For $x = 5, y = 27.5$, ratio $= \frac{27.5}{5} = 5.5$
Since all ratios are equal, $y$ and $x$ are proportional.
The completed table of values is:
| x | y |
|---|---|
| 2 | 11 |
| 3 | 16.5 |
| 4 | 22 |
| 5 | 27.5 |
Yes, the relationship is proportional.
More Information
The linear equation $y = \frac{11}{2}x$ indicates a direct relationship between $y$ and $x$, which signifies that for every increase in $x$, there is a corresponding proportional increase in $y$. This type of relationship is typical in linear functions.
Tips
- Mixing up the order of operations. Always calculate based on the equation provided.
- Forgetting to simplify fractions properly. Ensure that all fractions are presented in their simplest form.
