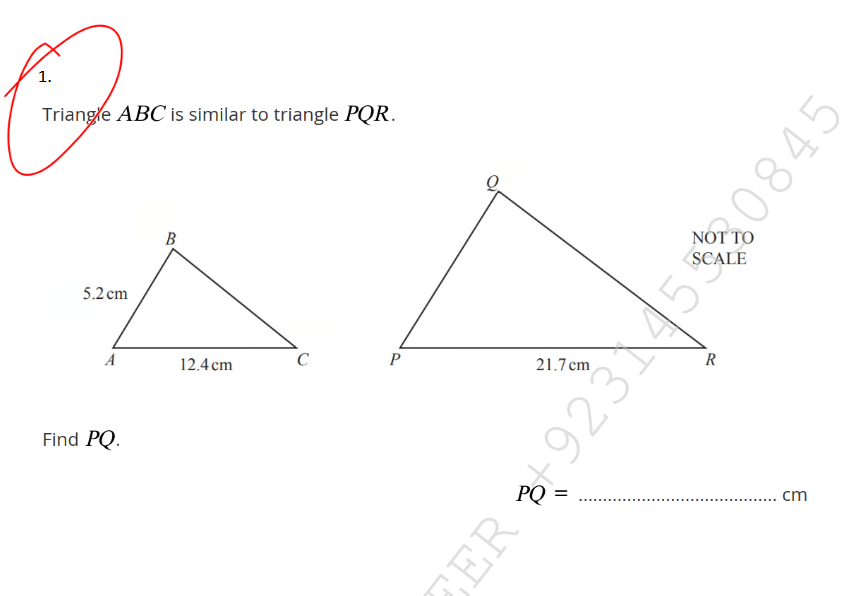
Triangle ABC is similar to triangle PQR. Find PQ.

Understand the Problem

The question involves finding the length of side PQ in triangle PQR based on the similarity between triangles ABC and PQR. The corresponding sides can be used to set up a proportion for solving the problem.

Answer

$PQ = 9.05 \, \text{cm}$

Steps to Solve

Identify Corresponding Sides Since triangles ABC and PQR are similar, corresponding sides are proportional. We identify the sides:
- ( AB ) corresponds to ( PQ )
- ( AC ) corresponds to ( PR )
- ( BC ) corresponds to ( QR )
Set Up the Proportion Using the lengths given:
- ( AB = 5.2 , \text{cm} )
- ( AC = 12.4 , \text{cm} )
- ( PR = 21.7 , \text{cm} )

We set up the proportion: $$ \frac{AB}{PQ} = \frac{AC}{PR} $$

Substitute the Values Substituting the known values into the proportion: $$ \frac{5.2}{PQ} = \frac{12.4}{21.7} $$
Cross Multiply Cross-multiplying gives: $$ 5.2 \times 21.7 = 12.4 \times PQ $$
Calculate the Left Side Calculating the left side: $$ 5.2 \times 21.7 = 112.24 $$
Solve for PQ Now we have: $$ 112.24 = 12.4 \times PQ $$

Dividing both sides by 12.4: $$ PQ = \frac{112.24}{12.4} $$

Final Calculation Calculating the final value for ( PQ ): $$ PQ = 9.05 , \text{cm} $$

$PQ = 9.05 , \text{cm}$

More Information

The similarity of triangles allows us to use the property of proportional sides, which is useful for various applications in geometry, including real-world measurements and scaling.

Tips

Forgetting to Cross Multiply: Make sure to cross multiply correctly when setting up proportions.
Misidentifying Corresponding Sides: Always double-check that you correctly identify the corresponding sides of the similar triangles.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!