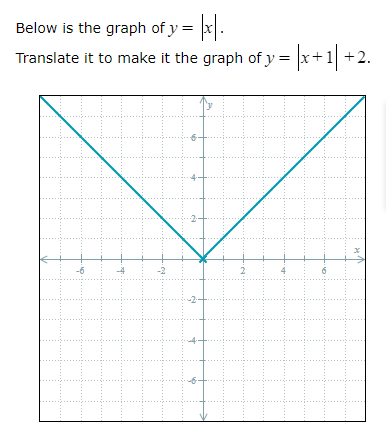
Translate the graph of y = |x| to make it the graph of y = |x + 1| + 2.
Understand the Problem
The question is asking how to apply transformations to the graph of the absolute value function to obtain the graph of a modified absolute value function. Specifically, it's asking to shift the graph of y = |x| to represent y = |x + 1| + 2, which involves translating the graph horizontally and vertically.
Answer
The vertex of the graph for $y = |x + 1| + 2$ is at the point (-1, 2).
Answer for screen readers
The transformed graph of $y = |x + 1| + 2$ has its vertex at the point (-1, 2).
Steps to Solve
-
Identify the Original Function The original function is $y = |x|$. This is the standard absolute value function, which has a vertex at the origin (0,0) and opens upwards.
-
Apply Horizontal Shift To transform the graph of $y = |x|$ to $y = |x + 1|$, we need to apply a horizontal shift. The expression $|x + 1|$ means we shift the graph 1 unit to the left. Thus, the vertex moves from (0,0) to (-1,0).
-
Apply Vertical Shift Next, we need to apply a vertical shift to get from $y = |x + 1|$ to $y = |x + 1| + 2$. The $+2$ indicates that we shift the entire graph upwards by 2 units. Therefore, the vertex moves from (-1,0) to (-1,2).
The transformed graph of $y = |x + 1| + 2$ has its vertex at the point (-1, 2).
More Information
The transformations of absolute value functions involve shifting the graph horizontally and vertically based on the modifications made to the equation. In this case, a leftward shift by 1 unit and an upward shift by 2 units were applied.
Tips
- Confusing horizontal and vertical shifts: Remember that $|x + c|$ shifts horizontally, while adding a constant outside the absolute value shifts vertically.
- Not adjusting the vertex correctly: Ensure you track the changes to the vertex position after each transformation.
AI-generated content may contain errors. Please verify critical information