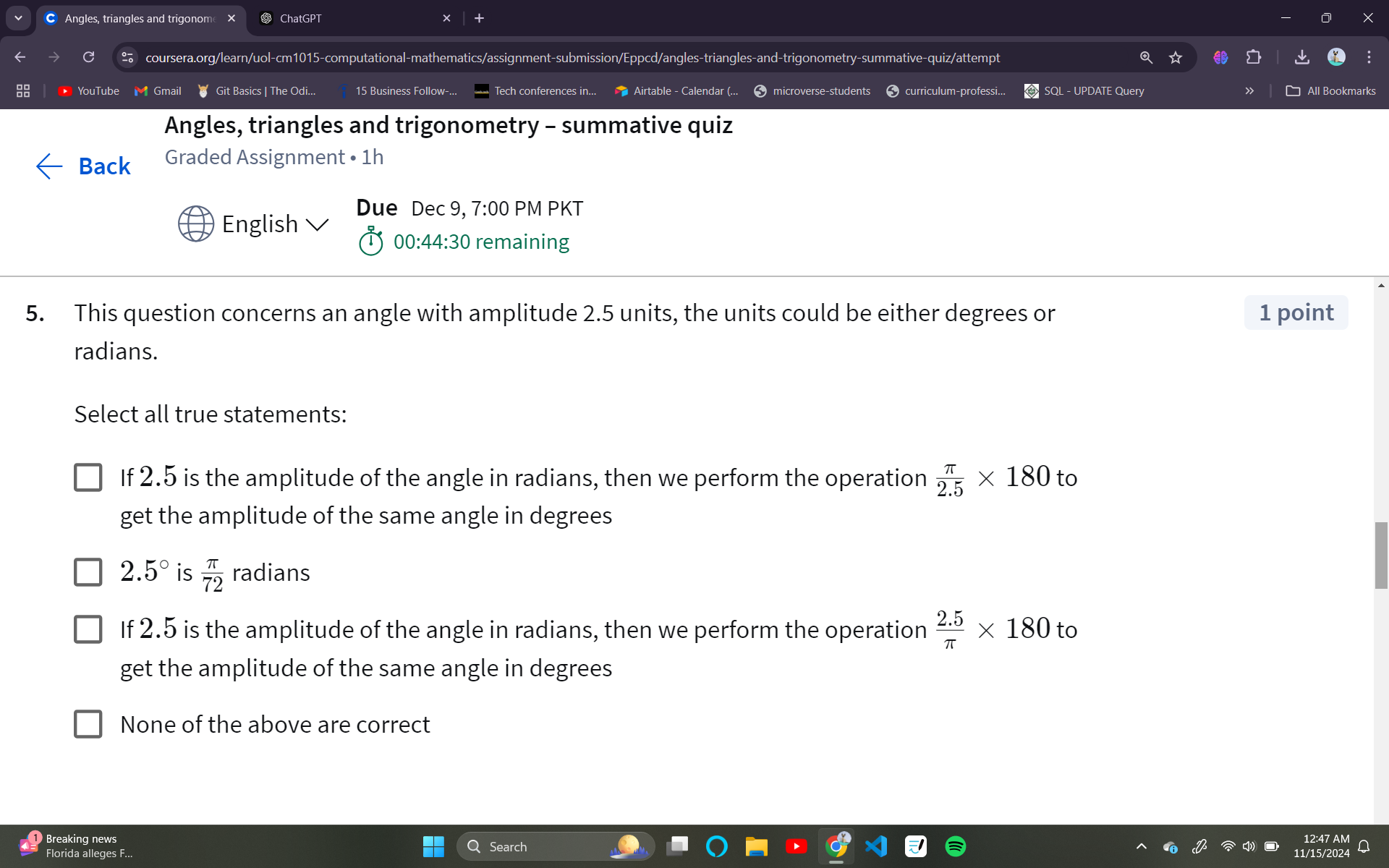
This question concerns an angle with amplitude 2.5 units, the units could be either degrees or radians. Select all true statements: If 2.5 is the amplitude of the angle in radians,... This question concerns an angle with amplitude 2.5 units, the units could be either degrees or radians. Select all true statements: If 2.5 is the amplitude of the angle in radians, then we perform the operation π/2.5 × 180 to get the amplitude of the same angle in degrees. 2.5° is π/72 radians. If 2.5 is the amplitude of the angle in radians, then we perform the operation 2.5/π × 180 to get the amplitude of the same angle in degrees. None of the above are correct. Read more

Steps to Solve

1. Understanding Radian to Degree Conversion

To convert radians to degrees, we use the formula: $$ \text{Degrees} = \text{Radians} \times \frac{180}{\pi} $$

2. Evaluating Statement 1

The first statement reads: "If 2.5 is the amplitude of the angle in radians, then we perform the operation $\frac{\pi}{2.5} \times 180$ to get the amplitude of the same angle in degrees."

Using the conversion formula: $$ 2.5 \text{ radians} \times \frac{180}{\pi} $$ This indicates that the statement is incorrect because the operation given does not equal the correct conversion.

3. Evaluating Statement 2

The second statement is "2.5° is $\frac{\pi}{72}$ radians."

To check the validity, we can convert 2.5 degrees to radians: $$ 2.5 \times \frac{\pi}{180} = \frac{2.5\pi}{180} = \frac{\pi}{72} $$ This indicates that this statement is true.

4. Evaluating Statement 3

The third statement states: "If 2.5 is the amplitude of the angle in radians, then we perform the operation $\frac{2.5}{\pi} \times 180$ to get the amplitude of the same angle in degrees."

We can check this: Using the correct conversion, $$ 2.5 \text{ radians} \times \frac{180}{\pi} $$ This is instead $\frac{2.5 \times 180}{\pi}$, thus this statement is also incorrect.

5. Final Evaluation

The only true statement is statement 2, "2.5° is $\frac{\pi}{72}$ radians."