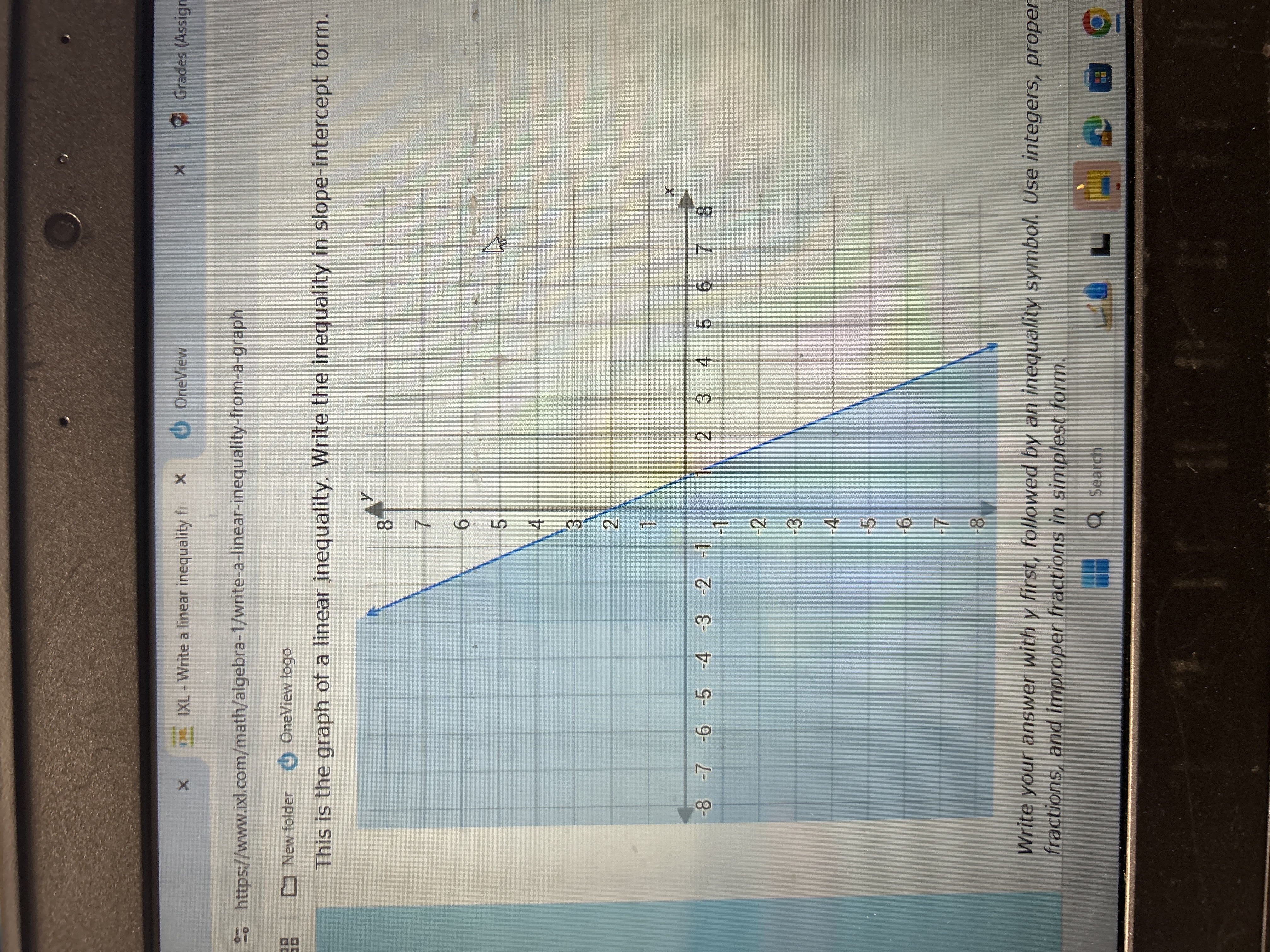
This is the graph of a linear inequality. Write the inequality in slope-intercept form.
Understand the Problem
The question is asking for the equation of a linear inequality that corresponds to a given graph. It specifies that the answer should be in slope-intercept form, which includes identifying the slope and y-intercept from the graph.
Answer
The inequality is given by: $$ y > -2x + 4 $$
Answer for screen readers
The inequality in slope-intercept form is:
$$ y > -2x + 4 $$
Steps to Solve
-
Identify the Slope and Y-Intercept From the graph, identify two points on the line to calculate the slope ($m$). For example, if the line passes through points (0, 4) and (2, 0), the slope $m$ can be calculated as:
$$ m = \frac{y_2 - y_1}{x_2 - x_1} = \frac{0 - 4}{2 - 0} = \frac{-4}{2} = -2 $$
The y-intercept ($b$) is the point where the line crosses the y-axis. In this case, it is 4. -
Write the Slope-Intercept Equation Using the slope and y-intercept, we can write the line in slope-intercept form ($y = mx + b$):
$$ y = -2x + 4 $$ -
Determine the Inequality Symbol Since the line is dashed (indicating that points on the line are not included), the inequality is not "less than or equal to" or "greater than or equal to." We need to assess the region shaded. If the shading is above the line, we write:
$$ y > -2x + 4 $$
If it is below the line, we would write:
$$ y < -2x + 4 $$
Based on the graph, if the shading is above, we keep the inequality as $y > -2x + 4$.
The inequality in slope-intercept form is:
$$ y > -2x + 4 $$
More Information
In a graph of a linear inequality, a dashed line means that points on the line are not included in the solution set. The shading direction indicates which area of the graph satisfies the inequality.
Tips
- Forgetting to check if the line is solid or dashed: A solid line means "greater than or equal to" (≥) or "less than or equal to" (≤), while a dashed line does not include those points.
- Misidentifying the shading area: Always confirm which side of the line is shaded to determine the correct inequality.
AI-generated content may contain errors. Please verify critical information