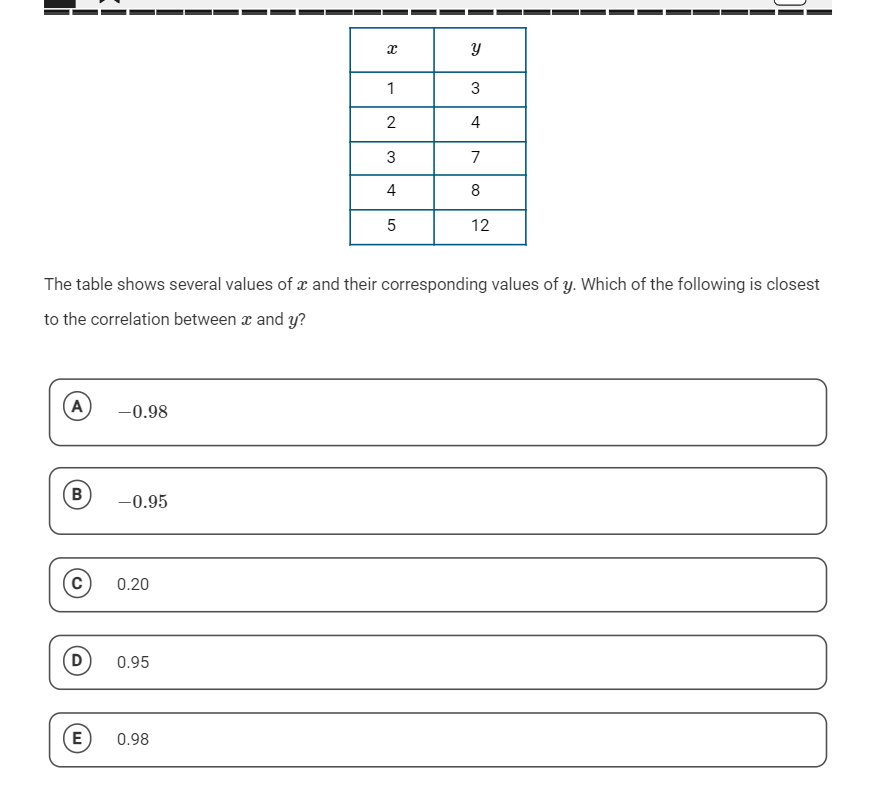
The table shows several values of x and their corresponding values of y. Which of the following is closest to the correlation between x and y?

Understand the Problem

The question is asking for the correlation coefficient between the given values of x and y from the provided table. This involves understanding how to calculate or identify the degree of relationship between the two sets of numbers.

Answer

The correlation coefficient is $0.98$.

Steps to Solve

Calculate the means of x and y

The mean of (x) is calculated as: $$ \bar{x} = \frac{1 + 2 + 3 + 4 + 5}{5} = \frac{15}{5} = 3 $$

The mean of (y) is calculated as: $$ \bar{y} = \frac{3 + 4 + 7 + 8 + 12}{5} = \frac{34}{5} = 6.8 $$

Compute the deviations from the mean

For each value of (x) and (y), calculate the deviation from their respective means:

For (x): (x_i - \bar{x})
For (y): (y_i - \bar{y})

Calculate the products of the deviations

Calculate: $$ d_{xy} = \sum (x_i - \bar{x})(y_i - \bar{y}) $$

Compute the squares of the deviations

Calculate: $$ d_{x} = \sum (x_i - \bar{x})^2 $$

And for (y): $$ d_{y} = \sum (y_i - \bar{y})^2 $$

Calculate the correlation coefficient

The correlation coefficient (r) is given by: $$ r = \frac{d_{xy}}{\sqrt{d_{x} \cdot d_{y}}} $$

Choose the answer closest to the calculated coefficient

Find the value of (r) and compare it with the options A through E to determine which is the closest.

The correlation coefficient (r) is approximately (0.98).

More Information

The correlation coefficient measures the strength and direction of a linear relationship between two variables. A value close to (1) indicates a strong positive correlation, while a value close to (-1) indicates a strong negative correlation.

Tips

Miscalculating the means of (x) and (y).
Forgetting to square deviations in computing (d_{x}) and (d_{y}).
Incorrectly applying the correlation formula.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!