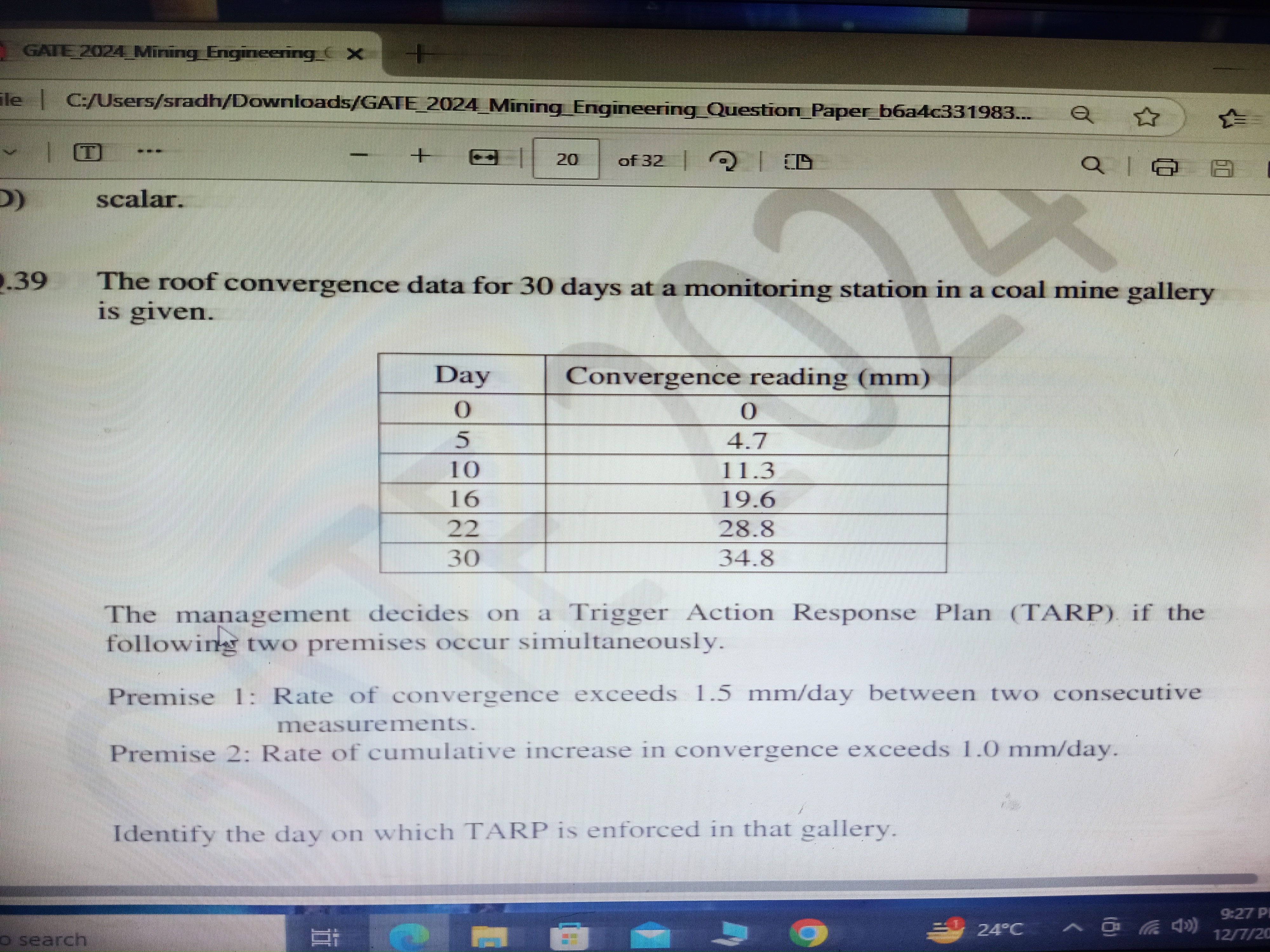
The roof convergence data for 30 days at a monitoring station in a coal mine gallery is given. The management decides on a Trigger Action Response Plan (TARP) if the following two... The roof convergence data for 30 days at a monitoring station in a coal mine gallery is given. The management decides on a Trigger Action Response Plan (TARP) if the following two premises occur simultaneously: Premise 1: Rate of convergence exceeds 1.5 mm/day between two consecutive measurements. Premise 2: Rate of cumulative increase in convergence exceeds 1.0 mm/day. Identify the day on which TARP is enforced in that gallery.
Understand the Problem
The question is asking to identify the day on which a Trigger Action Response Plan (TARP) is enforced based on the provided convergence data and the specified conditions regarding the rate of convergence.
Answer
TARP is enforced from Day 22.
Answer for screen readers
The TARP is enforced starting from Day 22.
Steps to Solve
- Calculate Daily Convergence Rates
We need to find the rate of convergence for each consecutive day:
-
From Day 0 to Day 5: $$ \text{Rate} = \frac{4.7 - 0}{5 - 0} = \frac{4.7}{5} = 0.94 , \text{mm/day} $$
-
From Day 5 to Day 10: $$ \text{Rate} = \frac{11.3 - 4.7}{10 - 5} = \frac{6.6}{5} = 1.32 , \text{mm/day} $$
-
From Day 10 to Day 16: $$ \text{Rate} = \frac{19.6 - 11.3}{16 - 10} = \frac{8.3}{6} \approx 1.38 , \text{mm/day} $$
-
From Day 16 to Day 22: $$ \text{Rate} = \frac{28.8 - 19.6}{22 - 16} = \frac{9.2}{6} \approx 1.53 , \text{mm/day} $$
-
From Day 22 to Day 30: $$ \text{Rate} = \frac{34.8 - 28.8}{30 - 22} = \frac{6.0}{8} = 0.75 , \text{mm/day} $$
- Identify Days Exceeding 1.5 mm/day
We compare the rates calculated to the 1.5 mm/day threshold:
- Day 0 to Day 5: 0.94 mm/day (not exceeding)
- Day 5 to Day 10: 1.32 mm/day (not exceeding)
- Day 10 to Day 16: 1.38 mm/day (not exceeding)
- Day 16 to Day 22: 1.53 mm/day (exceeds)
- Day 22 to Day 30: 0.75 mm/day (not exceeding)
Only the interval from Day 16 to Day 22 exceeds 1.5 mm/day.
- Calculate Cumulative Convergence Increase
Now, we need to calculate cumulative increases:
-
From Day 0 to Day 5: $$ 4.7 , \text{mm} $$
-
From Day 0 to Day 10: $$ 11.3 , \text{mm} $$
-
From Day 0 to Day 16: $$ 19.6 , \text{mm} $$
-
From Day 0 to Day 22: $$ 28.8 , \text{mm} $$
-
From Day 0 to Day 30: $$ 34.8 , \text{mm} $$
Now calculate the daily cumulative increases for the days of interest:
- From Day 16 to Day 22: $$ \text{Cumulative Rate} = \frac{28.8 - 19.6}{22 - 16} = \frac{9.2}{6} \approx 1.53 , \text{mm/day} $$
- Determine When Both Premises are Met
- Premise 1 is satisfied on Day 16 to Day 22 (1.53 mm/day).
- Premise 2 is satisfied on Day 16 to Day 22 (1.53 mm/day).
Thus, TARP will be enforced after Day 16.
The TARP is enforced starting from Day 22.
More Information
The TARP is a safety protocol in mining that is activated based on certain risk criteria. It helps ensure the safety of the personnel and the integrity of mining operations.
Tips
- Not calculating the differences accurately between the convergence values.
- Failing to check both premises simultaneously, leading to premature conclusions.
- Misinterpreting daily vs. cumulative rates.
AI-generated content may contain errors. Please verify critical information