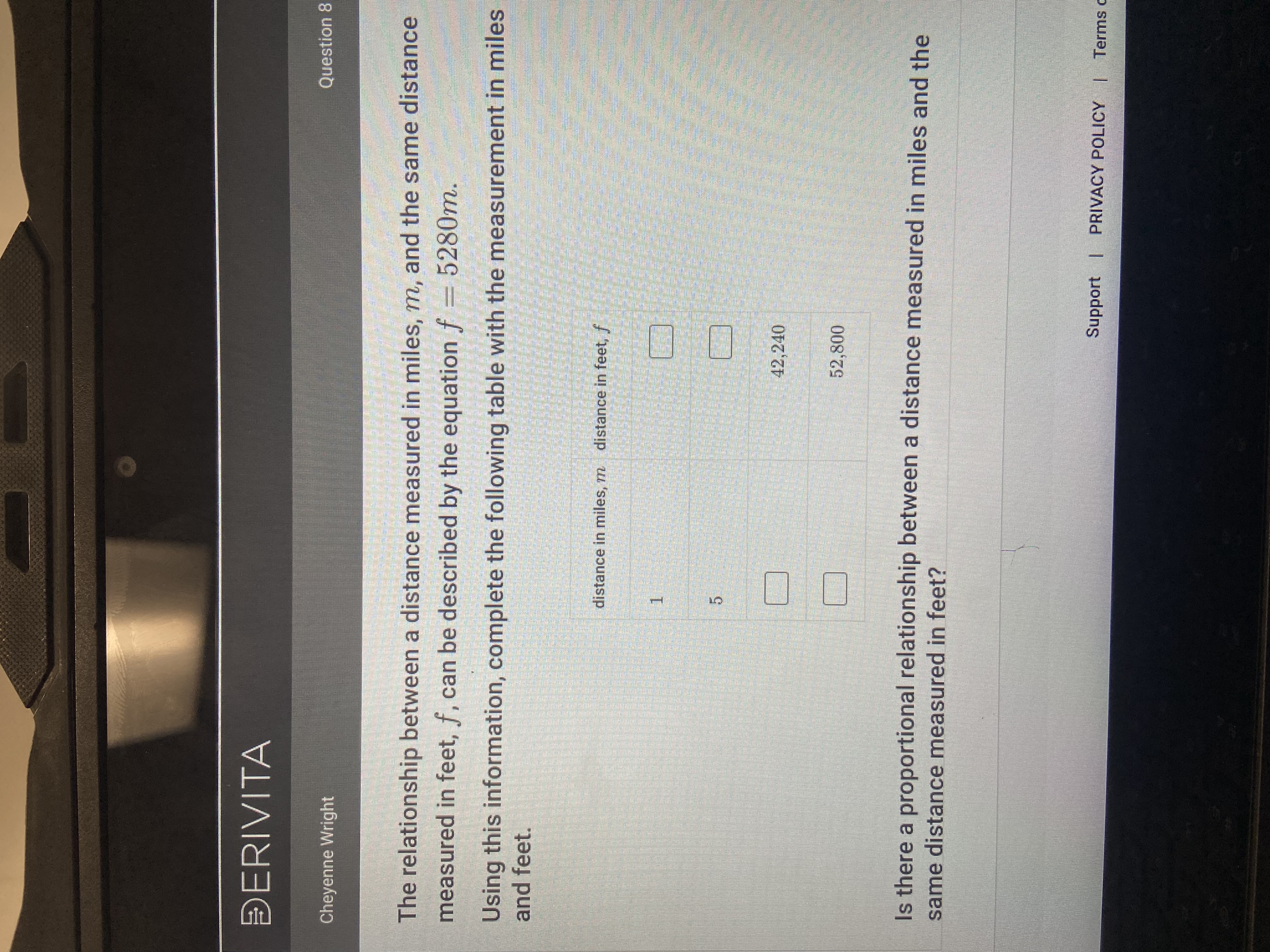
The relationship between a distance measured in miles, m, and the same distance measured in feet, f, can be described by the equation f = 5280m. Using this information, complete th... The relationship between a distance measured in miles, m, and the same distance measured in feet, f, can be described by the equation f = 5280m. Using this information, complete the following table with the measurement in miles and feet. Is there a proportional relationship between a distance measured in miles and the same distance measured in feet?
Understand the Problem
The question is asking to complete a table that relates distances in miles to feet based on a given equation, and to determine if there is a proportional relationship between these two measurements.
Answer
Yes, there is a proportional relationship between distance in miles and the same distance measured in feet.
Answer for screen readers
The completed table is:
| distance in miles, ( m ) | distance in feet, ( f ) |
|---|---|
| 1 | 5280 |
| 5 | 26400 |
| 8 | 42240 |
| 10 | 52800 |
Yes, there is a proportional relationship.
Steps to Solve
-
Understanding the Equation The relationship between miles and feet is given by the equation ( f = 5280m ). Here, ( f ) represents the distance in feet, and ( m ) represents the distance in miles.
-
Calculate Distance in Feet for 1 Mile Using the equation for ( m = 1 ): [ f = 5280 \times 1 = 5280 \text{ feet} ] This fills in the first row of the table.
-
Calculate Distance in Feet for 5 Miles Now for ( m = 5 ): [ f = 5280 \times 5 = 26400 \text{ feet} ] This fills in the second row of the table.
-
Check Given Values for 42,240 and 52,800 Feet To see if ( 42,240 ) and ( 52,800 ) are valid:
- For ( 42,240 ): [ \frac{42240}{5280} = 8 \implies m = 8 \text{ miles} ]
- For ( 52,800 ): [ \frac{52800}{5280} = 10 \implies m = 10 \text{ miles} ] This indicates both values correspond to specific distances in miles.
-
Determine Proportional Relationship To check if the relationship is proportional, see if the ratio ( \frac{f}{m} ) is constant:
- For ( m = 1 ), ( \frac{5280}{1} = 5280 )
- For ( m = 5 ), ( \frac{26400}{5} = 5280 )
- For ( m = 8 ), ( \frac{42240}{8} = 5280 )
- For ( m = 10 ), ( \frac{52800}{10} = 5280 )
Since the ratio is constant, the relationship is proportional.
The completed table is:
| distance in miles, ( m ) | distance in feet, ( f ) |
|---|---|
| 1 | 5280 |
| 5 | 26400 |
| 8 | 42240 |
| 10 | 52800 |
Yes, there is a proportional relationship.
More Information
The equation ( f = 5280m ) shows a direct relationship between miles and feet. This means for every mile, there are 5280 feet, which is a standard conversion.
Tips
- Miscalculating the multiplication when finding ( f ) for each mile.
- Forgetting to check if the ratios ( \frac{f}{m} ) are consistent to determine proportionality.
AI-generated content may contain errors. Please verify critical information