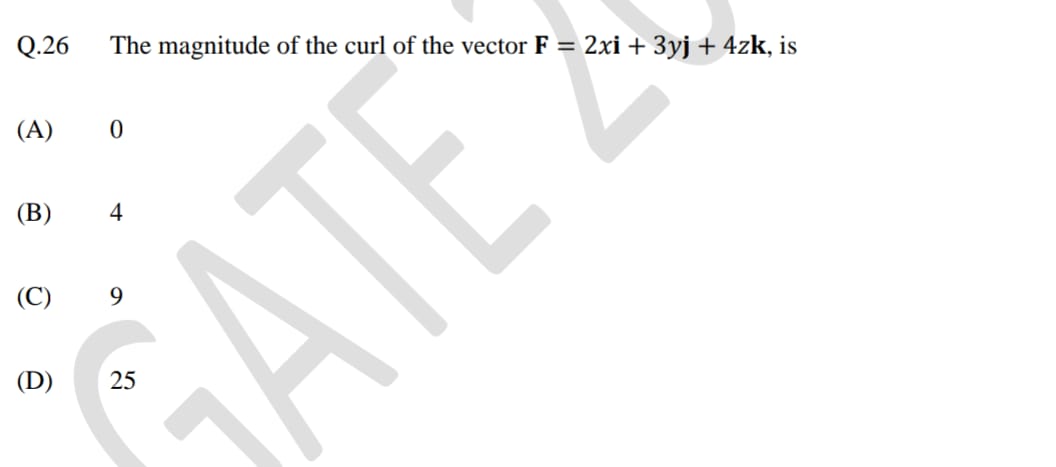
The magnitude of the curl of the vector F = 2xi + 3yj + 4zk, is
Understand the Problem
The question is asking for the magnitude of the curl of a given vector field F. Specifically, it provides the vector as F = 2xi + 3yj + 4zk and requests the calculation of its curl's magnitude.
Answer
The magnitude of the curl is $0$.
Answer for screen readers
The magnitude of the curl of the vector ( \mathbf{F} = 2x \mathbf{i} + 3y \mathbf{j} + 4z \mathbf{k} ) is ( 0 ).
Steps to Solve
- Identify the components of the vector field The vector field is given as ( \mathbf{F} = 2x \mathbf{i} + 3y \mathbf{j} + 4z \mathbf{k} ). Thus, the components are:
- ( F_1 = 2x )
- ( F_2 = 3y )
- ( F_3 = 4z )
-
Set up the curl formula The curl of a vector field ( \mathbf{F} ) in three dimensions is calculated using the formula: $$ \nabla \times \mathbf{F} = \begin{vmatrix} \mathbf{i} & \mathbf{j} & \mathbf{k} \ \frac{\partial F_1}{\partial x} & \frac{\partial F_2}{\partial x} & \frac{\partial F_3}{\partial x} \ \frac{\partial F_1}{\partial y} & \frac{\partial F_2}{\partial y} & \frac{\partial F_3}{\partial y} \ \frac{\partial F_1}{\partial z} & \frac{\partial F_2}{\partial z} & \frac{\partial F_3}{\partial z} \end{vmatrix} $$
-
Compute partial derivatives Calculate the necessary partial derivatives:
- ( \frac{\partial F_1}{\partial y} = 0 )
- ( \frac{\partial F_1}{\partial z} = 0 )
- ( \frac{\partial F_2}{\partial x} = 0 )
- ( \frac{\partial F_2}{\partial z} = 0 )
- ( \frac{\partial F_3}{\partial x} = 0 )
- ( \frac{\partial F_3}{\partial y} = 0 )
-
Evaluate the determinant Substituting the values into the determinant gives: $$ \nabla \times \mathbf{F} = \begin{vmatrix} \mathbf{i} & \mathbf{j} & \mathbf{k} \ 0 & 0 & 0 \ 0 & 0 & 0 \end{vmatrix} = \mathbf{0} $$ Thus, the curl of the vector field is zero.
-
Calculate the magnitude of the curl The magnitude of a zero vector is: $$ |\nabla \times \mathbf{F}| = 0 $$
The magnitude of the curl of the vector ( \mathbf{F} = 2x \mathbf{i} + 3y \mathbf{j} + 4z \mathbf{k} ) is ( 0 ).
More Information
The curl measures the rotation of a vector field. In this case, since the vector field is linear (no terms depending on both ( y ) and ( z ), for example), the field does not rotate, resulting in a curl of zero.
Tips
- Forgetting to compute the partial derivatives correctly.
- Misunderstanding the curl's significance — it's specifically zero for linear vector fields with constant coefficients.
AI-generated content may contain errors. Please verify critical information