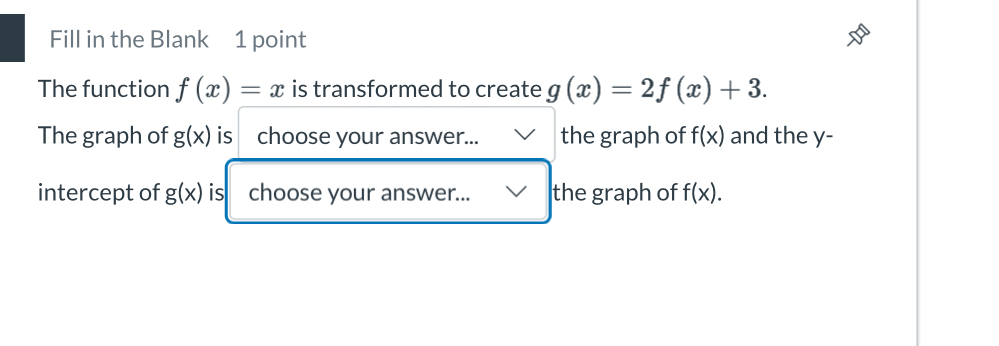
The function f(x) = x is transformed to create g(x) = 2f(x) + 3. The graph of g(x) is ______________ the graph of f(x) and the y-intercept of g(x) is ______________ the graph of f(... The function f(x) = x is transformed to create g(x) = 2f(x) + 3. The graph of g(x) is ______________ the graph of f(x) and the y-intercept of g(x) is ______________ the graph of f(x).
Understand the Problem
The question is asking about the transformation of a function based on a given mathematical expression, specifically regarding how the graph of the function changes compared to the original function. It involves understanding transformations such as vertical and horizontal shifts and scaling.
Answer
The graph of $g(x)$ is **not** the graph of $f(x)$ and the y-intercept of $g(x)$ is **not** the graph of $f(x)$.
Answer for screen readers
The graph of $g(x)$ is not the graph of $f(x)$ and the y-intercept of $g(x)$ is not the graph of $f(x)$.
Steps to Solve
-
Identify the function
The original function is given as $f(x) = x$. The transformed function is $g(x) = 2f(x) + 3$. -
Substitute the original function into g(x)
Substituting $f(x)$ into $g(x)$ gives:
$$ g(x) = 2(x) + 3 = 2x + 3 $$ -
Analyze the transformations
The transformation involves:
- Vertical scaling by a factor of 2 (which stretches the graph vertically).
- A vertical shift upward by 3 units.
-
Determine the effect on the graph
Since $g(x)$ is vertically stretched and shifted, it will not be the same as the graph of $f(x)$. Thus, the graph of $g(x)$ is not the graph of $f(x)$. -
Find the y-intercept of g(x)
To find the y-intercept, set $x = 0$:
$$ g(0) = 2(0) + 3 = 3 $$
Therefore, the y-intercept of $g(x)$ is at (0, 3). -
Conclusion about the y-intercept
Since the y-intercept of $g(x)$ is (0, 3) and does not fall on the y-intercept of $f(x)$ (which is (0, 0)), the y-intercept of $g(x)$ is also not on the graph of $f(x)$.
The graph of $g(x)$ is not the graph of $f(x)$ and the y-intercept of $g(x)$ is not the graph of $f(x)$.
More Information
The transformation of the function $f(x) = x$ to $g(x) = 2f(x) + 3$ involves a vertical stretch and a vertical shift, significantly altering the graph's position and shape.
Tips
Common mistakes include:
- Confusing vertical shifts with horizontal shifts.
- Ignoring the effect of scaling when determining the new graph's appearance.
AI-generated content may contain errors. Please verify critical information