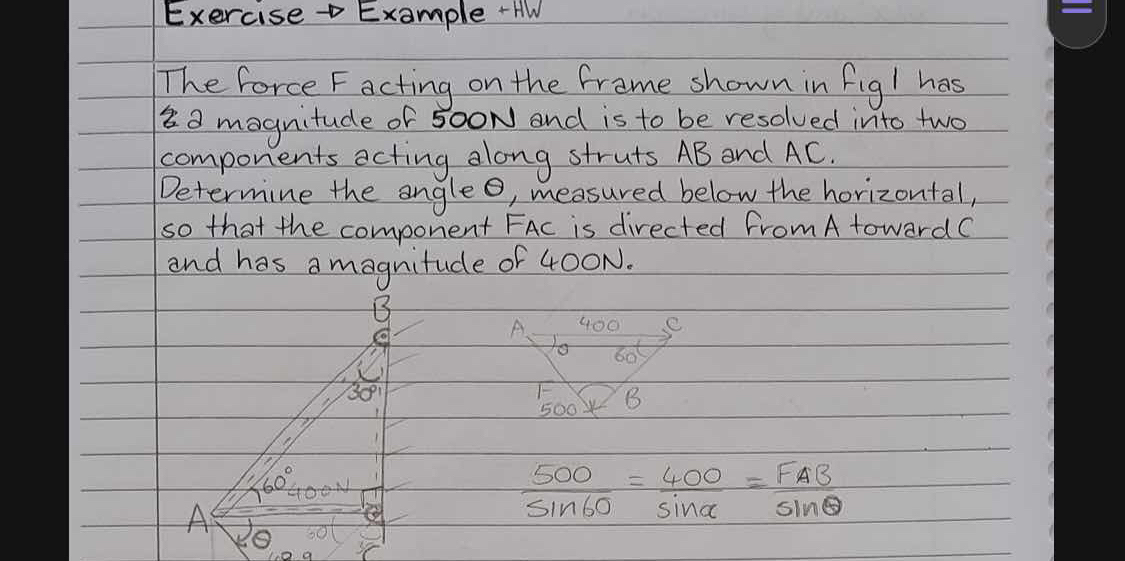
The force F acting on the frame shown in fig 1 has a magnitude of 500N and is to be resolved into two components acting along struts AB and AC. Determine the angle θ, measured belo... The force F acting on the frame shown in fig 1 has a magnitude of 500N and is to be resolved into two components acting along struts AB and AC. Determine the angle θ, measured below the horizontal, so that the component FAC is directed from A toward C and has a magnitude of 400N. Read more

Steps to Solve

1. Identify the known values and equations
   Given the force magnitude ( F = 500 , \text{N} ) and the component ( F_{AC} = 400 , \text{N} ), we can use the force resolution equations. The equations based on the triangle formed by the forces are: $$ F = F_{AC} + F_{AB} $$

2. Apply the sine rule for force components
   Using the sine rule for resolving the forces along struts AB and AC: $$ \frac{F}{\sin(50^\circ)} = \frac{F_{AC}}{\sin(\theta)} = \frac{F_{AB}}{\sin(60^\circ)} $$

3. Substitute known values into the equation
   Substituting for ( F_{AC} = 400 , \text{N} ): $$ \frac{500}{\sin(50^\circ)} = \frac{400}{\sin(\theta)} $$

4. Cross-multiply and solve for ( \sin(\theta) )
   Cross-multiplying gives us: $$ 500 \cdot \sin(\theta) = 400 \cdot \sin(50^\circ) $$ Rearranging this gives: $$ \sin(\theta) = \frac{400 \cdot \sin(50^\circ)}{500} $$

5. Calculate ( \sin(50^\circ) )
   Using a calculator or reference: $$ \sin(50^\circ) \approx 0.766 $$ Then compute: $$ \sin(\theta) = \frac{400 \cdot 0.766}{500} = 0.613 $$

6. Find ( \theta )
   Taking the inverse sine to find ( \theta ): $$ \theta = \sin^{-1}(0.613) \approx 37.67^\circ $$