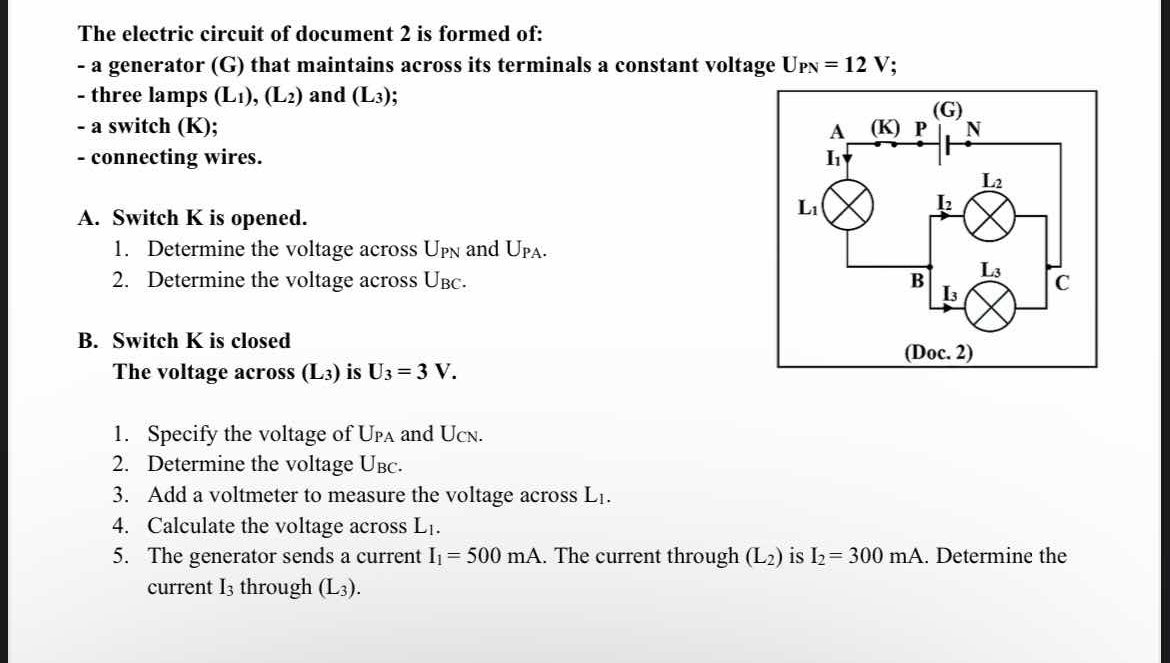
The electric circuit of document 2 is formed of a generator, three lamps, a switch, and connecting wires. When switch K is opened, determine the voltage across UPN and UPA, and the... The electric circuit of document 2 is formed of a generator, three lamps, a switch, and connecting wires. When switch K is opened, determine the voltage across UPN and UPA, and the voltage across UBC. When switch K is closed and the voltage across L3 is 3V, specify the voltage of UPA and UCN, determine the voltage UBC, add a voltmeter to measure the voltage across L1, calculate the voltage across L1, and determine the current through L3 given that I1 is 500 mA and I2 is 300 mA. Read more

Steps to Solve

1. Analyzing the Circuit with Switch K Opened

When the switch ( K ) is opened, the circuit is broken. The total voltage across the generator ( U_{PN} = 12 , \text{V} ) will be dropped across the lamps ( L_1 ) and ( L_2 ).

2. Determining Voltage Across ( U_{PA} ) and ( U_{PN} )

Since the total voltage from the generator is ( 12 , \text{V} ), and considering an ideal situation where there's no voltage drop across the wires, we have:

$$ U_{PA} + U_{CN} = U_{PN} = 12 , \text{V} $$

If both lamps ( L_1 ) and ( L_2 ) have equal resistance, then they will share the voltage equally.

3. Using Voltage Divider Rule

Assuming equal resistance for ( L_1 ) and ( L_2 ):

$$ U_{PA} = U_{CN} = \frac{U_{PN}}{2} = \frac{12 , \text{V}}{2} = 6 , \text{V} $$

4. Determining Voltage Across ( U_{BC} )

To find ( U_{BC} ), observe that it is the voltage across the two lamps in parallel, which must be equal:

$$ U_{BC} = U_{PA} = 6 , \text{V} $$

5. Switch K is Closed

Now we analyze the circuit when switch ( K ) is closed. The voltage across lamp ( L_3 ) is given as ( U_3 = 3 , \text{V} ).

6. Determining Voltages Across ( U_{PA} ) and ( U_{CN} )

Using Kirchhoff's Voltage Law, the total voltage must equal the sum of the voltages across the components. Since ( U_{3} = 3 , \text{V} ):

$$ U_{PA} + U_{CN} + U_3 = U_{PN} $$

Therefore:

$$ U_{PA} + U_{CN} + 3 , \text{V} = 12 , \text{V} $$

From this equation:

$$ U_{PA} + U_{CN} = 12 , \text{V} - 3 , \text{V} = 9 , \text{V} $$

7. Dividing Voltage Between ( U_{PA} ) and ( U_{CN} )

Assuming ( U_{PA} = U_{CN} ):

$$ U_{PA} = U_{CN} = \frac{9 , \text{V}}{2} = 4.5 , \text{V} $$

8. Determining Voltage Across ( U_{BC} )

The voltage ( U_{BC} ) across the two parallel lamps can be calculated similarly or verified through Kirchhoff’s Law:

$$ U_{BC} = U_{PN} - U_{3} = 12 , \text{V} - 3 , \text{V} = 9 , \text{V} $$

9. Calculating Voltage Across ( L_1 )

Using voltage division:

If ( I_1 = 500 , \text{mA} ) and ( I_2 = 300 , \text{mA} ) are the currents through ( L_1 ) and ( L_2 ), then

$$ I_3 = I_1 - I_2 = 500 , \text{mA} - 300 , \text{mA} = 200 , \text{mA} $$

Assuming equal resistance, we can calculate voltage across ( L_1 ) using Ohm's Law.