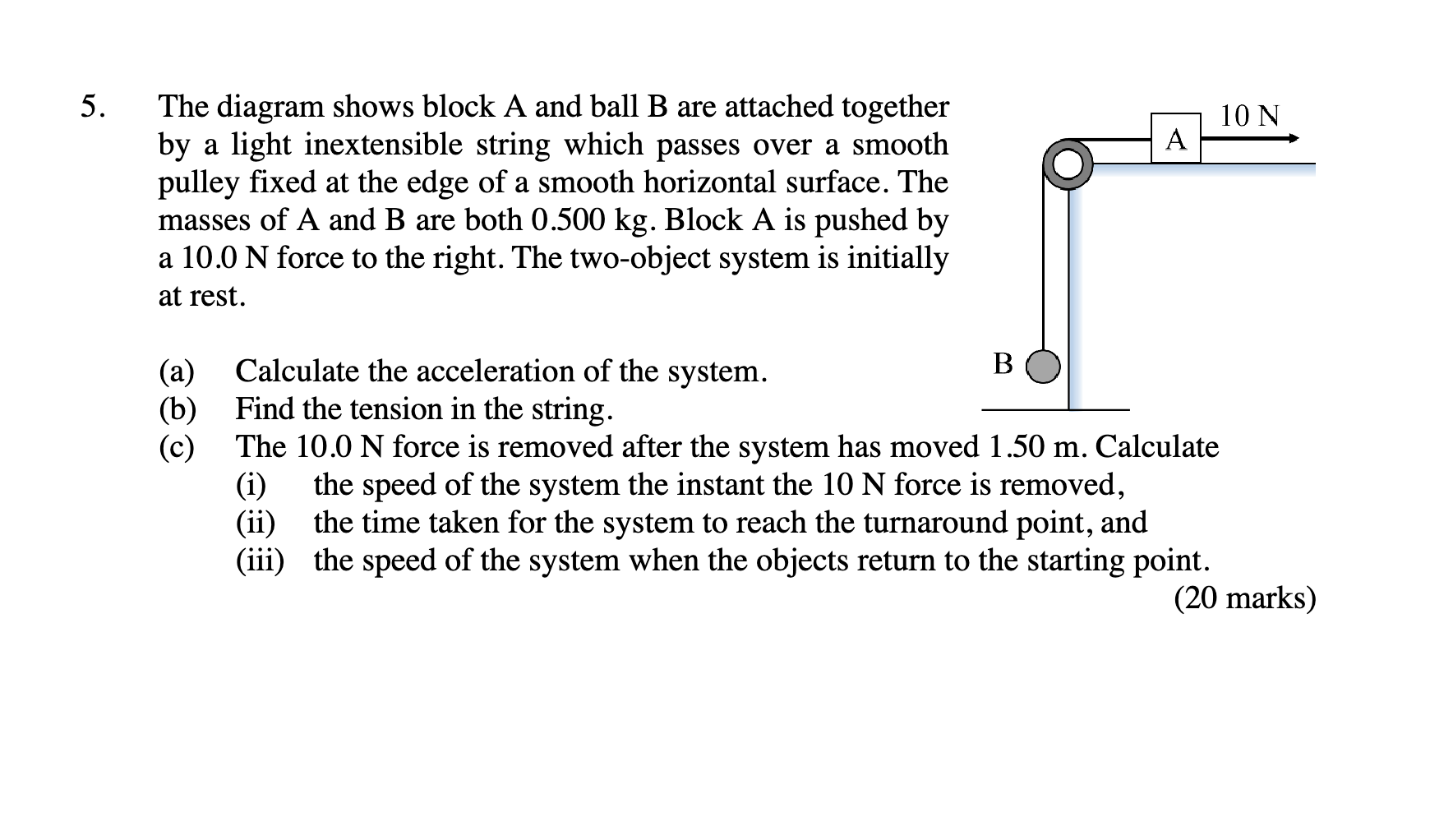
The diagram shows block A and ball B are attached together by a light inextensible string which passes over a smooth pulley fixed at the edge of a smooth horizontal surface. The ma... The diagram shows block A and ball B are attached together by a light inextensible string which passes over a smooth pulley fixed at the edge of a smooth horizontal surface. The masses of A and B are both 0.500 kg. Block A is pushed by a 10.0 N force to the right. The two-object system is initially at rest. (a) Calculate the acceleration of the system. (b) Find the tension in the string. (c) The 10.0 N force is removed after the system has moved 1.50. Calculate (i) the speed of the system the instant the 10 N force is removed, (ii) the time taken for the system to reach the turnaround point, and (iii) the speed of the system when the objects return to the starting point. Read more

Steps to Solve

1. Calculate the acceleration of the system

The net force acting on the system can be calculated. Here, the applied force is 10 N, and we need to consider the weight of block B as a force acting downwards. Block B's weight is given by $mg = 0.500 , \text{kg} \times 9.81 , \text{m/s}^2 = 4.905 , \text{N}$.

The total mass of the system (A and B) is:

$$ m_{total} = m_A + m_B = 0.500 , \text{kg} + 0.500 , \text{kg} = 1.000 , \text{kg} $$

The net force $F_{net}$ acting on the system is:

$$ F_{net} = F - F_B = 10.0 , \text{N} - 4.905 , \text{N} = 5.095 , \text{N} $$

Now, use Newton's second law ($F = ma$) to find the acceleration $a$:

$$ a = \frac{F_{net}}{m_{total}} = \frac{5.095 , \text{N}}{1.000 , \text{kg}} = 5.095 , \text{m/s}^2 $$

2. Find the tension in the string

The tension $T$ in the string can be found by analyzing the forces acting on block B. The net force acting on block B is its weight minus the tension. Using:

$$ F_B = m_B \cdot g = 0.500 , \text{kg} \times 9.81 , \text{m/s}^2 = 4.905 , \text{N} $$

The net force on block B is:

$$ F_{net , B} = T - F_B $$

Using Newton’s second law for block B, we find:

$$ T = F_B + m_B \cdot a $$ $$ T = 4.905 , \text{N} + 0.500 , \text{kg} \times 5.095 , \text{m/s}^2 $$ $$ T = 4.905 , \text{N} + 2.5475 , \text{N} = 7.4525 , \text{N} $$

3. Calculate the speed of the system when the force is removed

When the 10 N force is removed, the only forces acting on the system are the weight of block B and the tension in the string. Block A will have moved 1.50 m before the force was removed. We can calculate the speed $v$ using the equation of motion:

$$ v^2 = u^2 + 2as $$

Where $u = 0$ (initial speed), $a = 5.095 , \text{m/s}^2$, and $s = 1.50 , \text{m}$.

Calculating $v$ gives:

$$ v^2 = 0 + 2 \cdot 5.095 , \text{m/s}^2 \cdot 1.50 , \text{m} $$ $$ v^2 = 15.1425 $$ $$ v = \sqrt{15.1425} \approx 3.887 , \text{m/s} $$

4. Calculate the time taken to reach the turnaround point

Using the equation of motion again, we solve for the time $t$:

$$ v = u + at $$

Rearranging gives:

$$ t = \frac{v - u}{a} = \frac{3.887 , \text{m/s} - 0}{5.095 , \text{m/s}^2} \approx 0.764 , \text{s} $$

5. Calculate the speed when the objects return to the starting point

When the system returns to the starting point, it will have undergone deceleration due to the tension in the string (now acting against the motion). The speed can be calculated using energy conservation or kinematic equations but will depend on the distance traveled during deceleration. Thus, we can say that it will return to zero speed when they return.