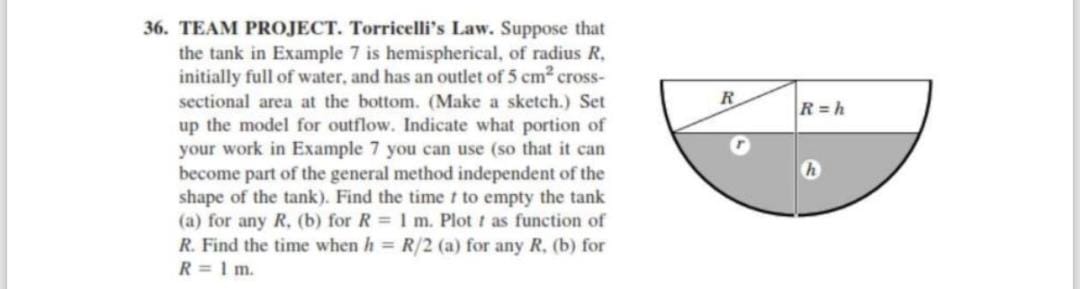
Suppose that the tank is hemispherical, of radius R, initially full of water, and has an outlet of 5 cm² cross-sectional area at the bottom. Set up the model for outflow. Find the... Suppose that the tank is hemispherical, of radius R, initially full of water, and has an outlet of 5 cm² cross-sectional area at the bottom. Set up the model for outflow. Find the time t to empty the tank (a) for any R, (b) for R = 1 m. Plot t as a function of R. Find the time when h = R/2 (a) for any R, (b) for R = 1 m. Read more

Steps to Solve

1. Understanding Torricelli's Law

   Torricelli's Law states that the speed of fluid flowing out of an orifice under the force of gravity is given by: $$ v = \sqrt{2gh} $$ where ( g ) is the acceleration due to gravity and ( h ) is the height of the fluid above the outlet.

2. Set Up the Equation for Outflow

   The flow rate through the outlet can be written as: $$ Q = A v $$ where ( A ) is the cross-sectional area of the outlet. Given ( A = 5 , \text{cm}^2 = 0.0005 , \text{m}^2 ), we have: $$ Q = 0.0005 \sqrt{2gh} $$

3. Relate Flow Rate to Change in Volume

   The volume ( V ) of water in a hemispherical tank can be represented as: $$ V = \frac{1}{2} \pi h^2 (3R - h) $$ The change in volume is related to the change in height by: $$ \frac{dV}{dt} = -Q $$

4. Set Up Volume Differential Equation

   By substituting ( Q ) into the volume equation, we have: $$ -0.0005 \sqrt{2gh} = \frac{dV}{dt} $$ and since ( dV = \frac{dV}{dh} dh ): $$ -0.0005 \sqrt{2gh} = \frac{dV}{dh} \frac{dh}{dt} $$

5. Determine ( \frac{dV}{dh} ) and Rearrange

   Compute ( \frac{dV}{dh} ): $$ \frac{dV}{dh} = \frac{d}{dh} \left( \frac{1}{2} \pi h^2 (3R - h) \right) $$ This gives: $$ \frac{dV}{dh} = \pi h(3R - h) - \frac{1}{2} \pi h^2 $$

6. Solve the Differential Equation

   Set up the differential equation with variables separated: $$ dt = -\frac{0.0005 \sqrt{2gh}}{\frac{dV}{dh}} dh $$

7. Integrate to Find Time t

   Integrate to solve for ( t ): $$ t = \int_{h=H}^{h=0} -\frac{0.0005 \sqrt{2gh}}{\frac{dV}{dh}} dh $$

8. Calculate Specific Cases

   For ( R = 1,m ), input the specific values into the integrated equation to find the time ( t ).

9. Plot ( t ) as a Function of ( R )

   Use computational tools or graphing to visualize ( t ) against various values of ( R ).

10. Find Time When ( h = \frac{R}{2} )

    Substitute ( h = \frac{R}{2} ) into the equation derived to solve for specific time values.