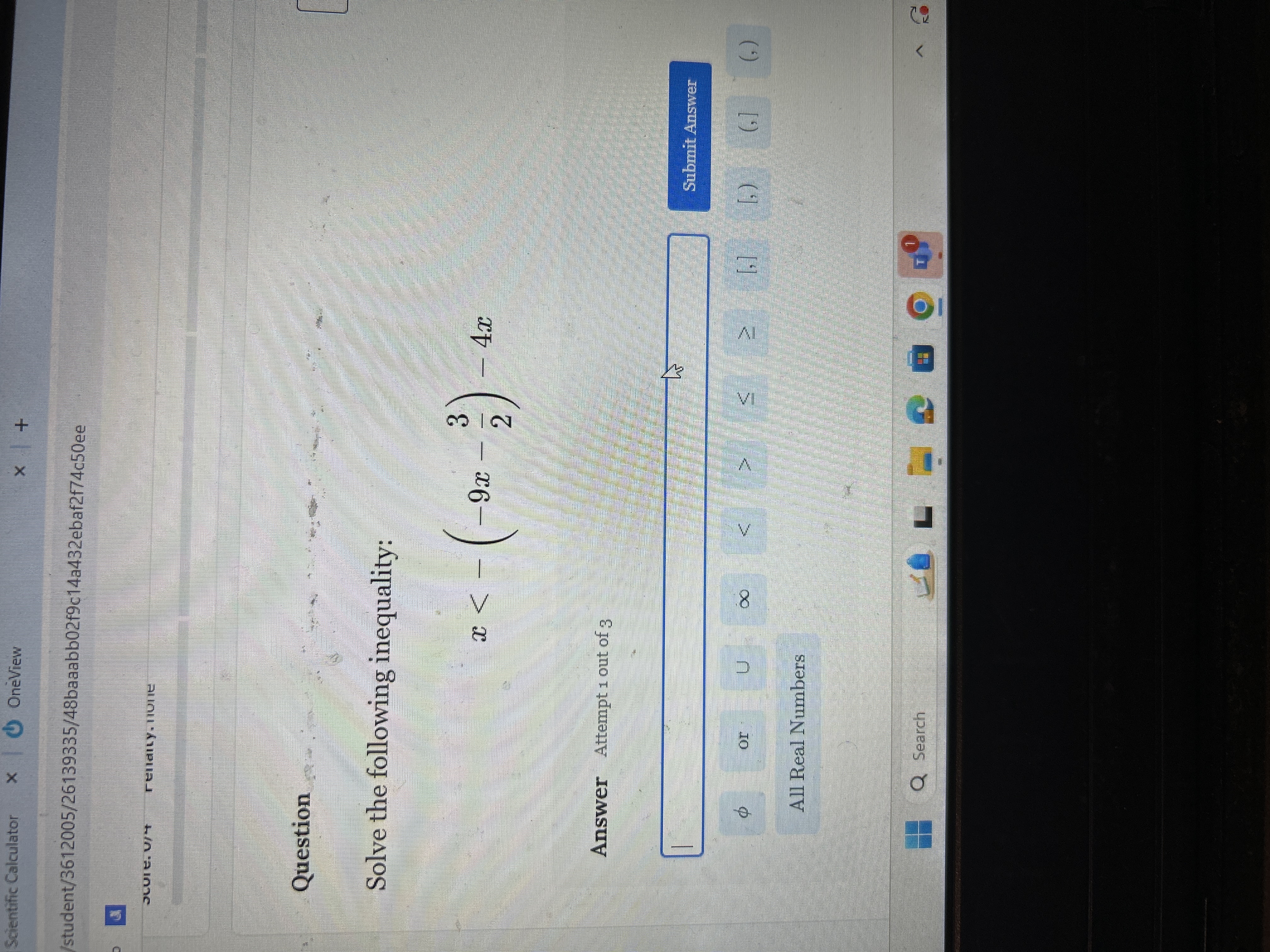
Solve the following inequality: x < -(-9x - 3/2) - 4x.
Understand the Problem
The question is asking us to solve the inequality: x < -(-9x - 3/2) - 4x. We will simplify the inequality and find the values of x that satisfy it.
Answer
The solution to the inequality is \(x > -\frac{3}{8}\).
Answer for screen readers
The solution to the inequality is:
$$ x > -\frac{3}{8} $$
Steps to Solve
- Start with the original inequality
We begin with the inequality:
$$ x < -(-9x - \frac{3}{2}) - 4x $$
- Simplify the right side
First, simplify the right side. The double negative turns into a positive:
$$ x < 9x + \frac{3}{2} - 4x $$
Now, combine like terms:
$$ x < (9x - 4x) + \frac{3}{2} $$
Which simplifies to:
$$ x < 5x + \frac{3}{2} $$
- Rearranging the inequality
Next, move all terms involving (x) to one side. Subtract (5x) from both sides:
$$ x - 5x < \frac{3}{2} $$
This simplifies to:
$$ -4x < \frac{3}{2} $$
- Isolate (x)
Now, divide both sides by (-4). Remember, when dividing by a negative number, the inequality sign flips:
$$ x > -\frac{3}{8} $$
The solution to the inequality is:
$$ x > -\frac{3}{8} $$
More Information
This means that (x) can take any value greater than (-\frac{3}{8}). In interval notation, this is expressed as ((- \frac{3}{8}, \infty)).
Tips
- Not flipping the inequality sign when dividing by a negative number is a common mistake. Always remember that this is necessary when isolating the variable in an inequality.
AI-generated content may contain errors. Please verify critical information