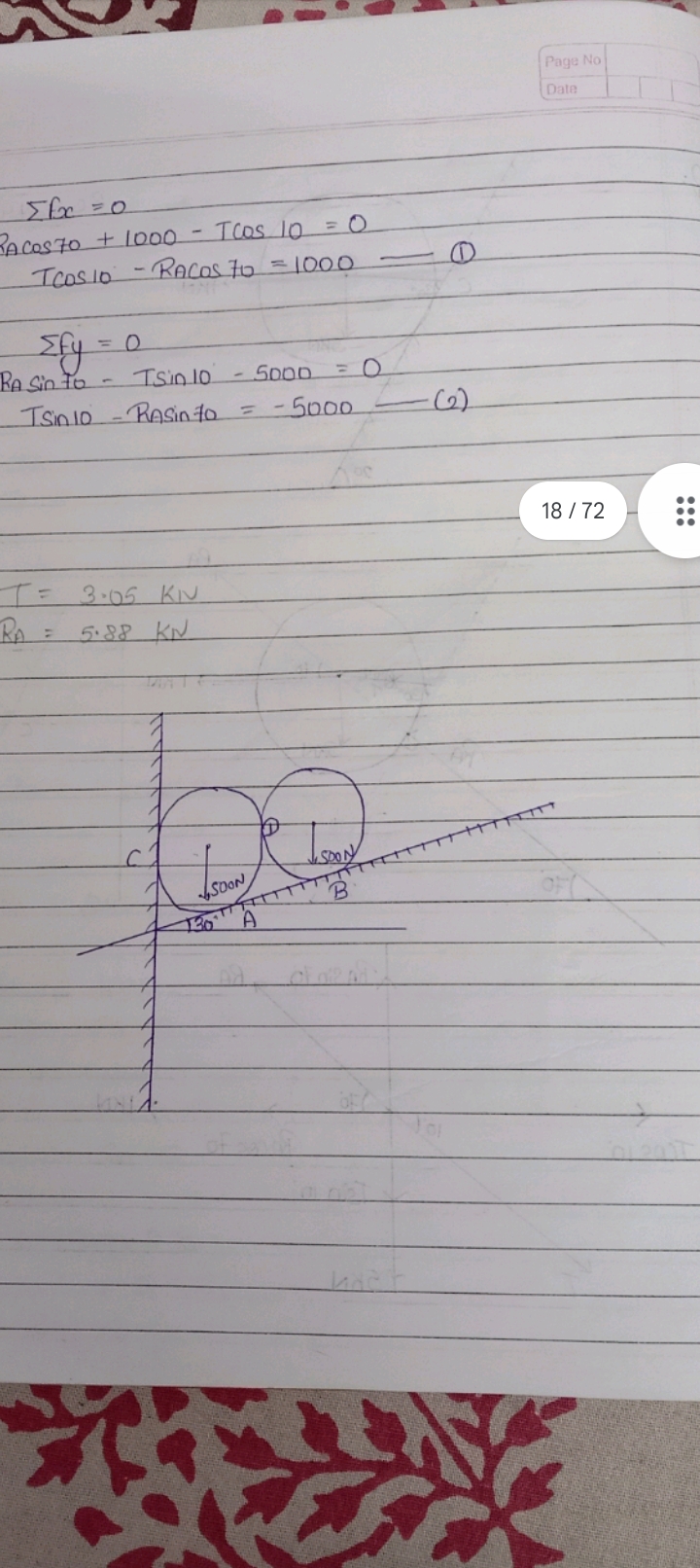
Solve the equations given in the image for Ra and T based on the equilibrium conditions.
Understand the Problem
The question involves solving equilibrium equations related to forces acting on a system of pulleys or similar setup. It provides equations that must be resolved to find unknown forces.
Answer
$$ T = 3.05 \text{ kN}, \quad R_a = 5.88 \text{ kN} $$
Answer for screen readers
The calculated values are: $$ T = 3.05 \text{ kN} $$ $$ R_a = 5.88 \text{ kN} $$
Steps to Solve
-
Start with the equations We have two equations derived from equilibrium conditions in the x and y directions.
- From the x-direction: $$ R_a \cos 70 + 1000 - T \cos 10 = 0 $$
- From the y-direction: $$ R_a \sin 10 - T \sin 10 - 5000 = 0 $$
-
Rearrange the equations Express ( T ) from the first equation: $$ T \cos 10 = R_a \cos 70 + 1000 $$ Then, we can solve for ( T ): $$ T = \frac{ R_a \cos 70 + 1000}{\cos 10} $$
-
Substitute ( T ) into the second equation Now we place the expression for ( T ) into the second equation: $$ R_a \sin 10 - \left(\frac{ R_a \cos 70 + 1000}{\cos 10}\right) \sin 10 - 5000 = 0 $$ This will help us isolate ( R_a ).
-
Simplify and solve for ( R_a ) Multiply through by ( \cos 10 ) to eliminate the fraction: $$ R_a \sin 10 \cos 10 - (R_a \cos 70 + 1000) \sin 10 - 5000 \cos 10 = 0 $$ Then combine terms to isolate ( R_a ): $$ (R_a \sin 10 \cos 10 - R_a \sin 10 \cos 70) = 5000 \cos 10 + 1000 \sin 10 $$
-
Factor out ( R_a ) $$ R_a (\sin 10 \cos 10 - \sin 10 \cos 70) = 5000 \cos 10 + 1000 \sin 10 $$ Now solve for ( R_a ): $$ R_a = \frac{5000 \cos 10 + 1000 \sin 10}{\sin 10 \cos 10 - \sin 10 \cos 70} $$
-
Calculate values for ( R_a ) and ( T ) Substitute known values of ( \sin ) and ( \cos ) into the equation to find numerical values for ( R_a ) and then substitute ( R_a ) back to find ( T ).
The calculated values are: $$ T = 3.05 \text{ kN} $$ $$ R_a = 5.88 \text{ kN} $$
More Information
The values for tension ( T ) and reaction force ( R_a ) reflect the balance of forces in equilibrium. The angles given (70° and 10°) played a crucial role in the calculation, affecting how the forces resolve in both horizontal and vertical directions.
Tips
- Forgetting to account for both directions: It's important to write equilibrium equations for both x and y directions.
- Neglecting to reduce fractions: When rearranging equations, ensure fractions are simplified to prevent errors in calculations.
- Miscalculating trigonometric values: Always double-check sine and cosine values, especially for less common angles.
AI-generated content may contain errors. Please verify critical information