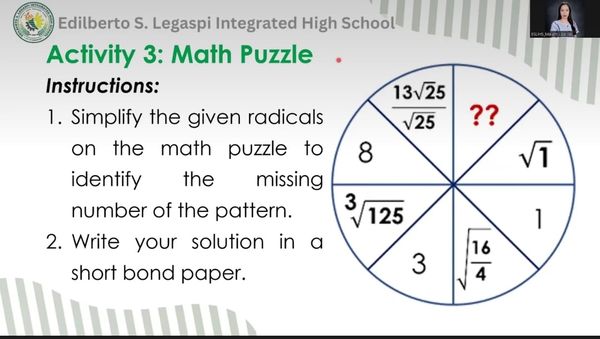
Simplify the given radicals on the math puzzle to identify the missing number of the pattern.

Steps to Solve

1. Simplify the first radical: $\sqrt{25}$

The square root of 25 can be simplified directly: $$ \sqrt{25} = 5 $$

2. Simplify the second radical: $\sqrt{16}$

The square root of 16 is: $$ \sqrt{16} = 4 $$

3. Simplify the third radical: $\sqrt{125}$

The square root of 125 can be rewritten as: $$ \sqrt{125} = \sqrt{25 \cdot 5} = \sqrt{25} \cdot \sqrt{5} = 5\sqrt{5} $$

4. Simplify the fourth radical: $\sqrt{3}$

This radical is already in its simplest form: $$ \sqrt{3} = \sqrt{3} $$

5. Calculate the given values and find the pattern

Now we look at the results:

• $\sqrt{25} = 5$
• $\sqrt{16} = 4$
• $\sqrt{125} = 5\sqrt{5}$
• $\sqrt{3} = \sqrt{3}$

Identify relationships among these values to find the missing number. Since:

• $5$ is a result of $\sqrt{25}$
• $4$ is from $\sqrt{16}$

To find the missing number we need the pattern between these values. Specifically, observe how many times each simplifies or how they relate.

6. Identify the missing number

Based on the pattern from the previous computations, we can estimate the missing value by completing the visual sequence from the puzzle. The simplification should align with logical steps previously taken.