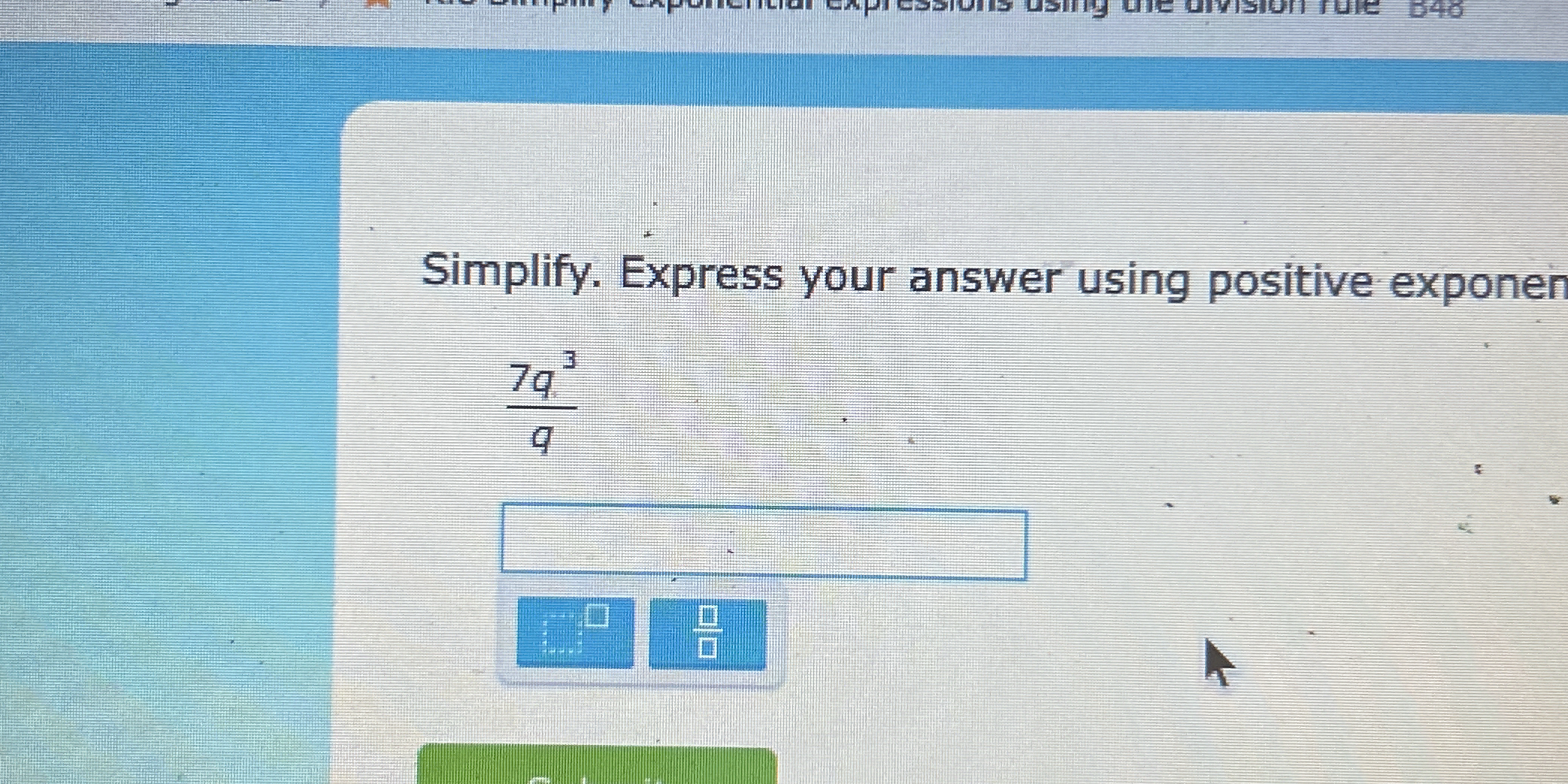
Simplify 7q^3/q. Express your answer using positive exponents.
Understand the Problem
The question is asking for the simplification of the expression 7q^3/q using the division rule of exponents and to express the answer using positive exponents.
Answer
The simplified expression is \( 7q^2 \).
Answer for screen readers
The simplified expression is ( 7q^2 ).
Steps to Solve
-
Identify the expression We start with the expression $$ \frac{7q^3}{q} $$.
-
Apply the division rule of exponents When dividing terms with the same base, we subtract the exponents. Therefore, we can write: $$ q^3 \div q = q^{3-1} = q^2 $$.
-
Write the simplified expression Now substitute back into the expression: $$ \frac{7q^3}{q} = 7 \cdot q^2 $$.
-
Final expression with positive exponent The simplified form is: $$ 7q^2 $$.
The simplified expression is ( 7q^2 ).
More Information
This expression shows the product of a constant (7) and a power of the variable ( q ). The division rule of exponents is essential when simplifying fractions involving exponential terms.
Tips
- Forgetting to apply the exponent subtraction correctly.
- Not simplifying all parts of the expression. Ensure to simplify both the coefficient and the variable.
AI-generated content may contain errors. Please verify critical information