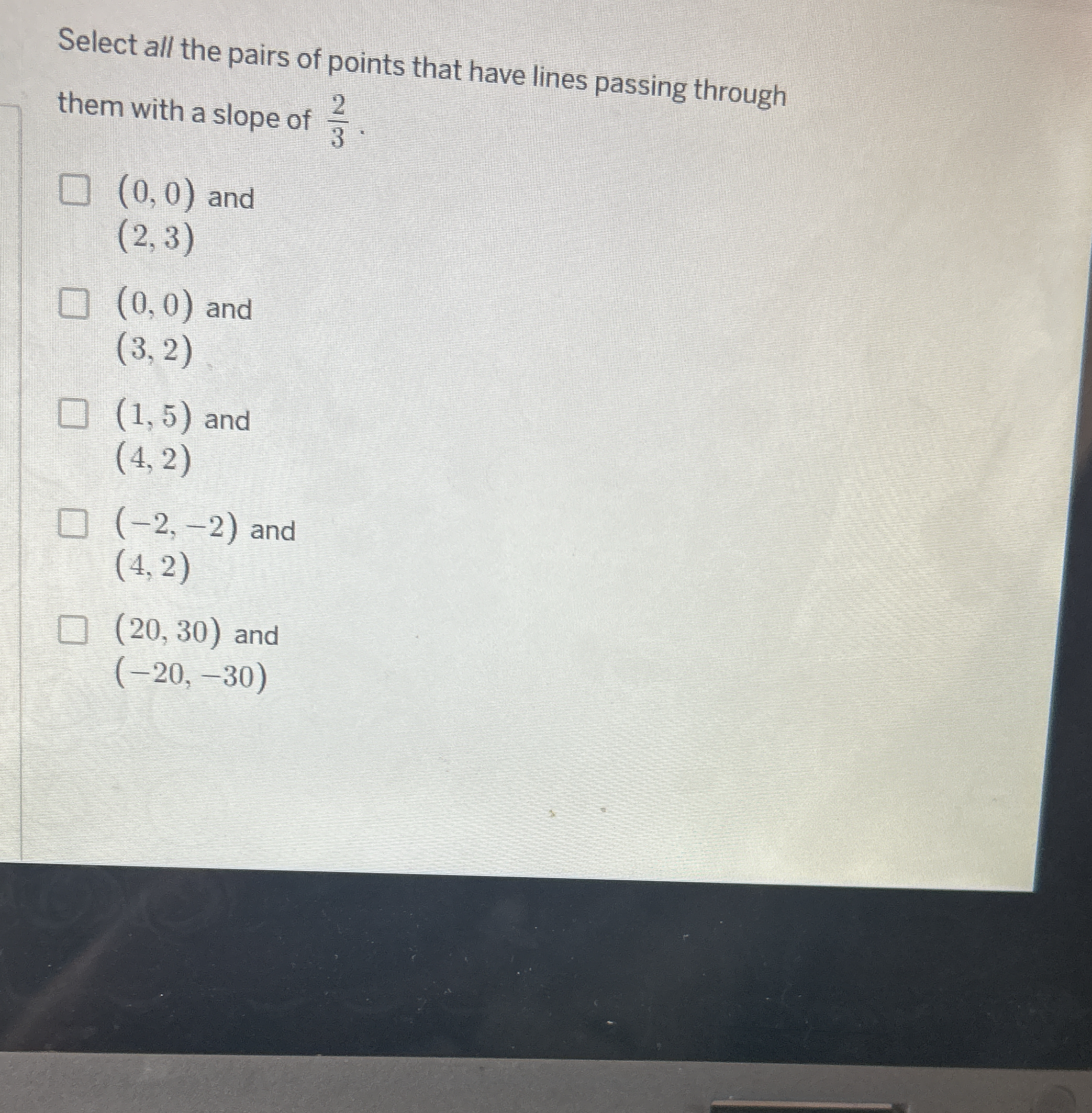
Select all the pairs of points that have lines passing through them with a slope of 2/3.

Steps to Solve

1. Slope Formula Introduction

To determine if the pairs of points have a slope of ( \frac{2}{3} ), we'll use the slope formula: $$ m = \frac{y_2 - y_1}{x_2 - x_1} $$ where ( (x_1, y_1) ) and ( (x_2, y_2) ) are the coordinates of the two points.

2. Calculate Slope for Each Pair

We'll calculate the slope for each pair of points.

Pair 1: (0, 0) and (2, 3)

$$ m = \frac{3 - 0}{2 - 0} = \frac{3}{2} $$

Pair 2: (0, 0) and (3, 2)

$$ m = \frac{2 - 0}{3 - 0} = \frac{2}{3} $$

Pair 3: (1, 5) and (4, 2)

$$ m = \frac{2 - 5}{4 - 1} = \frac{-3}{3} = -1 $$

Pair 4: (-2, -2) and (4, 2)

$$ m = \frac{2 - (-2)}{4 - (-2)} = \frac{4}{6} = \frac{2}{3} $$

Pair 5: (20, 30) and (-20, -30)

$$ m = \frac{-30 - 30}{-20 - 20} = \frac{-60}{-40} = \frac{3}{2} $$

3. Identify Matching Slopes

Now, we check which slopes equal ( \frac{2}{3} ):

• Pair 1: ( \frac{3}{2} )
• Pair 2: ( \frac{2}{3} ) (Match)
• Pair 3: ( -1 )
• Pair 4: ( \frac{2}{3} ) (Match)
• Pair 5: ( \frac{3}{2} )