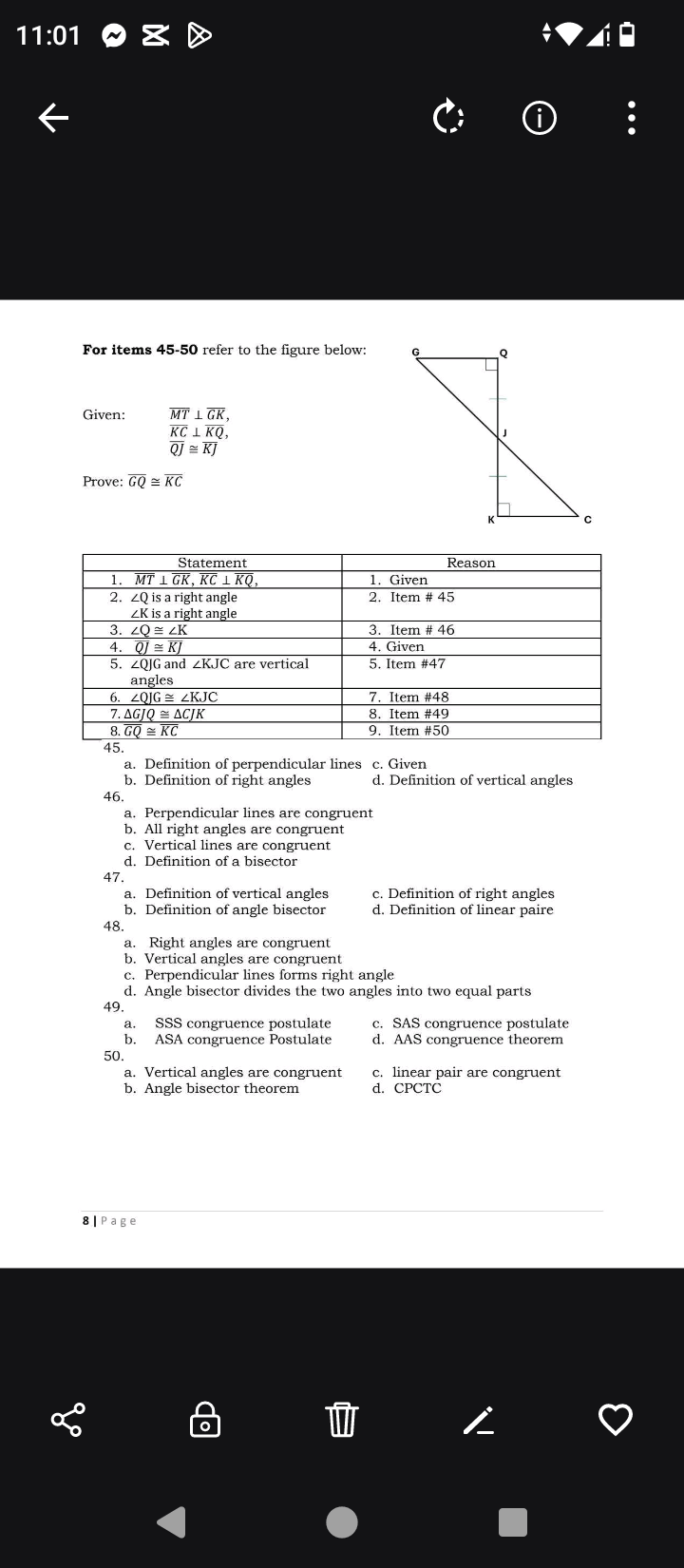
Refer to the figure, given MT ⊥ GK, KC ⊥ KQ, QJ ≅ KJ. Prove GQ ≅ KC. Answer items 45-50.
Understand the Problem
The image presents a geometry proof problem. It gives a diagram, provides some initial statements (Given), and asks to prove a final statement. Numbers 45-50 are questions about the steps and reasons of the proof which require knowledge of geometric theorems, definitions, and postulates.
Answer
$GQ \cong KC$
Answer for screen readers
$GQ \cong KC$
Steps to Solve
-
Identify the given information The statement starts with two perpendicular lines: $MT \perp GK$ and $KC \perp KQ$. This also implies that $\angle Q$ and $\angle K$ are right angles.
-
Establish the right angles Since both $\angle Q$ and $\angle K$ are right angles, we can use the property that all right angles are congruent. Thus, $\angle Q \cong \angle K$.
-
Recognize vertical angles The angles $\angle QJG$ and $\angle KJC$ are vertical angles formed by the intersection of two lines, hence they are congruent: $\angle QJG \cong \angle KJC$.
-
Apply triangle congruence Using the Angle-Side-Angle (ASA) postulate, since we have two congruent angles and a congruent side between them ($QJ \cong KJ$), we can conclude that $\triangle GJQ \cong \triangle CJK$.
-
Derive the final conclusion From the congruence of triangles, we utilize the Corresponding Parts of Congruent Triangles are Congruent (CPCTC) principle to conclude that $GQ \cong KC$.
$GQ \cong KC$
More Information
This proof applies the properties of right angles, vertical angles, and triangle congruence to establish the relationship between the line segments $GQ$ and $KC$. Recognizing the relationships of angles and sides based on geometric definitions and postulates is key in proofs.
Tips
- Confusing the definitions of right angles and vertical angles. It's important to remember that right angles are congruent by definition, while vertical angles are congruent due to their geometric positioning.
- Misapplying the triangle congruence postulates. Ensure the correct relationships (angles and sides) are established for the respective triangles to apply the postulates properly.
AI-generated content may contain errors. Please verify critical information