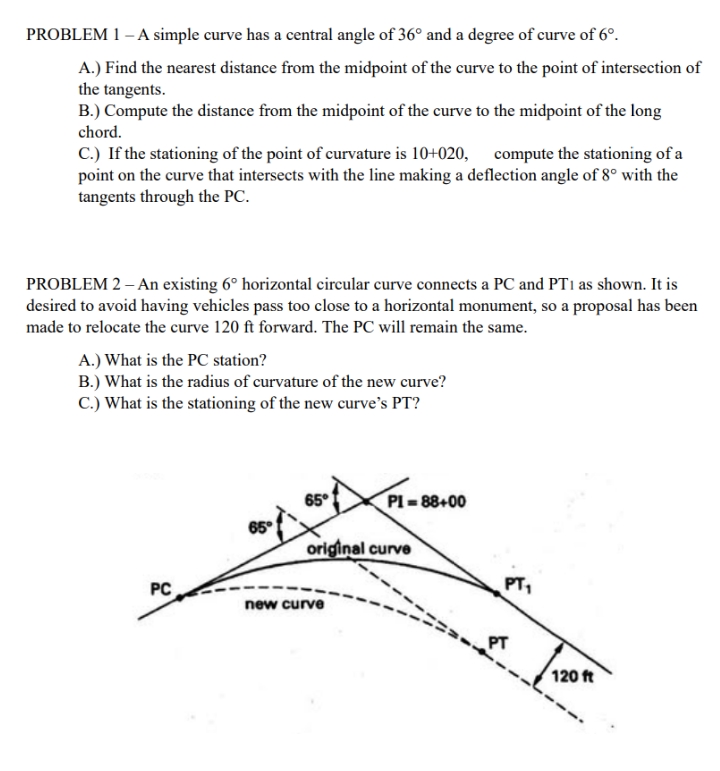
PROBLEM 1 – A simple curve has a central angle of 36° and a degree of curve of 6°. A.) Find the nearest distance from the midpoint of the curve to the point of intersection of the... PROBLEM 1 – A simple curve has a central angle of 36° and a degree of curve of 6°. A.) Find the nearest distance from the midpoint of the curve to the point of intersection of the tangents. B.) Compute the distance from the midpoint of the curve to the midpoint of the long chord. C.) If the stationing of the point of curvature is 10+020, compute the stationing of a point on the curve that intersects with the line making a deflection angle of 8° with the tangents through the PC. PROBLEM 2 – An existing 6° horizontal circular curve connects a PC and PT1 as shown. It is desired to avoid having vehicles pass too close to a horizontal monument, so a proposal has been made to relocate the curve 120 ft forward. The PC will remain the same. A.) What is the PC station? B.) What is the radius of curvature of the new curve? C.) What is the stationing of the new curve’s PT? Read more

Steps to Solve

PROBLEM 1

1. Finding the Radius of the Curve The radius $R$ of the curve can be calculated using the degree of curvature (DC): $$ R = \frac{5729.58}{DC} $$ Thus, for $DC = 6°$: $$ R = \frac{5729.58}{6} \approx 954.93 \text{ ft} $$

2. Calculating the Nearest Distance to Point of Intersection The distance from the midpoint of the curve ($D$) to the point of intersection (PI) is given by: $$ D = R(1 - \cos\left(\frac{\theta}{2}\right)) $$ where $\theta$ is the central angle. Thus, for $\theta = 36°$: $$ D = R(1 - \cos(18°)) $$ Substitute the value of $R$: $$ D \approx 954.93(1 - \cos(18°)) $$

3. Computing the Midpoint of the Long Chord The length of the long chord $L$ can be found using: $$ L = 2R \sin\left(\frac{\theta}{2}\right) $$ Thus: $$ L = 2 \times 954.93 \times \sin(18°) $$ The distance from the midpoint of the curve to the midpoint of the long chord $D_m$ is: $$ D_m = \frac{L}{2} $$

4. Calculating the Stationing of the Point on the Curve To find the stationing of a point making a deflection angle of $8°$:

   • First find the angle along the arc corresponding to $8°$ called $\theta' = \theta + 8$.
   • Then compute the distance along the curve to this point: $$ s = R \cdot \theta' $$

PROBLEM 2

1. Determining the PC Station The PC station remains the same as given in the problem, which is $PC = 88 + 00$.

2. Finding the Radius of the New Curve For the new curve that has been moved forward by $120$ ft, the radius stays as before (which was $954.93$ ft) because the degree of curvature hasn't changed.

3. Calculating the Stationing of the New Curve’s PT The PT station can be calculated by taking the PC station and adding the length of the new curve $L_{new}$, where: $$ L_{new} = 2R \cdot \sin\left(\frac{\theta}{2}\right) $$ This will give: $$ PT = PC + L_{new} $$