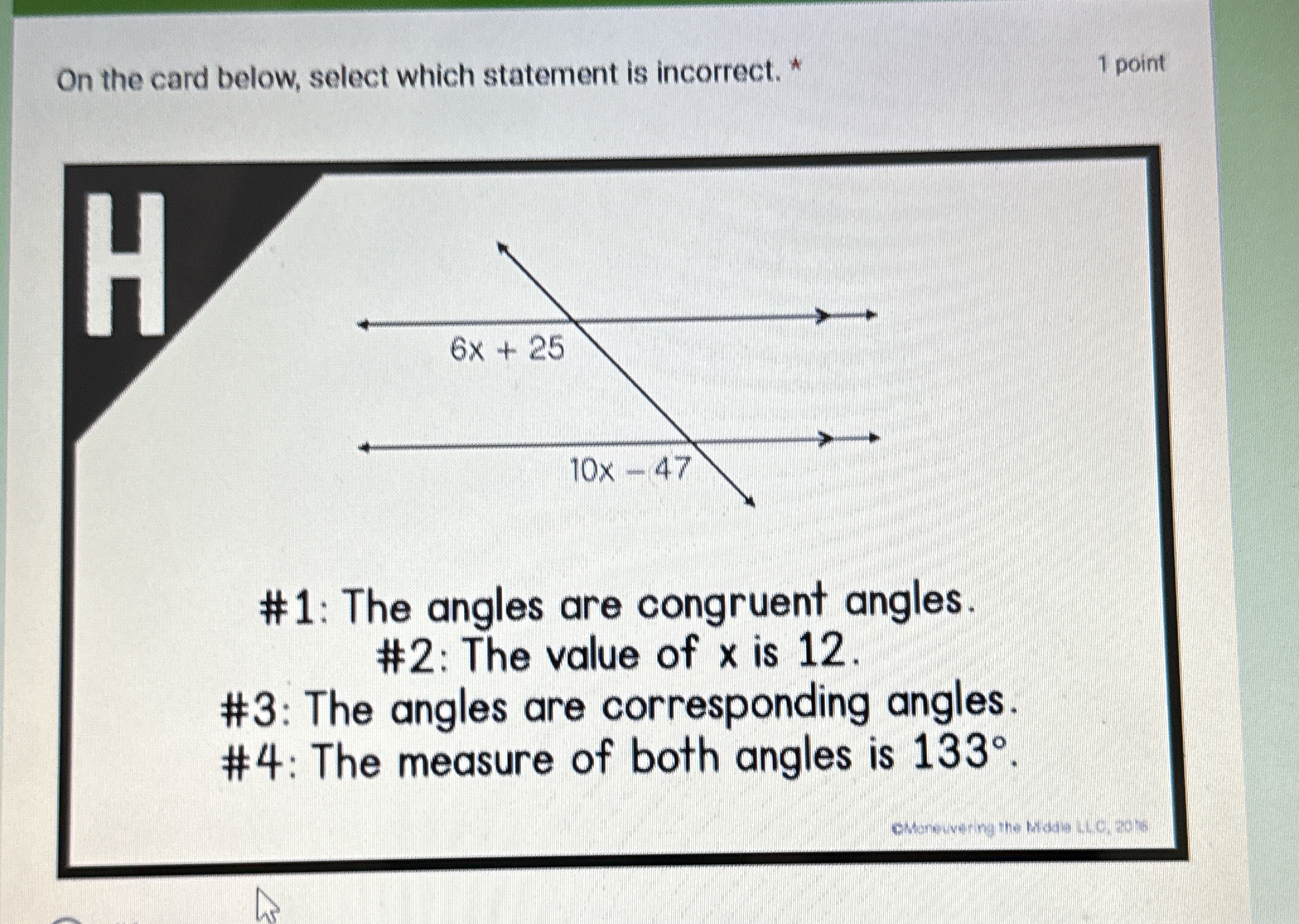
On the card below, select which statement is incorrect: #1: The angles are congruent angles. #2: The value of x is 12. #3: The angles are corresponding angles. #4: The measure of b... On the card below, select which statement is incorrect: #1: The angles are congruent angles. #2: The value of x is 12. #3: The angles are corresponding angles. #4: The measure of both angles is 133°. Read more

Steps to Solve

1. Set Up the Equation for the Angles

We know that the two angles formed by the intersecting lines are given by:

• Angle 1: $6x + 25$
• Angle 2: $10x - 47$

Since these angles are intersecting and form linear pairs, they must be equal, therefore we set up the equation:

$$ 6x + 25 = 10x - 47 $$

2. Solve for x

Now, we solve for $x$ by rearranging the equation:

• First, move $6x$ to the right side:

$$ 25 = 10x - 6x - 47 $$

This simplifies to:

$$ 25 = 4x - 47 $$

• Next, add $47$ to both sides:

$$ 25 + 47 = 4x $$

So,

$$ 72 = 4x $$

• Finally, divide by $4$:

$$ x = \frac{72}{4} = 18 $$

3. Calculate the Angles Using x

Now that we have $x$, we substitute it back into the expressions for the angles:

• Angle 1:

$$ 6x + 25 = 6(18) + 25 = 108 + 25 = 133 $$

• Angle 2:

$$ 10x - 47 = 10(18) - 47 = 180 - 47 = 133 $$

4. Analyze the Statements

Now we can evaluate each statement:

• Statement 1: The angles are congruent angles. (True, both angles are $133^\circ$.)
• Statement 2: The value of x is 12. (False, we found $x = 18$.)
• Statement 3: The angles are corresponding angles. (True, they are formed by transversal cutting parallel lines.)
• Statement 4: The measure of both angles is $133^\circ$. (True.)

The incorrect statement is Statement 2.