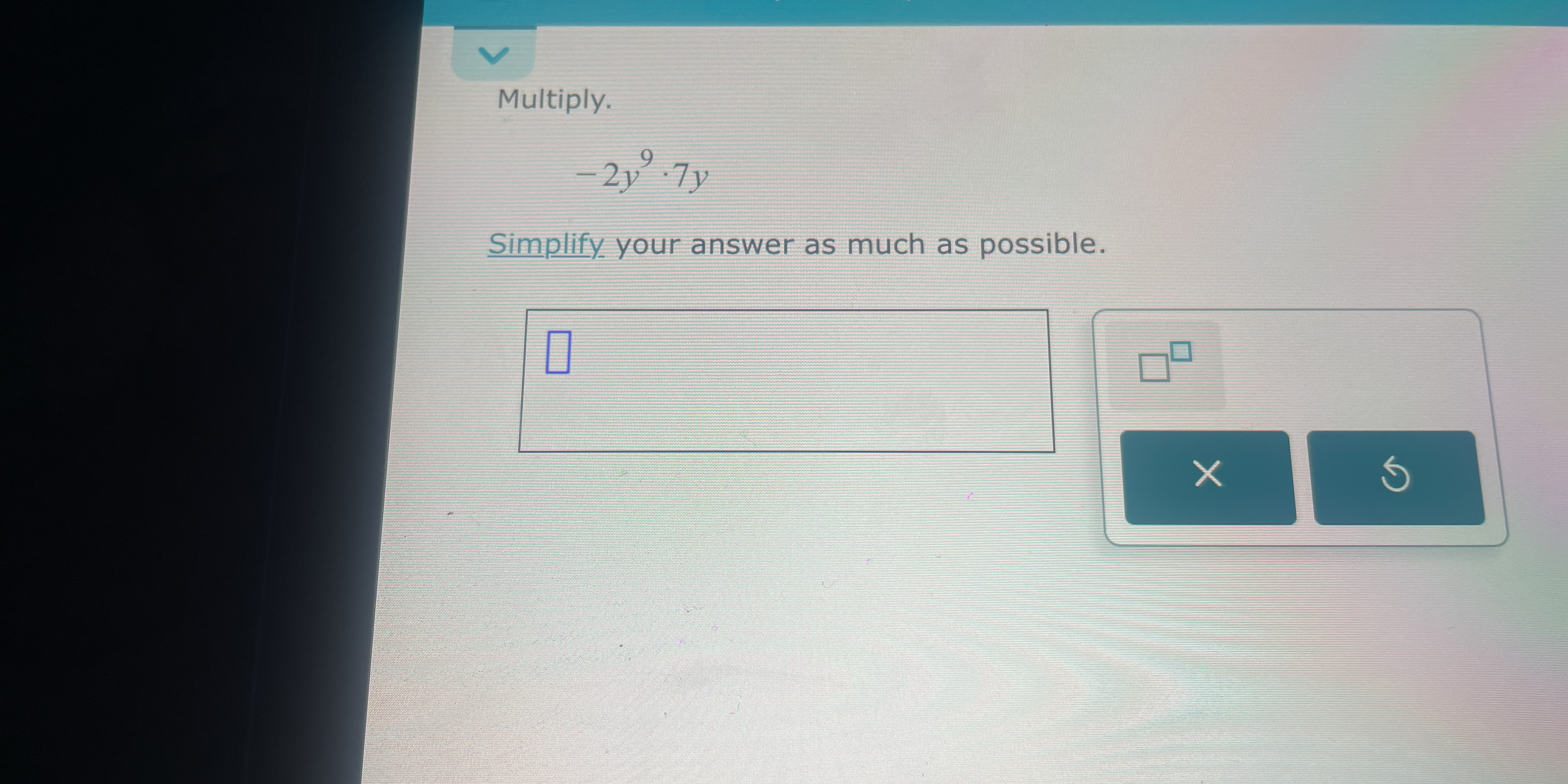
Multiply -2y^9 and 7y. Simplify your answer as much as possible.
Understand the Problem
The question is asking us to multiply the terms -2y^9 and 7y, and to simplify the result as much as possible.
Answer
The simplified answer is \( -14y^{10} \).
Answer for screen readers
The simplified result of multiplying the terms is ( -14y^{10} ).
Steps to Solve
- Multiply the coefficients
We start by multiplying the coefficients -2 and 7:
$$ -2 \times 7 = -14 $$
- Multiply the variables
Next, we multiply the variable terms ( y^9 ) and ( y ). When multiplying variables with the same base, we add their exponents:
$$ y^9 \cdot y^1 = y^{9 + 1} = y^{10} $$
- Combine results
Now we combine the results from steps 1 and 2 to form the final expression:
$$ -14y^{10} $$
The simplified result of multiplying the terms is ( -14y^{10} ).
More Information
The result ( -14y^{10} ) is a polynomial term where the coefficient is -14 and the variable ( y ) has an exponent of 10. This means that the term is negative and has a high degree, indicating that it will significantly influence the value of the polynomial as ( y ) increases.
Tips
- Forgetting to add exponents: When multiplying the variable terms, it’s easy to forget that you must add the exponents. Remember, when multiplying, use the rule ( a^m \cdot a^n = a^{m+n} ).
- Incorrectly multiplying coefficients: Double-check the multiplication of coefficients to avoid sign errors.
AI-generated content may contain errors. Please verify critical information