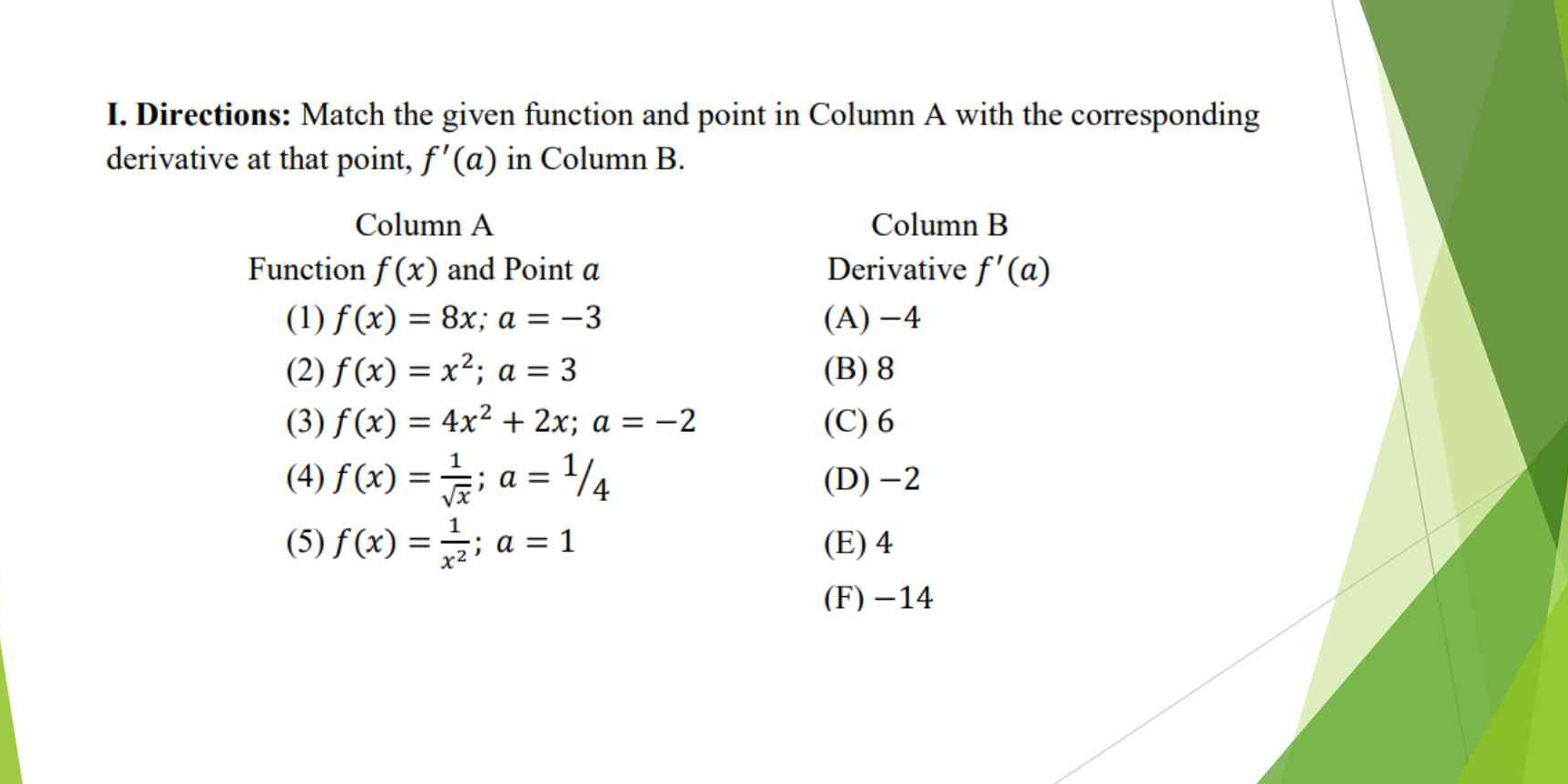
Match the given function and point in Column A with the corresponding derivative at that point, $f'(a)$ in Column B.
Understand the Problem
The question asks us to find the derivative of each given function $f(x)$ in Column A and then evaluate the derivative $f'(a)$ at the specified point $a$. Finally, we need to match the calculated derivative value with the corresponding value in Column B.
Answer
(1) $\rightarrow$ (B) (2) $\rightarrow$ (C) (3) $\rightarrow$ (F) (4) $\rightarrow$ (A) (5) $\rightarrow$ (D)
Answer for screen readers
(1) $\rightarrow$ (B) (2) $\rightarrow$ (C) (3) $\rightarrow$ (F) (4) $\rightarrow$ (A) (5) $\rightarrow$ (D)
Steps to Solve
- Find the derivative of $f(x) = 8x$ and evaluate at $a = -3$
The derivative of $f(x) = 8x$ is $f'(x) = 8$. Since the derivative is a constant, $f'(-3) = 8$. This matches with (B).
- Find the derivative of $f(x) = x^2$ and evaluate at $a = 3$
The derivative of $f(x) = x^2$ is $f'(x) = 2x$. Evaluating at $a = 3$, we get $f'(3) = 2(3) = 6$. This matches with (C).
- Find the derivative of $f(x) = 4x^2 + 2x$ and evaluate at $a = -2$
The derivative of $f(x) = 4x^2 + 2x$ is $f'(x) = 8x + 2$. Evaluating at $a = -2$, we get $f'(-2) = 8(-2) + 2 = -16 + 2 = -14$. This matches with (F).
- Find the derivative of $f(x) = \frac{1}{\sqrt{x}}$ and evaluate at $a = \frac{1}{4}$
First, rewrite the function as $f(x) = x^{-\frac{1}{2}}$. Then, the derivative is $f'(x) = -\frac{1}{2}x^{-\frac{3}{2}} = -\frac{1}{2\sqrt{x^3}}$. Evaluating at $a = \frac{1}{4}$, we have $f'(\frac{1}{4}) = -\frac{1}{2\sqrt{(\frac{1}{4})^3}} = -\frac{1}{2\sqrt{\frac{1}{64}}} = -\frac{1}{2(\frac{1}{8})} = -\frac{1}{\frac{1}{4}} = -4$. This matches with (A).
- Find the derivative of $f(x) = \frac{1}{x^2}$ and evaluate at $a = 1$
First, rewrite the function as $f(x) = x^{-2}$. Then, the derivative is $f'(x) = -2x^{-3} = -\frac{2}{x^3}$. Evaluating at $a = 1$, we have $f'(1) = -\frac{2}{1^3} = -2$. This matches with (D).
(1) $\rightarrow$ (B) (2) $\rightarrow$ (C) (3) $\rightarrow$ (F) (4) $\rightarrow$ (A) (5) $\rightarrow$ (D)
More Information
The derivative of a function represents the instantaneous rate of change of the function with respect to its variable. Evaluating the derivative at a specific point gives the slope of the tangent line to the function's graph at that point.
Tips
A common mistake is incorrectly applying the power rule when finding the derivative. For example, when differentiating $\frac{1}{\sqrt{x}} = x^{-\frac{1}{2}}$, some may forget the negative sign or incorrectly calculate the new exponent. Also, not simplifying after taking the derivative, which can make the evaluation more difficult. Another common mistake could be evaluating the original function at $a$ instead of the derivative.
AI-generated content may contain errors. Please verify critical information