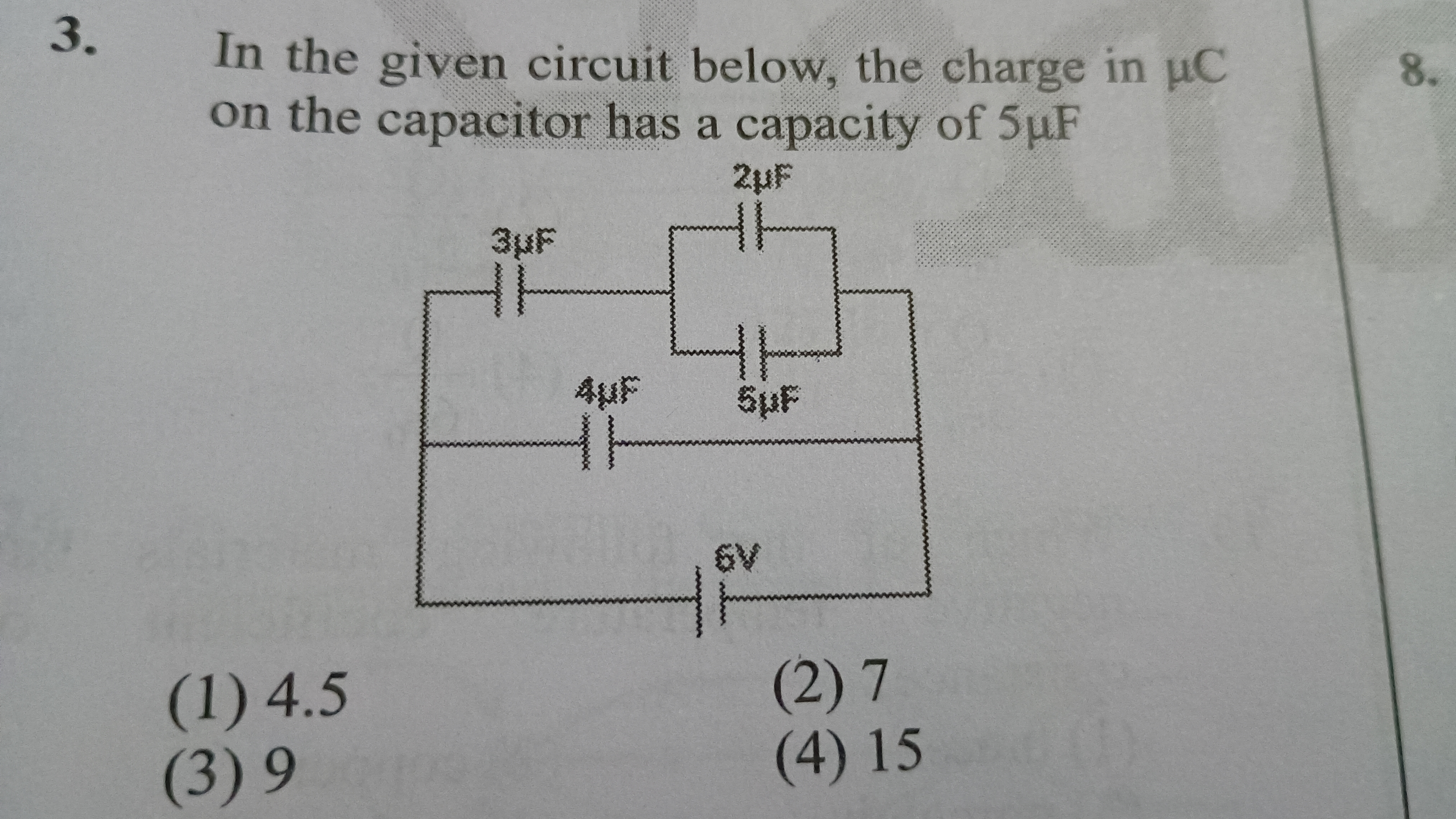
In the given circuit below, the charge in µC on the capacitor has a capacity of 5 µF.

Steps to Solve

1. Identify the Capacitors and Their Configuration

In the given circuit, we have a combination of capacitors: a 2 µF capacitor in parallel with an equivalent capacitor formed by a 5 µF and 4 µF capacitor in series.

2. Calculate the Equivalent Capacitance of Series Capacitors

For capacitors in series, the equivalent capacitance ($C_{eq1}$) is calculated using the formula:

$$ \frac{1}{C_{eq1}} = \frac{1}{C_1} + \frac{1}{C_2} $$

Here, $C_1 = 5 , \mu F$ and $C_2 = 4 , \mu F$:

$$ \frac{1}{C_{eq1}} = \frac{1}{5} + \frac{1}{4} $$

Calculating gives:

$$ \frac{1}{C_{eq1}} = \frac{4 + 5}{20} = \frac{9}{20} $$

Thus,

$$ C_{eq1} = \frac{20}{9} , \mu F \approx 2.22 , \mu F $$

3. Determine the Total Capacitance in Parallel

Now, we place the 2 µF capacitor in parallel with the equivalent capacitance we just found:

$$ C_{eq2} = C_{parallel} + C_{eq1} = 2 , \mu F + \frac{20}{9} , \mu F $$

Converting (2 , \mu F) to a fraction:

$$ C_{eq2} = 2 + \frac{20}{9} = \frac{18}{9} + \frac{20}{9} = \frac{38}{9} , \mu F \approx 4.22 , \mu F $$

4. Calculate the Total Charge on the Capacitor

Now we can find the total charge ($Q$) stored in the capacitor using the formula:

$$ Q = C_{eq2} \cdot V $$

Where (V = 6 , V) is the voltage across the capacitors.

Substituting values:

$$ Q = \frac{38}{9} , \mu F \cdot 6 , V = \frac{228}{9} , \mu C \approx 25.33 , \mu C $$

5. Charge on the 5 µF Capacitor

The charge across each capacitor in a parallel configuration is the same, so the charge on the 5 µF capacitor is:

$$ Q_{5 , \mu F} = C_{5 , \mu F} \cdot V_{5 , \mu F} $$

Using (C_{eq2}) calculated earlier for direct voltage:

Assuming full voltage across entire series setup we have:

$$ Q = 5 , \mu F \cdot V = 5 , \mu F \cdot 6 , V $$

Solving gives:

$$ Q = 30, \mu C $$

In terms of maximum seen charge in configuration fo the series setup the total can be computed if necessary.