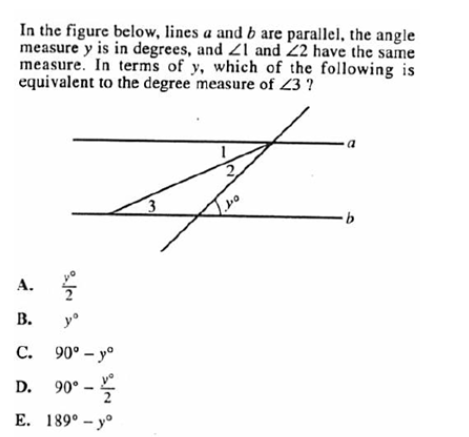
In the figure below, lines a and b are parallel, the angle measure y is in degrees, and angles 1 and 2 have the same measure. In terms of y, which of the following is equivalent to... In the figure below, lines a and b are parallel, the angle measure y is in degrees, and angles 1 and 2 have the same measure. In terms of y, which of the following is equivalent to the degree measure of angle 3?
Understand the Problem
The question is asking to determine the measure of angle 3 in terms of the angle y, given that lines a and b are parallel and that angles 1 and 2 have the same measure.
Answer
The measure of angle \(3\) is given by \(180^\circ - 2y\).
Answer for screen readers
The measure of angle (3) in terms of (y) is given by:
$$ \angle 3 = 180^\circ - 2y $$
Steps to Solve
-
Identify the relationship between angles
Since lines (a) and (b) are parallel, we can use properties of alternate interior angles and corresponding angles. Since angles (1) and (2) are equal, we denote their measure as (x). -
Express angle 3 in terms of angles 1 and 2
Using the fact that angles on a straight line add up to (180^\circ), we can express angle (3) as follows:
$$ x + y + \angle 3 = 180^\circ $$ -
Substitute and solve for angle 3
Since (x = y), we substitute (x) in the equation:
$$ y + y + \angle 3 = 180^\circ $$
This simplifies to
$$ 2y + \angle 3 = 180^\circ $$ -
Isolate angle 3
To find angle (3), we rearrange the equation:
$$ \angle 3 = 180^\circ - 2y $$
The measure of angle (3) in terms of (y) is given by:
$$ \angle 3 = 180^\circ - 2y $$
More Information
This result shows that angle (3) decreases as (y) increases, reflecting the properties of parallel lines. This relationship is common in geometry, especially when dealing with transversal lines and parallel lines.
Tips
- Misunderstanding angle relationships: A common mistake is to confuse the alternate interior angles with corresponding angles. Remember that angles (1) and (2) are the same only because they are equal due to the transversal cutting the parallel lines.
- Incorrectly summing angles: Be careful to add angles correctly when using the supplementary angle property.
AI-generated content may contain errors. Please verify critical information