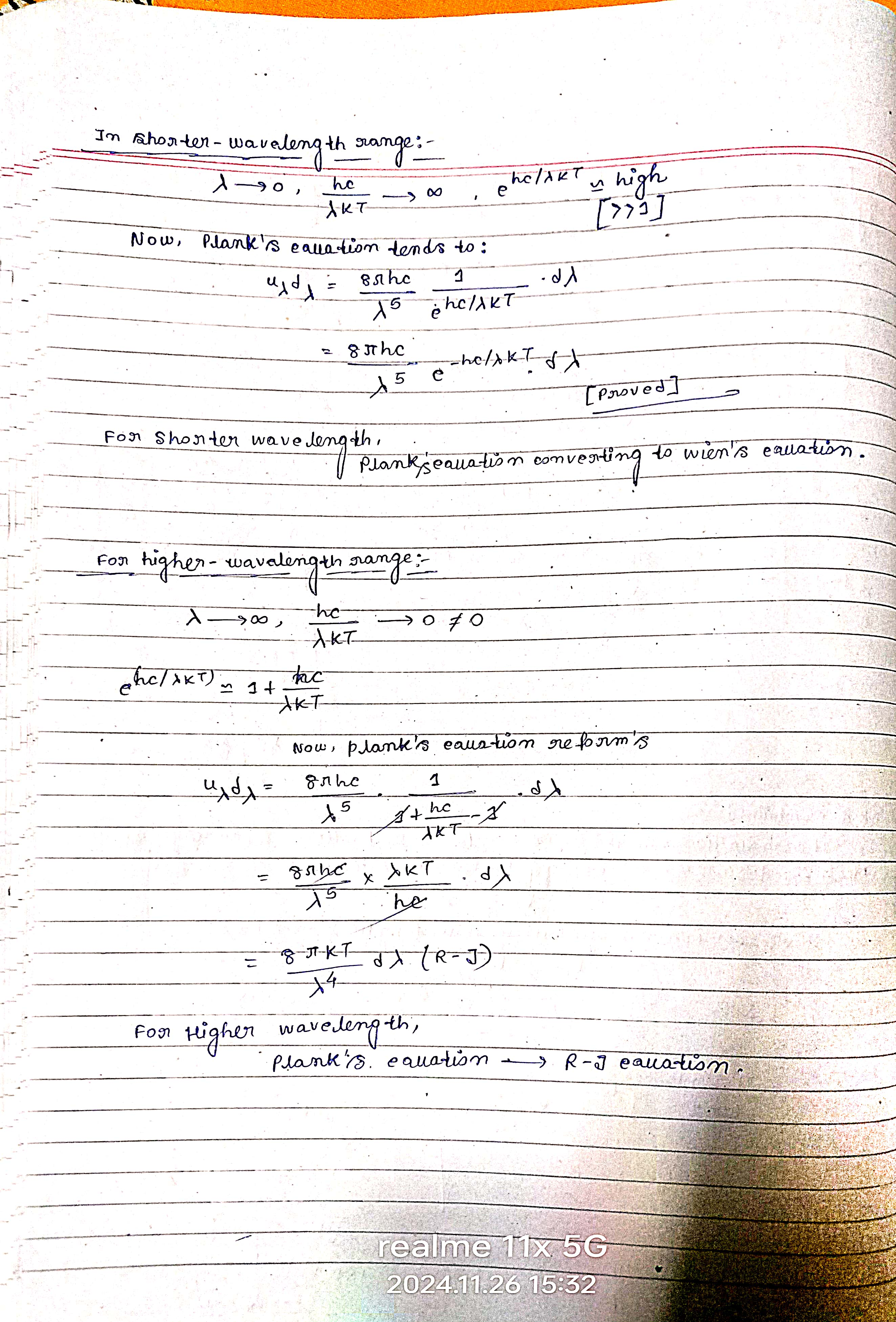
In shorter wavelength range: λ → 0, hc/λkt → ∞, e^(hc/λkt) → high. Now, Planck's equation tends to: ... For higher wavelength range, Planck's equation → R-J equation.
Understand the Problem
The question discusses the derivation and application of Planck's equation across different wavelength ranges, particularly how it relates to Wien’s law for shorter wavelengths and further forms for higher wavelengths. It seems to be focused on the mathematical transformation of these equations under specific conditions.
Answer
Planck's law becomes Wien's law at short wavelengths and Rayleigh-Jeans law at long wavelengths.
For shorter wavelength, Planck's equation tends to Wien's law. For higher wavelength, it approximates to the Rayleigh-Jeans law.
Answer for screen readers
For shorter wavelength, Planck's equation tends to Wien's law. For higher wavelength, it approximates to the Rayleigh-Jeans law.
More Information
In the context of thermal radiation, the transition from Planck's law to Wien's law and Rayleigh-Jeans law helps describe black body radiation behavior across different wavelength ranges.
Tips
Ensure to correctly identify the limits and approximations applied to the exponential terms in Planck's equation.
Sources
- Planck's law - Wikipedia - en.wikipedia.org
- Planck's Law in terms of wavelength - Physics Stack Exchange - physics.stackexchange.com
AI-generated content may contain errors. Please verify critical information