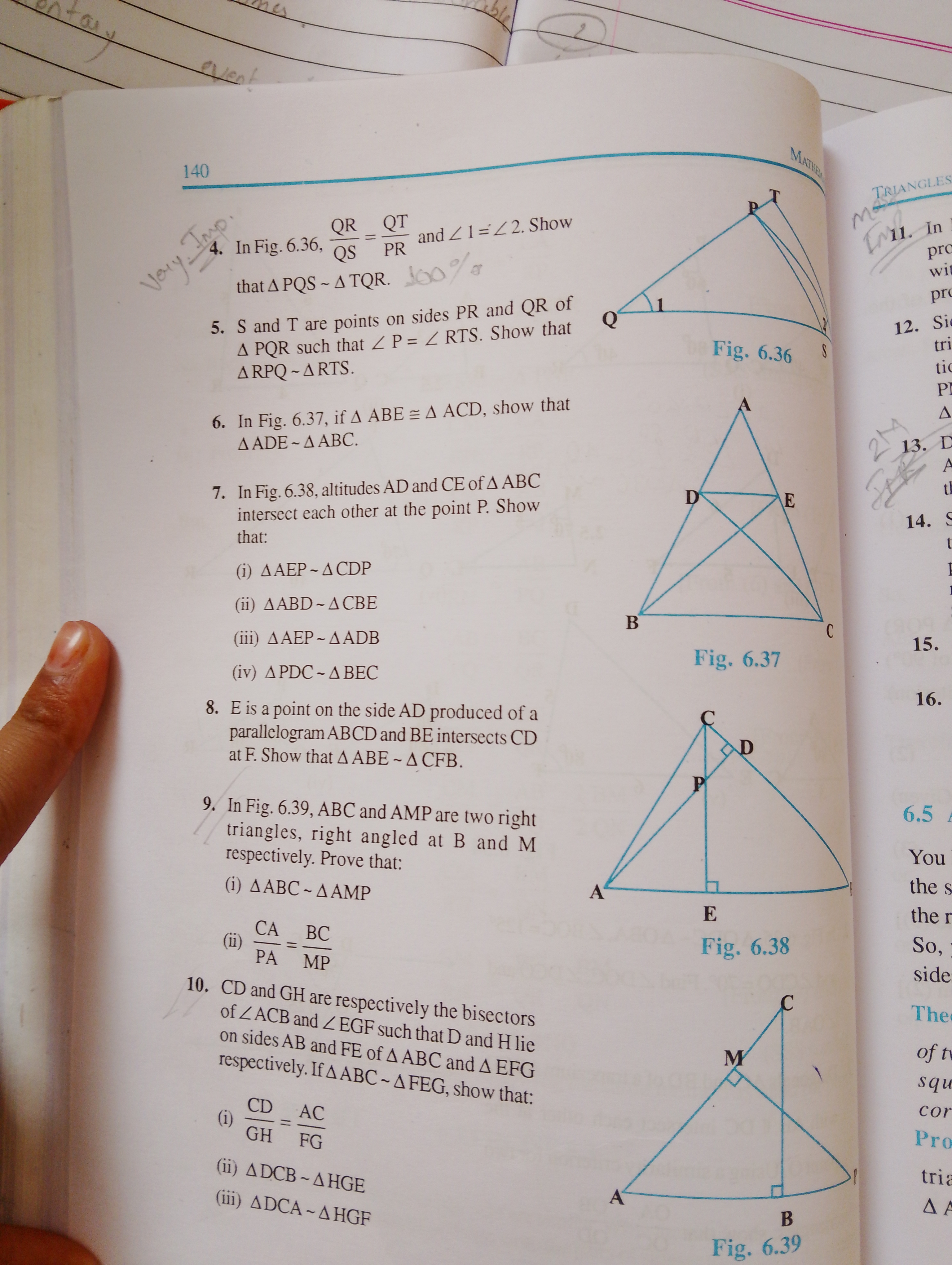
In Fig. 6.36, QR/ QS = QT/ PR and ∠1 = ∠2. Show that ΔPQS ~ ΔTQR. S and T are points on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ~ ΔRTS. In Fig. 6.37, if ΔABE ≅... In Fig. 6.36, QR/ QS = QT/ PR and ∠1 = ∠2. Show that ΔPQS ~ ΔTQR. S and T are points on sides PR and QR of ΔPQR such that ∠P = ∠RTS. Show that ΔRPQ ~ ΔRTS. In Fig. 6.37, if ΔABE ≅ ΔACD, show that ΔADE ≅ ΔABC. In Fig. 6.38, altitudes AD and CE of ΔABC intersect each other at the point P. Show that: (i) ΔAEP ≅ ΔCDP (ii) ΔABD ≅ ΔCBE (iii) ΔAED ≅ ΔADB (iv) ΔPDC ≅ ΔBEC. E is a point on the side AD produced of a parallelogram ABCD and BE intersects CD at F. Show that ΔABE ≅ ΔCFB. In Fig. 6.39, ABC and AMP are two right triangles, right angled at B and M respectively. Prove that: (i) ΔABC ≅ ΔAMP (ii) CA/PA = BC/MP. CD and GH are respectively the bisectors of ∠ACB and ∠EGF such that D and H lie on sides AB and FE of ΔABC and ΔEFG respectively. If ΔABC ≅ ΔFEG, show that: (i) CD/GH = AC/FG (ii) ΔDCB ≅ ΔDGE (iii) ΔDCA ≅ ΔHGF.
Understand the Problem
The question is asking for geometric proofs related to triangles and their properties, focusing on congruence and equality of angles and segments. The problems involve proving congruence or equality in different configurations of triangles.
Answer
The triangles are congruent: $\triangle AEP \cong \triangle CDP$.
Answer for screen readers
The triangles $\triangle AEP \cong \triangle CDP$.
Steps to Solve
-
Understanding Given Information
We have two triangles in question: triangle $AEP$ and triangle $CDP$. We're given that either the sides or angles in these triangles correspond and need to prove their congruence. -
Identify Congruent Parts
From the provided angles, observe:
- Given: $\angle P$ is common to both triangles.
- We're also looking at other parts of the triangles—identify any equal sides or angles.
-
Apply Congruence Criteria
Use the criteria for establishing triangle congruence. The common congruence rules include:
- Side-Angle-Side (SAS): If two sides and the included angle of one triangle are equal to two sides and the included angle of another triangle.
-
Angle-Side-Angle (ASA): If two angles and the included side of one triangle are equal to two angles and the included side of the other triangle.
We will find which criteria fit the information we have.
-
Use Equal Measurements
Using equal angles and segments:
- If $\angle AEP = \angle ADB$ can be shown via some previous postulates or theorems.
- If $AE = CD$ and $EP = DP$, you can use SAS or ASA for equating the triangles.
-
Conclusion
Combine the information and criteria discussed to conclude that $\triangle AEP \cong \triangle CDP$.
This indicates that they are congruent based on whichever criteria applies.
The triangles $\triangle AEP \cong \triangle CDP$.
More Information
Triangles are congruent when they have the same shape and size, regardless of their orientation. Congruence can be established through the Side-Angle-Side (SAS) or Angle-Side-Angle (ASA) criteria, which are fundamental in geometry.
Tips
- Forgetting to clearly state which parts are congruent can lead to confusion about how to prove the triangles are congruent.
- Misidentifying the angle or side relationships can result in incorrect conclusions regarding congruence.