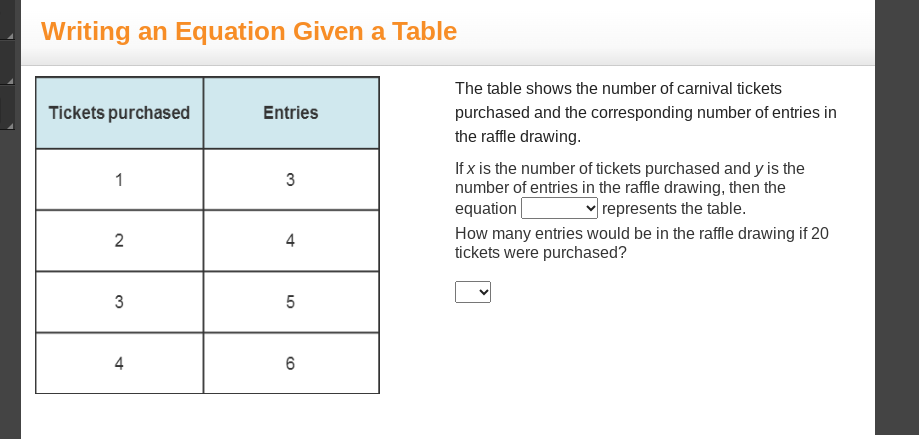
If x is the number of tickets purchased and y is the number of entries in the raffle drawing, then what equation represents the table? How many entries would be in the raffle drawi... If x is the number of tickets purchased and y is the number of entries in the raffle drawing, then what equation represents the table? How many entries would be in the raffle drawing if 20 tickets were purchased?
Understand the Problem
The question is asking us to determine the relationship between the number of tickets purchased and the corresponding entries in a raffle. It requires us to derive an equation based on the table provided and then calculate the number of raffle entries for a specified number of tickets (20).
Answer
The number of entries for 20 tickets is \( 22 \).
Answer for screen readers
The number of entries in the raffle drawing when 20 tickets are purchased is ( 22 ).
Steps to Solve
- Identify the relationship between tickets and entries
From the table, we see the following data:
- When 1 ticket is purchased, there are 3 entries.
- When 2 tickets are purchased, there are 4 entries.
- When 3 tickets are purchased, there are 5 entries.
- When 4 tickets are purchased, there are 6 entries.
This shows that for each additional ticket purchased, the number of entries increases by 1.
- Determine the equation
Let ( x ) be the number of tickets purchased and ( y ) be the number of entries in the raffle.
From the observed pattern, we can establish the relationship:
$$ y = x + 2 $$
- Calculate entries for 20 tickets
To find the number of entries for 20 tickets, substitute ( x = 20 ) into the equation:
$$ y = 20 + 2 $$
- Final calculation
Now, compute the value:
$$ y = 22 $$
Thus, the number of entries when 20 tickets are purchased is 22.
The number of entries in the raffle drawing when 20 tickets are purchased is ( 22 ).
More Information
The relationship was identified as linear, showing a consistent increase in raffle entries with each ticket purchased. This scenario illustrates a simple linear equation where a base number of entries (2) is added to the number of tickets purchased.
Tips
- Confusing the relationship: It's important to recognize that the entries increase by 1 for each ticket after the base entry. Not accounting for the initial entries can lead to incorrect calculations.
- Miscalculating the addition in the final step: Double-checking the arithmetic is key to ensuring you arrive at the correct number of entries.
AI-generated content may contain errors. Please verify critical information