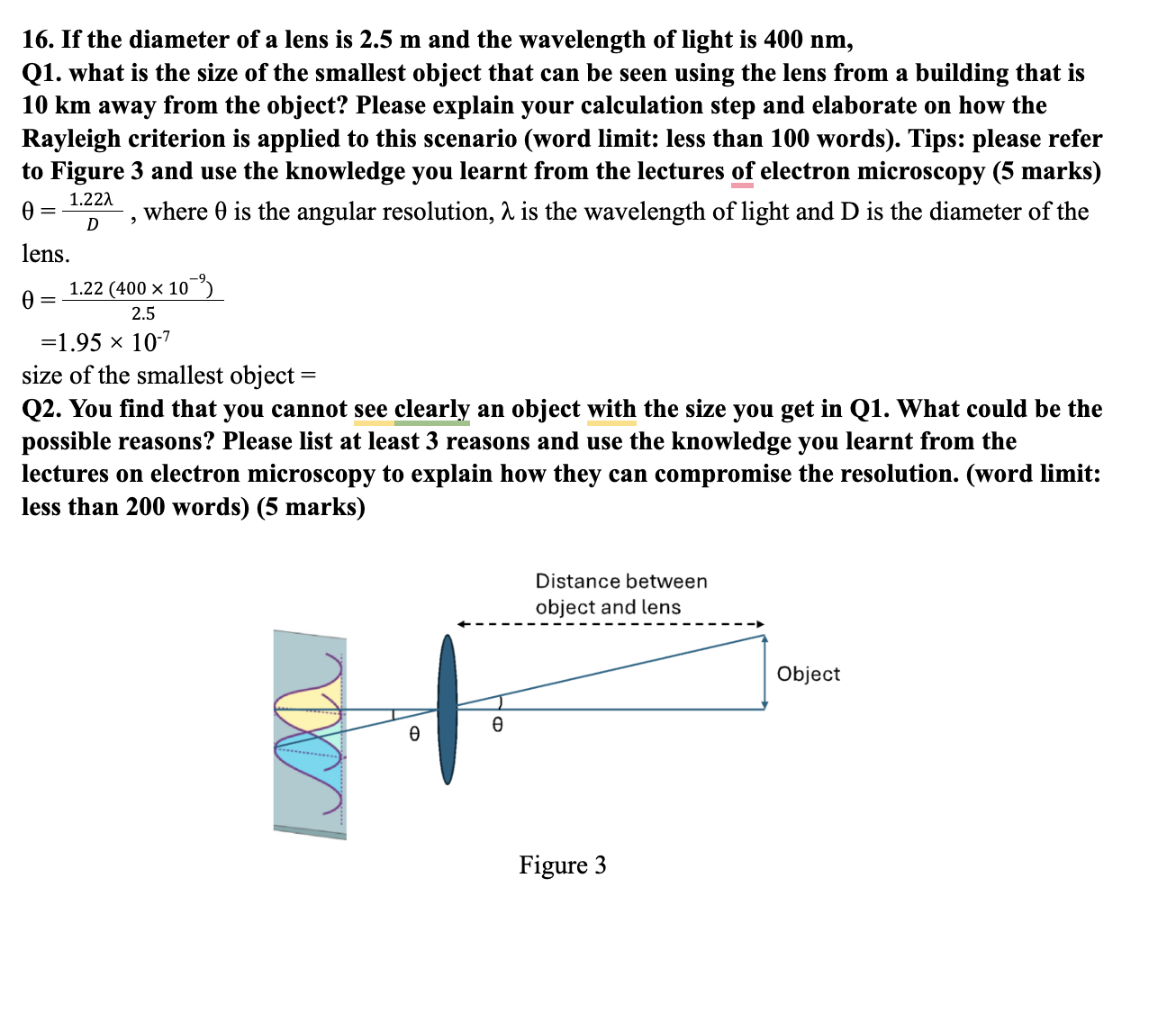
If the diameter of a lens is 2.5 m and the wavelength of light is 400 nm, what is the size of the smallest object that can be seen using the lens from a building that is 10 km away... If the diameter of a lens is 2.5 m and the wavelength of light is 400 nm, what is the size of the smallest object that can be seen using the lens from a building that is 10 km away from the object? Please explain your calculation step and elaborate on how the Rayleigh criterion is applied to this scenario. You find that you cannot see clearly an object with the size you get in the first question. What could be the possible reasons? Please list at least 3 reasons and explain how they can compromise the resolution.
Understand the Problem
The question consists of two parts. The first part asks to calculate the size of the smallest object that can be seen using a lens given its diameter and the wavelength of light, applying the Rayleigh criterion for resolution. The second part asks for possible reasons why an object of that calculated size may not be clearly visible, requiring a discussion based on knowledge of electron microscopy.
Answer
The size of the smallest object that can be seen is approximately $1.95 \text{ mm}$.
Answer for screen readers
The size of the smallest object that can be seen using the lens is approximately $1.95 \text{ mm}$.
Steps to Solve
- Understand the Rayleigh Criterion for Resolution
The Rayleigh criterion states that the minimum angular resolution $\theta$ of a lens can be approximated by the formula:
$$ \theta = \frac{1.22 \lambda}{D} $$
where $\lambda$ is the wavelength of light in meters, and $D$ is the diameter of the lens in meters.
- Convert Wavelength Units
Given the wavelength of light is 400 nm, we first convert this to meters.
$$ 400 \text{ nm} = 400 \times 10^{-9} \text{ m} = 4.00 \times 10^{-7} \text{ m} $$
- Calculate the Angular Resolution
Using the diameter of the lens ($D = 2.5 \text{ m}$) and the wavelength ($\lambda = 4.00 \times 10^{-7} \text{ m}$), we calculate the angular resolution $\theta$:
$$ \theta = \frac{1.22 \times (4.00 \times 10^{-7})}{2.5} $$
Calculating this gives:
$$ \theta \approx 1.95 \times 10^{-7} \text{ radians} $$
- Calculate the Size of the Smallest Object
To find the size of the smallest object $s$ that can be resolved at a distance $L$ (10 km or $10,000 \text{ m}$), we use:
$$ s = L \cdot \theta $$
Substituting the values:
$$ s = 10,000 \times (1.95 \times 10^{-7}) $$
Calculating this gives:
$$ s \approx 1.95 \times 10^{-3} \text{ m} = 1.95 \text{ mm} $$
The size of the smallest object that can be seen using the lens is approximately $1.95 \text{ mm}$.
More Information
The Rayleigh criterion is fundamental in optics, determining the limits of resolution that can be achieved with optical instruments. For this case, the calculated size indicates that objects smaller than 1.95 mm will not be distinguishable with the given lens and wavelength of light.
Tips
- Incorrect unit conversion: Ensure wavelength is converted correctly from nanometers to meters.
- Failure to apply the formula correctly: Carefully substitute values into the Rayleigh criterion equation.
- Ignoring the distance effect: Remember that the object size resolution is directly affected by the distance from the lens.
AI-generated content may contain errors. Please verify critical information