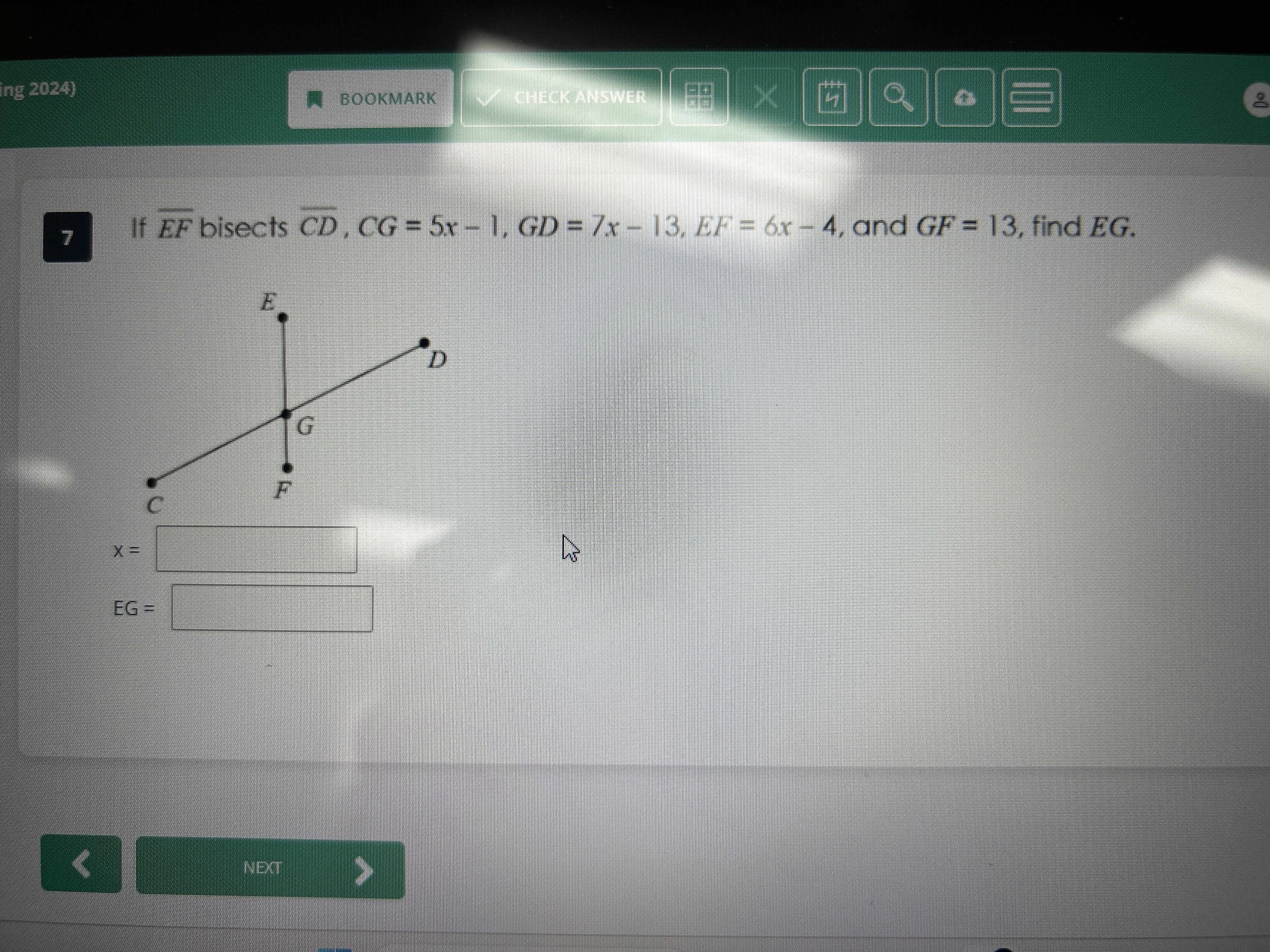
If EF bisects CD, CG = 5x - 1, GD = 7x - 13, EF = 6x - 4, and GF = 13, find EG.

Understand the Problem

The question is asking to find the value of x and the length of segment EG, given various segment lengths and the condition that EF bisects CD. This involves setting up equations based on the bisector properties and solving for the unknowns.

Answer

$x = 6$, $ EG = 16 $

Steps to Solve

Set up the equation for the bisector Since EF bisects CD, we know that ( CG = GD ). We have ( CG = 5x - 1 ) and ( GD = 7x - 13 ). Therefore, we set up the equation: $$ 5x - 1 = 7x - 13 $$
Solve for x To solve the equation, we first rearrange it to isolate ( x ): $$ 5x - 7x = -13 + 1 $$ This simplifies to: $$ -2x = -12 $$ Dividing both sides by -2 gives: $$ x = 6 $$
Calculate the lengths of CG and GD Now that we have ( x = 6 ), we substitute it back into the expressions for CG and GD: $$ CG = 5(6) - 1 = 30 - 1 = 29 $$ $$ GD = 7(6) - 13 = 42 - 13 = 29 $$
Confirm equality of CG and GD Since both segments are equal and ( EF ) indeed bisects ( CD ), we have confirmed the calculation.
Find EG Given that ( GF = 13 ) and now we know ( CG = 29 ): To find ( EG ): $$ EG = CG - GF = 29 - 13 = 16 $$

The value of ( x ) is ( 6 ), and the length of segment ( EG ) is ( 16 ).

More Information

The problem involves segment relationships created by the angle bisector theorem, confirming properties of equality in bisected segments.

Tips

Forgetting to set up the equation correctly based on the bisector property.
Miscalculating when substituting to find ( x ).
Confusing segment lengths, especially when adding or subtracting segments.

AI-generated content may contain errors. Please verify critical information

Thank you for voting!