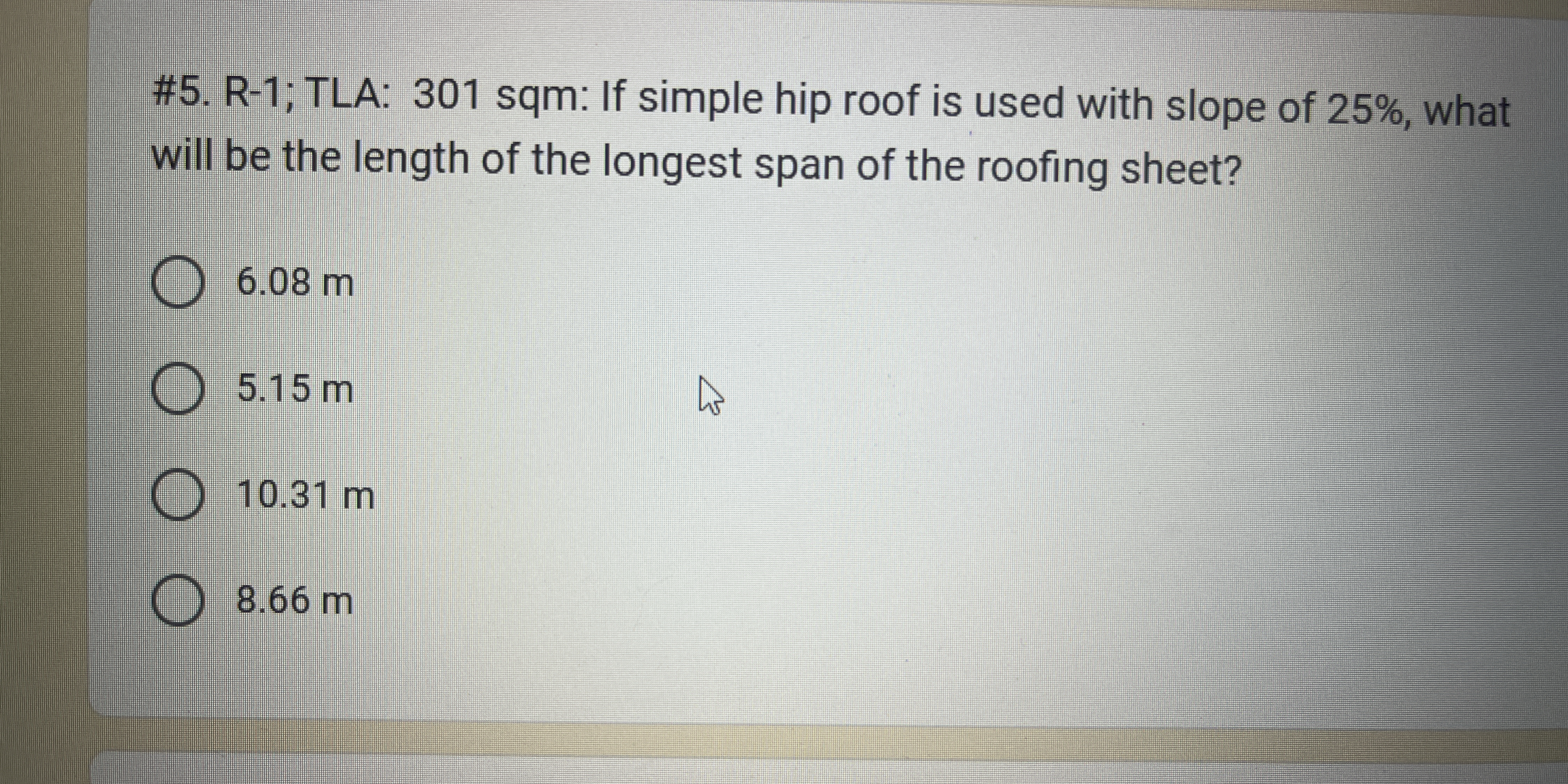
If a simple hip roof is used with a slope of 25%, what will be the length of the longest span of the roofing sheet?

Steps to Solve

1. Determine the Area of the Roof The area ( A ) of the hip roof is given as 301 square meters.

2. Calculate the Total Length of the Roof's Base For a simple hip roof, the area can be represented as: $$ A = \text{Base Length} \times \text{Height} $$

To find the base length, let ( l ) be the length of the base, and ( h ) be the height: $$ 301 = l \times h $$

3. Identify Relationship from Slope The slope of the roof is given as 25%. This means: $$ \tan(\theta) = \frac{\text{Vertical Rise}}{\text{Horizontal Run}} = 0.25 $$

From this, we can derive: $$ \theta = \tan^{-1}(0.25) $$

4. Determine Height using the Slope Knowing that: $$ h = l \cdot \tan(\theta) $$

Substituting this into the area equation from step 2: $$ 301 = l \cdot (l \cdot \tan(\theta)) $$

Thus rearranging gives: $$ l^2 \cdot \tan(\theta) = 301 $$

5. Solve for the Length of Base Using the previous equation: $$ l^2 = \frac{301}{\tan(\theta)} $$

6. Calculate the length of the longest span The span ( s ) of the roofing sheet can be calculated as follows: Using the Pythagorean theorem for half the base and the height: $$ s = \sqrt{ \left( \frac{l}{2} \right)^2 + h^2 } $$

With ( h = l \cdot \tan(\theta) ).

7. Insert values and calculate Now, calculate for ( l ) and then the span: $$ l = \sqrt{\frac{301}{\tan(\theta)}} $$

Calculate ( \theta ): $$ \theta \approx 14.04^\circ $$ (using a calculator for tan inverse)
$$ \tan(14.04^\circ) \approx 0.25 $$

This will yield the value of ( l ) and subsequently allow for calculation of ( s ).