Given the line -3x + 2y = 5, then its slope is ........ Choose the correct answer: (1) The reflection through the y axis of the point (-2, 3) is the point ....... (2) The distance... Given the line -3x + 2y = 5, then its slope is ........ Choose the correct answer: (1) The reflection through the y axis of the point (-2, 3) is the point ....... (2) The distance between P1 = (-2, 3) and P2 = (6, -3) is ........ (3) The midpoint M of the line segment joining P1 = (4, 6) and P2 = (-6, 8) is ....... (4) The equation of a circle with radius 3 and center at (-1, 2) is .......
Understand the Problem
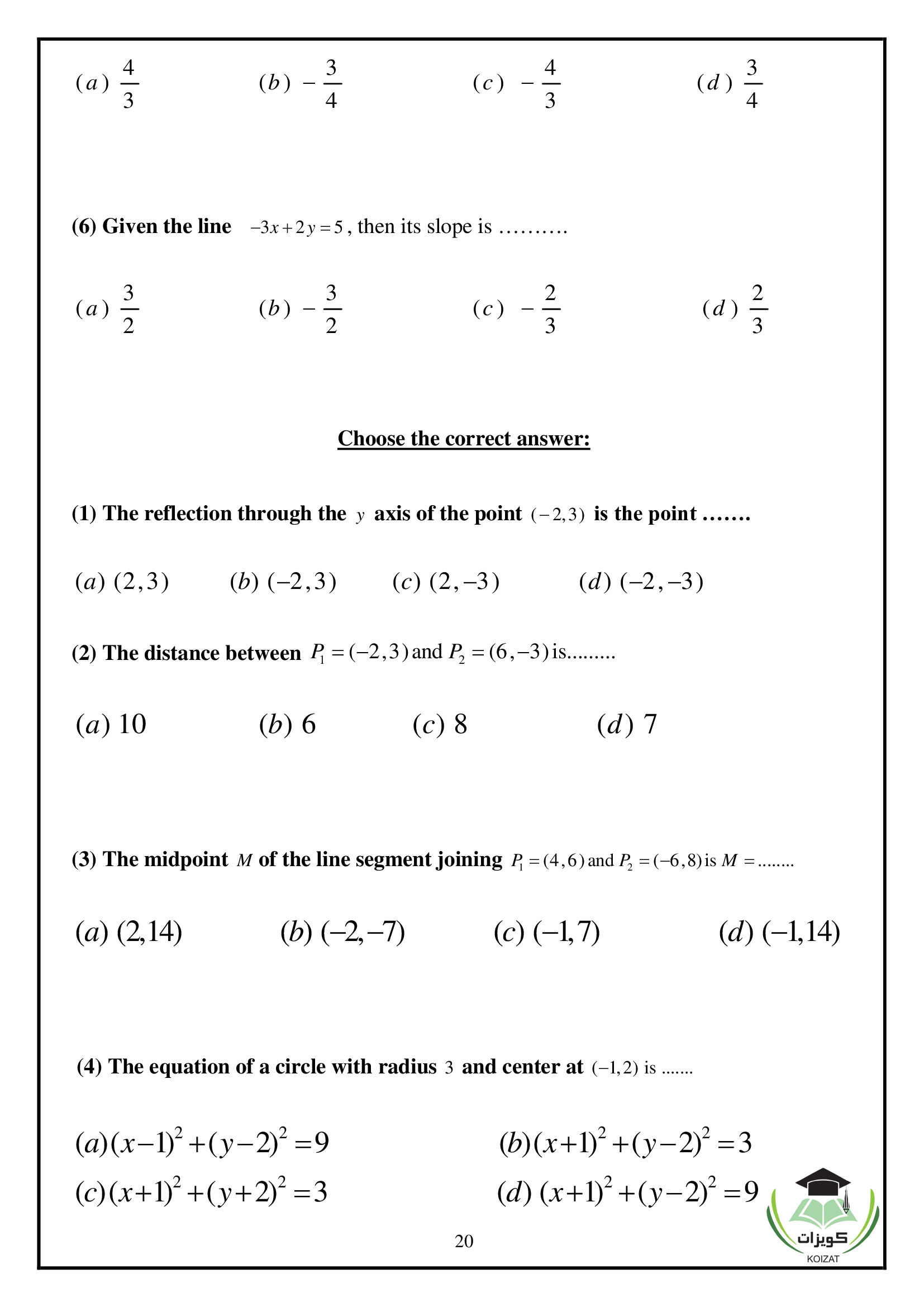
The image presents several math problems concerning slopes, reflections, distances, midpoints, and the equation of a circle, asking for the correct answers to each question.
Answer
1. $\frac{3}{2}$, 2. $(2, 3)$, 3. $10$, 4. $(-1, 7)$, 5. $(x + 1)^2 + (y - 2)^2 = 9$
Answer for screen readers
- The slope is $\frac{3}{2}$.
- The reflected point is $(2, 3)$.
- The distance is $10$.
- The midpoint is $(-1, 7)$.
- The equation of the circle is $(x + 1)^2 + (y - 2)^2 = 9$.
Steps to Solve
- Finding the slope of the line To find the slope of the line given by the equation $-3x + 2y = 5$, we can rearrange the equation into slope-intercept form ($y = mx + b$), where $m$ is the slope.
Starting with the given equation: $$ 2y = 3x + 5 $$
Dividing both sides by 2: $$ y = \frac{3}{2}x + \frac{5}{2} $$
Thus, the slope $m$ is $\frac{3}{2}$.
-
Reflecting a point through the y-axis The reflection of a point $(x, y)$ through the y-axis is given by $(-x, y)$. Thus, the reflection of the point $(-2, 3)$ is $(2, 3)$.
-
Calculating the distance between two points To find the distance between the points $P_1=(-2, 3)$ and $P_2=(6, -3)$, we use the distance formula: $$ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} $$
Substituting the coordinates: $$ d = \sqrt{(6 - (-2))^2 + (-3 - 3)^2} $$ $$ = \sqrt{(6 + 2)^2 + (-6)^2} $$ $$ = \sqrt{8^2 + 6^2} $$ $$ = \sqrt{64 + 36} $$ $$ = \sqrt{100} $$ $$ = 10 $$
- Finding the midpoint of a line segment The midpoint $M$ of a segment joining two points $P_1=(4, 6)$ and $P_2=(-6, 8)$ is calculated using: $$ M = \left( \frac{x_1 + x_2}{2}, \frac{y_1 + y_2}{2} \right) $$
Substituting the coordinates: $$ M = \left( \frac{4 + (-6)}{2}, \frac{6 + 8}{2} \right) $$ $$ = \left( \frac{-2}{2}, \frac{14}{2} \right) $$ $$ = (-1, 7) $$
- Writing the equation of a circle The standard form of a circle's equation is $(x - h)^2 + (y - k)^2 = r^2$, where $(h, k)$ is the center and $r$ is the radius. Here, the center is $(-1, 2)$ and the radius is $3$. Therefore, the equation is:
$$(x + 1)^2 + (y - 2)^2 = 9$$
- The slope is $\frac{3}{2}$.
- The reflected point is $(2, 3)$.
- The distance is $10$.
- The midpoint is $(-1, 7)$.
- The equation of the circle is $(x + 1)^2 + (y - 2)^2 = 9$.
More Information
These calculations involve basic concepts of geometry and algebra such as slopes, reflections, distances between points, midpoints, and the equation of a circle, which are all fundamental in coordinate geometry.
Tips
- Failing to rearrange the line equation correctly: Ensure to isolate $y$ to find the slope.
- Mistaking signs when reflecting points: Remember that reflecting over the y-axis changes the sign of the x-coordinate only.
- Using the distance formula incorrectly: Always ensure you’re using the correct coordinates for each point.
AI-generated content may contain errors. Please verify critical information