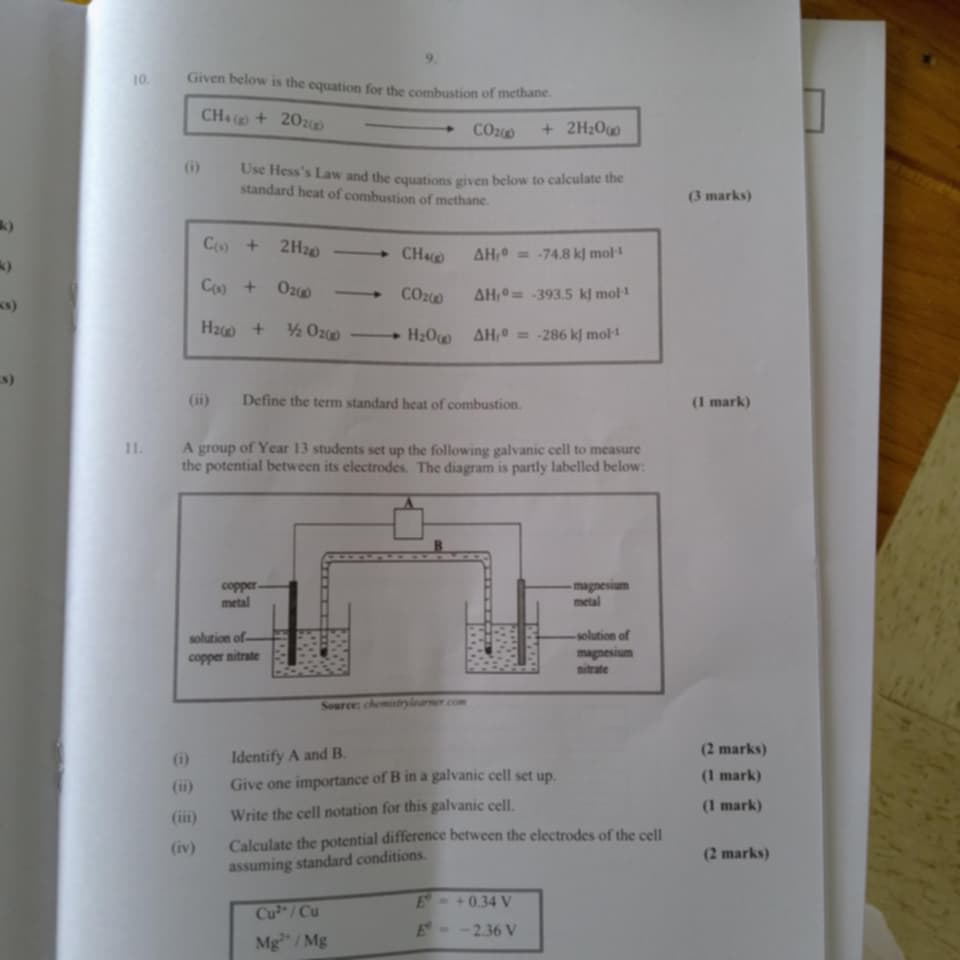
Given below is the equation for the combustion of methane. (i) Use Hess’s Law and the equations given below to calculate the standard heat of combustion of methane. (ii) Define the... Given below is the equation for the combustion of methane. (i) Use Hess’s Law and the equations given below to calculate the standard heat of combustion of methane. (ii) Define the term standard heat of combustion. A group of Year 13 students set up the following galvanic cell to measure the potential between its electrodes: Identify A and B. (ii) Give one importance of B in a galvanic cell set up. (iii) Write the cell notation for this galvanic cell. (iv) Calculate the potential difference between the electrodes of the cell assuming standard conditions.
Understand the Problem
The question is asking to calculate the standard heat of combustion of methane using Hess’s Law and provided equations, as well as to define the term 'standard heat of combustion.' It also involves identifying components of a galvanic cell, writing cell notation, and calculating the potential difference between electrodes.
Answer
The standard heat of combustion of methane is $$ -890.7 \text{ kJ mol}^{-1} $$.
Answer for screen readers
The standard heat of combustion of methane is $$ \Delta H^\circ_{\text{comb}}(\text{CH}_4) = -890.7 \text{ kJ mol}^{-1} $$.
Steps to Solve
-
Understanding the Given Data We have the combustion reaction of methane: $$ \text{CH}_4(g) + 2\text{O}_2(g) \rightarrow \text{CO}_2(g) + 2\text{H}_2\text{O}(l) $$ We are provided with the enthalpy changes for different reactions.
-
Applying Hess's Law Hess's Law states that the total enthalpy change of a reaction is equal to the sum of the enthalpy changes for individual steps. We need to manipulate the provided equations to obtain the reaction for methane combustion.
-
Manipulating Equations To achieve the equation for methane:
- Use the equation for the formation of $$\text{CO}_2$$ and $$\text{H}_2\text{O}$$
- Reverse the formation reaction for products (changing the sign of ΔH) when needed.
The relevant equations are:
- For water: $$ \text{H}_2(g) + ½ \text{O}_2(g) \rightarrow \text{H}_2\text{O}(l) \quad \Delta H^\circ = -286 \text{ kJ mol}^{-1} $$
- For carbon dioxide: $$ \text{C}(s) + \text{O}_2(g) \rightarrow \text{CO}_2(g) \quad \Delta H^\circ = -393.5 \text{ kJ mol}^{-1} $$
- Combining the Enthalpy Changes Combine the changes properly:
- Combine the enthalpy of combustion for methanol and the reversed enthalpies for the products.
Let:
- $ \Delta H^\circ_{\text{comb}}(\text{CH}_4) $ be what we want to find,
Then: $$ \Delta H^\circ_{\text{comb}}(\text{CH}4) = [\Delta H^\circ{\text{f}}(\text{CO}2) + 2\Delta H^\circ{\text{f}}(\text{H}2\text{O})] - \Delta H^\circ{\text{f}}(\text{CH}_4) $$
- Calculating the Values Substituting the known values into the equation:
- $$ \Delta H^\circ_{\text{comb}}(\text{CH}_4) = [-393.5 + 2(-286)] - (-74.8) $$
Calculating this: $$ \Delta H^\circ_{\text{comb}}(\text{CH}4) = -393.5 - 572 + 74.8 $$ $$ \Delta H^\circ{\text{comb}}(\text{CH}_4) = -890.7 \text{ kJ mol}^{-1} $$
- Defining Standard Heat of Combustion Standard heat of combustion refers to the enthalpy change when one mole of a substance combusts completely in oxygen under standard conditions.
The standard heat of combustion of methane is $$ \Delta H^\circ_{\text{comb}}(\text{CH}_4) = -890.7 \text{ kJ mol}^{-1} $$.
More Information
The standard heat of combustion is a crucial value in thermodynamics, important for understanding energy releases in combustion reactions. Methane is often used as a standard for comparing energy outputs of different fuels.
Tips
- Neglecting Sign Changes: When reversing reactions, remember to change the sign of ΔH.
- Incorrectly Combining Enthalpy Values: Make sure to follow the stoichiometry carefully.
AI-generated content may contain errors. Please verify critical information