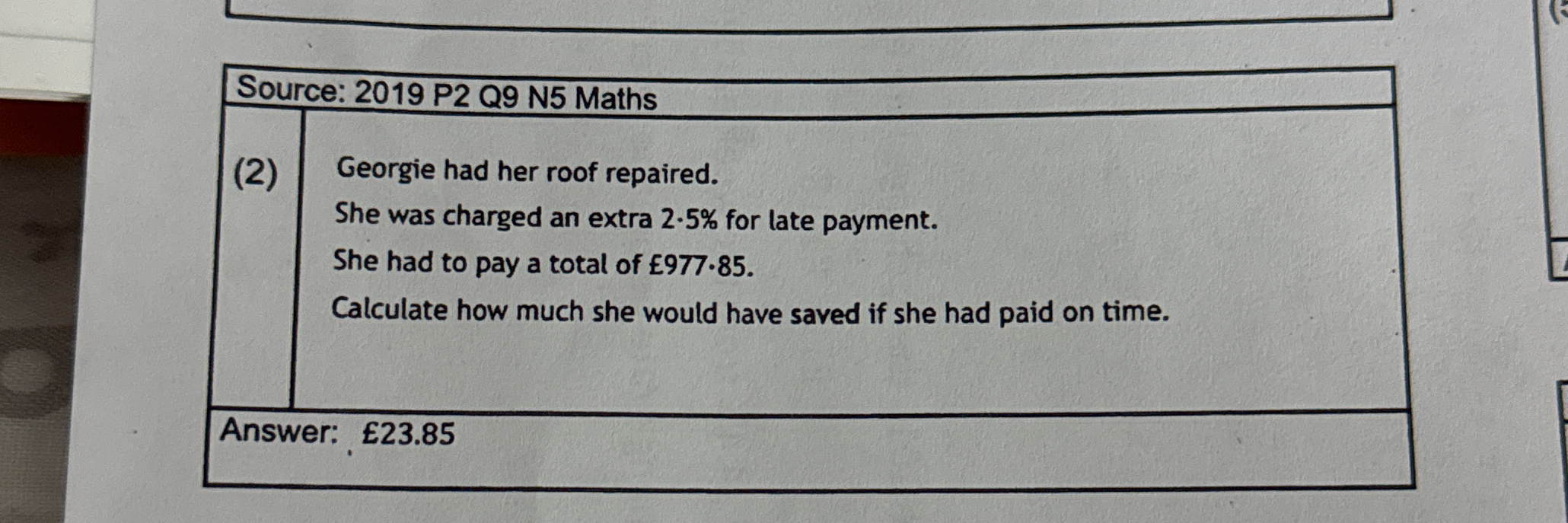
Georgie had her roof repaired. She was charged an extra 2.5% for late payment. She had to pay a total of £977.85. Calculate how much she would have saved if she had paid on time.
Understand the Problem
The question involves calculating the extra charge Georgie incurred due to late payment on her roof repairs. She was charged an additional 2.5% on the total bill. The task is to find out how much she would have saved had she paid on time.
Answer
£23.85
Answer for screen readers
The amount Georgie would have saved had she paid on time is £23.85.
Steps to Solve
- Identify the total bill without the late charge
Let the original amount (without the late charge) be represented as $x$.
The additional charge is 2.5% of the original amount: $$ \text{Total charge} = x + 0.025x = 1.025x $$
- Set up the equation based on the total amount paid
According to the problem, Georgie paid a total of £977.85. Therefore: $$ 1.025x = 977.85 $$
- Solve for the original amount
Now, we can isolate $x$ by dividing both sides by 1.025: $$ x = \frac{977.85}{1.025} \approx 955.24 $$
- Calculate the extra charge incurred
Now, to find the extra charge incurred due to the late payment, we can calculate 2.5% of the original amount: $$ \text{Extra charge} = 0.025 \times 955.24 \approx 23.85 $$
The amount Georgie would have saved had she paid on time is £23.85.
More Information
This calculation reflects the additional cost incurred due to a late payment, which highlights how even small percentages can add up to significant amounts.
Tips
- Miscalculating the percentage increase; ensure that you properly convert the percentage to decimal form (e.g., 2.5% = 0.025).
- Forgetting to use the increased amount when solving for the original bill; always set the total correctly in your equations.
AI-generated content may contain errors. Please verify critical information