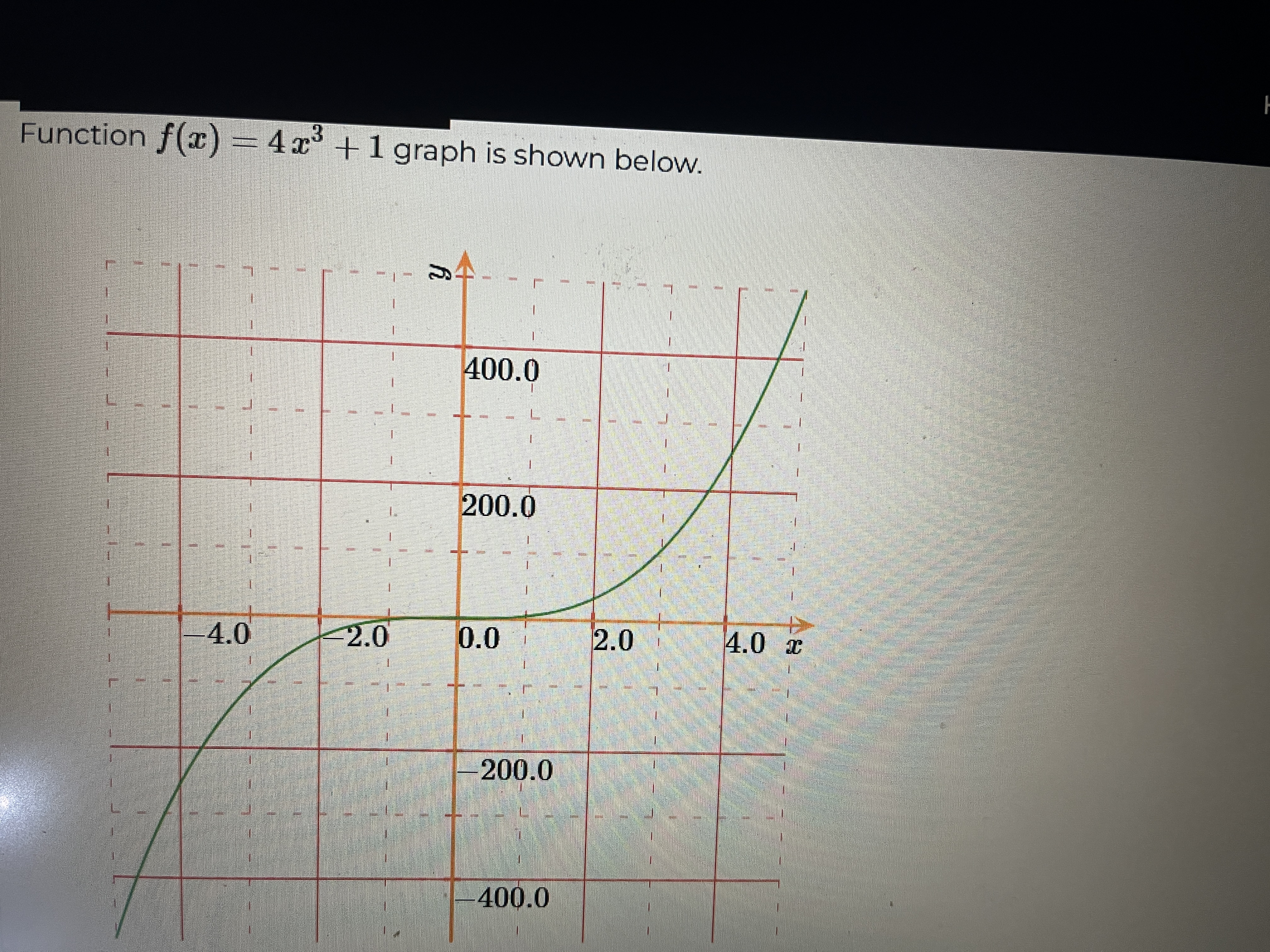
Function f(x) = 4x³ + 1 graph is shown below. What can be inferred from it?
Understand the Problem
The question is presenting a cubic function f(x) = 4x³ + 1 along with its graph, likely seeking an analysis or specific details regarding its behavior, intercepts, or characteristics.
Answer
Y-intercept: (0, 1); x-intercept: $\left(-\frac{1}{\sqrt[3]{4}}, 0\right)$; local minimum at (0, 1).
Answer for screen readers
The cubic function $f(x) = 4x^3 + 1$ has a y-intercept at (0, 1) and an x-intercept at $\left(-\frac{1}{\sqrt[3]{4}}, 0\right)$. It increases from left to right with a local minimum at (0, 1).
Steps to Solve
- Identify the function and its components
The given function is $f(x) = 4x^3 + 1$. This is a cubic function where the leading coefficient is positive, indicating it will extend upward as $x$ approaches both positive and negative infinity.
- Determine the y-intercept
To find the y-intercept, set $x = 0$:
$$ f(0) = 4(0)^3 + 1 = 1 $$
Thus, the y-intercept is at the point (0, 1).
- Find the x-intercepts
To find the x-intercepts, set $f(x) = 0$:
$$ 4x^3 + 1 = 0 $$
Solving for $x$:
$$ 4x^3 = -1 $$
$$ x^3 = -\frac{1}{4} $$
Taking the cube root:
$$ x = -\left(\frac{1}{4}\right)^{1/3} $$
The x-intercept is at the point $\left(-\frac{1}{\sqrt[3]{4}}, 0\right)$.
- Analyze the behavior of the function
As $x \rightarrow +\infty$, $f(x) \rightarrow +\infty$, and as $x \rightarrow -\infty$, $f(x) \rightarrow -\infty$. This shows that the ends of the graph rise on both sides.
- Determine local extrema
To find critical points, calculate the derivative:
$$ f'(x) = 12x^2 $$
Setting the derivative to zero:
$$ 12x^2 = 0 $$
The only critical point is at $x = 0$. To determine whether it is a maximum or minimum,
- For $x < 0$, $f'(x) < 0$ (decreasing)
- For $x > 0$, $f'(x) > 0$ (increasing)
Thus, there is a local minimum at $(0, 1)$.
The cubic function $f(x) = 4x^3 + 1$ has a y-intercept at (0, 1) and an x-intercept at $\left(-\frac{1}{\sqrt[3]{4}}, 0\right)$. It increases from left to right with a local minimum at (0, 1).
More Information
Cubic functions have a characteristic "S" shaped graph. This specific function rises steeply in both directions, indicating its strong growth as $x$ moves away from zero. The behavior at the intercepts provides key insights into how the function interacts with the axes.
Tips
- Miscalculating the x-intercept; it is important to correctly simplify expressions when solving for zeroes of the function.
- Not analyzing the behavior of the function at critical points or misunderstanding maxima and minima.