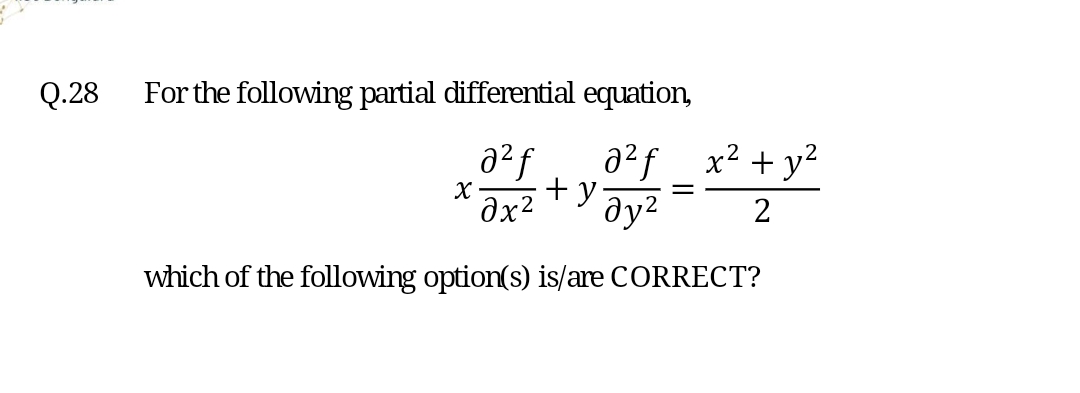
For the following partial differential equation, x ∂²f/∂x² + y ∂²f/∂y² = (x² + y²)/2, which of the following option(s) is/are CORRECT?

Steps to Solve

1. Identify the Given Differential Equation We have the partial differential equation (PDE): $$ x \frac{\partial^2 f}{\partial x^2} + y \frac{\partial^2 f}{\partial y^2} = \frac{x^2 + y^2}{2} $$

2. Analyze the Homogeneous Part The homogeneous version of this equation is: $$ x \frac{\partial^2 f}{\partial x^2} + y \frac{\partial^2 f}{\partial y^2} = 0 $$ This part can provide insights into the solutions.

3. Look for Particular Solutions To find a particular solution, we can assume a form like: $$ f(x, y) = A(x^2 + y^2) $$ where ( A ) is constant or a function of ( x ) and ( y ).

4. Calculate the Second Derivatives Compute the second derivatives: $$ \frac{\partial^2 f}{\partial x^2} = 2A $$ $$ \frac{\partial^2 f}{\partial y^2} = 2A $$ Substitute these back into the original equation.

5. Substitute Back into the PDE Substituting gives: $$ x(2A) + y(2A) = \frac{x^2 + y^2}{2} $$ This simplifies to: $$ 2A(x + y) = \frac{x^2 + y^2}{2} $$

6. Match Coefficients Solve for the coefficient ( A ) by matching terms:

   • From the left-hand side ( 2A(x + y) )
   • Right-hand side suggests comparing these terms to find a relation for ( A ).

7. Conclusion Determine the correct options based on the analysis of the particular solution ( f(x, y) ) against the options provided.