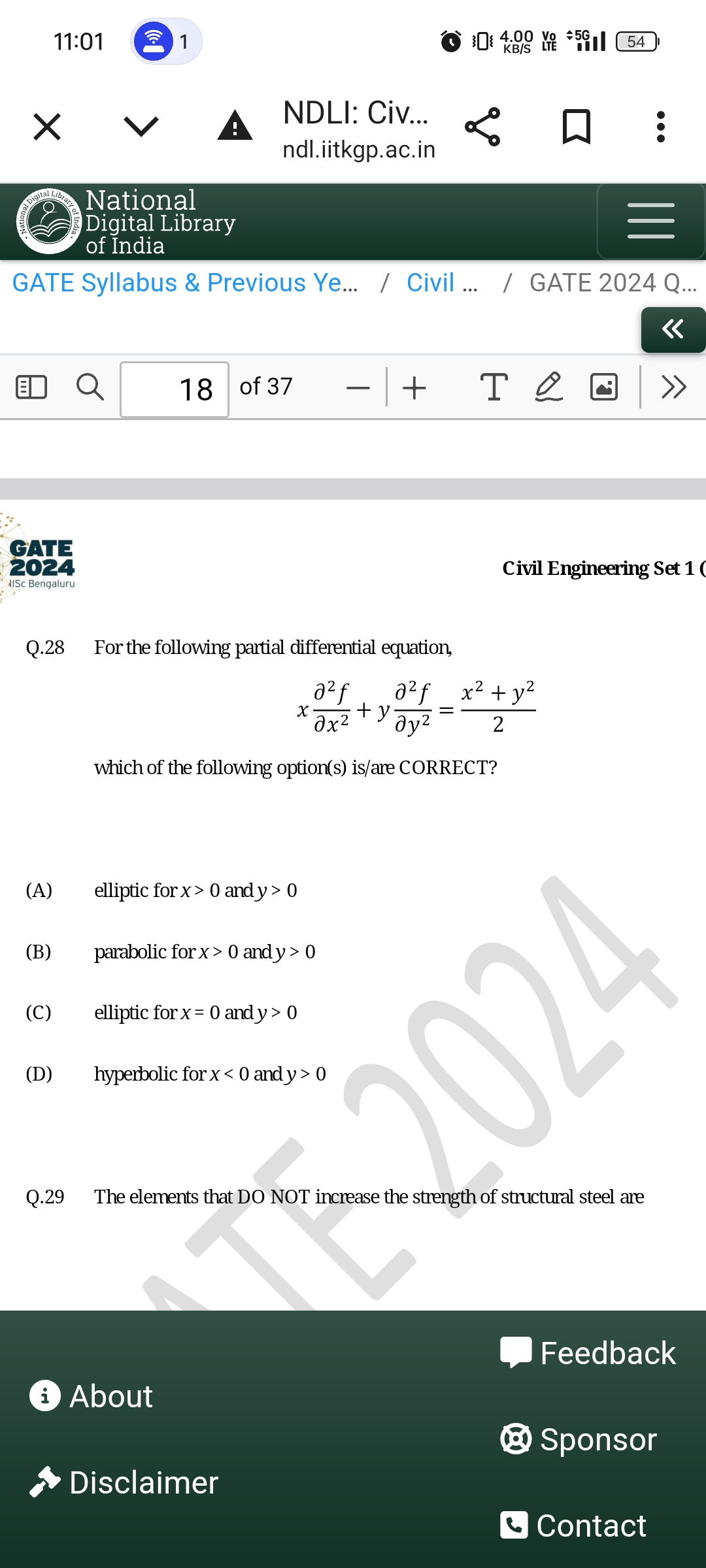
For the following partial differential equation, x ∂²f/∂x² + y ∂²f/∂y² = (x² + y²) / 2, which of the following option(s) is/are CORRECT? (A) elliptic for x > 0 and y > 0 (B) parabo... For the following partial differential equation, x ∂²f/∂x² + y ∂²f/∂y² = (x² + y²) / 2, which of the following option(s) is/are CORRECT? (A) elliptic for x > 0 and y > 0 (B) parabolic for x > 0 and y > 0 (C) elliptical for x = 0 and y > 0 (D) hyperbolic for x < 0 and y > 0 Read more

Steps to Solve

1. Identify the characteristics of the PDE The given partial differential equation (PDE) is:
   $$ x \frac{\partial^2 f}{\partial x^2} + y \frac{\partial^2 f}{\partial y^2} = \frac{x^2 + y^2}{2} $$

This equation can be analyzed based on the coefficients of the second derivatives.

2. Classify the PDE based on the conditions To classify the PDE, we examine the sign of the coefficients of the second-order derivatives in standard form:
   $$ A \frac{\partial^2 f}{\partial x^2} + B \frac{\partial^2 f}{\partial y^2} $$
   Where $A = x$ and $B = y$.

3. Determine the nature for specific regions

• For $x > 0$ and $y > 0$, both $A > 0$ and $B > 0$: The equation is elliptic.
• For $x = 0$ (regardless of $y$): Analyze the equation as it becomes $0 + y \frac{\partial^2 f}{\partial y^2}$, which is parabolic if $y > 0$.
• For $x < 0$ and $y > 0$: Here, $A < 0$, which means the equation becomes hyperbolic.

4. Summarize findings Based on the analysis:

• Option (A) is correct: elliptic for $x > 0$ and $y > 0$.
• Option (B) is incorrect: it is elliptic, not parabolic.
• Option (C) is correct: it is parabolic for $x = 0$ and $y > 0$.
• Option (D) is correct: it is hyperbolic for $x < 0$ and $y > 0$.