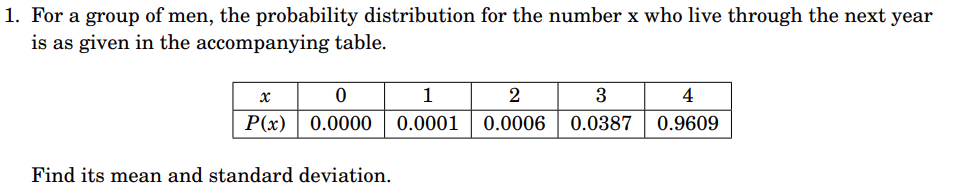
For a group of men, the probability distribution for the number x who live through the next year is as given in the accompanying table. Find its mean and standard deviation.

Steps to Solve

1. Calculating the Mean

To find the mean ($\mu$), we will use the formula:

$$ \mu = \sum (x \cdot P(x)) $$

Now, we calculate it by multiplying each $x$ value with its corresponding probability and summing these products.

[ \mu = (0 \cdot 0.0000) + (1 \cdot 0.0001) + (2 \cdot 0.0006) + (3 \cdot 0.0387) + (4 \cdot 0.9609) ]

Calculating each term:

• For $x=0$: $0 \cdot 0.0000 = 0$
• For $x=1$: $1 \cdot 0.0001 = 0.0001$
• For $x=2$: $2 \cdot 0.0006 = 0.0012$
• For $x=3$: $3 \cdot 0.0387 = 0.1161$
• For $x=4$: $4 \cdot 0.9609 = 3.8436$

Now, summing these values:

[ \mu = 0 + 0.0001 + 0.0012 + 0.1161 + 3.8436 = 3.9609 ]

2. Calculating the Variance

Next, we calculate the variance ($\sigma^2$) using the formula:

$$ \sigma^2 = \sum ((x - \mu)^2 \cdot P(x)) $$

We will substitute $\mu = 3.9609$ into the equation:

[ \sigma^2 = (0 - 3.9609)^2 \cdot 0.0000 + (1 - 3.9609)^2 \cdot 0.0001 + (2 - 3.9609)^2 \cdot 0.0006 + (3 - 3.9609)^2 \cdot 0.0387 + (4 - 3.9609)^2 \cdot 0.9609 ]

Calculating each term:

• For $x=0$: $(0 - 3.9609)^2 \cdot 0.0000 = 0$
• For $x=1$: $(1 - 3.9609)^2 \cdot 0.0001 = (2.9609)^2 \cdot 0.0001 = 8.7608 \cdot 0.0001 = 0.00087608$
• For $x=2$: $(2 - 3.9609)^2 \cdot 0.0006 = (1.9609)^2 \cdot 0.0006 = 3.8417 \cdot 0.0006 = 0.00230488$
• For $x=3$: $(3 - 3.9609)^2 \cdot 0.0387 = (0.9609)^2 \cdot 0.0387 = 0.9233 \cdot 0.0387 = 0.03575451$
• For $x=4$: $(4 - 3.9609)^2 \cdot 0.9609 = (0.0391)^2 \cdot 0.9609 = 0.00152961 \cdot 0.9609 = 0.001469132$

Summing these values:

[ \sigma^2 = 0 + 0.00087608 + 0.00230488 + 0.03575451 + 0.001469132 = 0.04040458 ]

3. Calculating the Standard Deviation

Finally, we calculate the standard deviation ($\sigma$) by taking the square root of the variance:

$$ \sigma = \sqrt{\sigma^2} $$

Calculating it:

[ \sigma = \sqrt{0.04040458} \approx 0.201 ]