Find the solution set for the following equation: 6 - 4 / (3 - 6 / x) = 2.
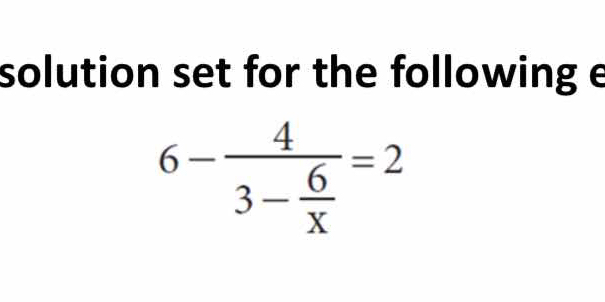
Understand the Problem
The question is asking for the solution set of a given algebraic equation that involves a fraction. This requires solving for the variable x.
Answer
The solution set is $x = 3$.
Answer for screen readers
The solution set for the equation is $x = 3$.
Steps to Solve
-
Isolate the fraction Start by isolating the fraction on one side of the equation. We move 6 to the other side: $$ -\frac{4}{3 - \frac{6}{x}} = 2 - 6 $$ This simplifies to: $$ -\frac{4}{3 - \frac{6}{x}} = -4 $$
-
Eliminate the negative sign Multiply both sides by -1 to make the equation easier to work with: $$ \frac{4}{3 - \frac{6}{x}} = 4 $$
-
Cross-multiply to eliminate the fraction Next, cross-multiply to eliminate the fraction: $$ 4 = 4(3 - \frac{6}{x}) $$
-
Distribute on the right side Distributing gives us: $$ 4 = 12 - \frac{24}{x} $$
-
Rearrange the equation Rearranging this equation to isolate the term with $x$: $$ \frac{24}{x} = 12 - 4 $$ So, $$ \frac{24}{x} = 8 $$
-
Cross-multiply again Cross-multiplying gives: $$ 24 = 8x $$
-
Solve for x Now, divide both sides by 8: $$ x = \frac{24}{8} = 3 $$
The solution set for the equation is $x = 3$.
More Information
The solution found, $x = 3$, satisfies the original equation. To verify, you can substitute $x$ back into the equation and check for equality.
Tips
- Failing to correctly isolate the fraction can lead to confusion.
- Neglecting to multiply out the right side properly during distribution.
AI-generated content may contain errors. Please verify critical information