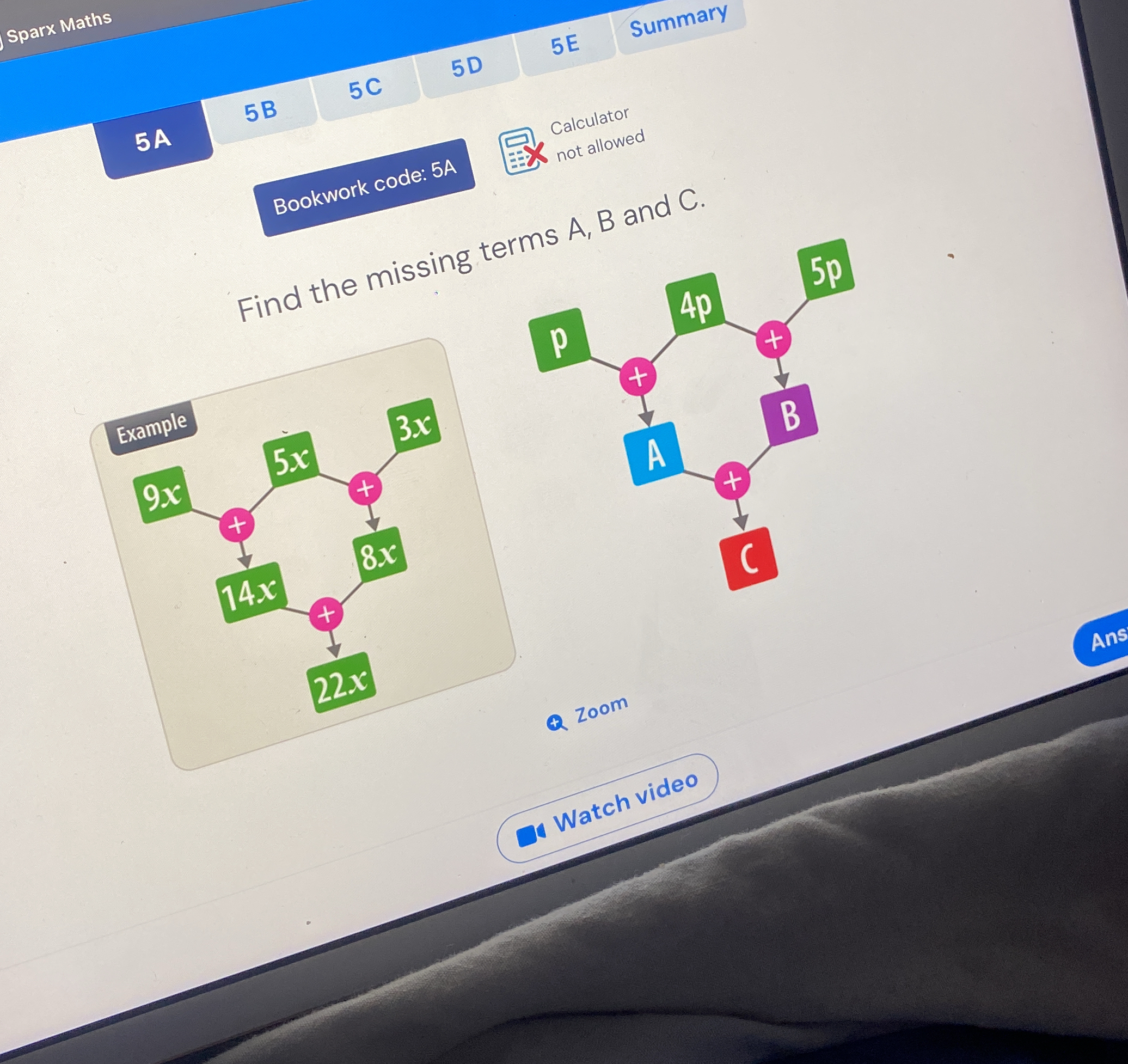
Find the missing terms A, B and C.
Understand the Problem
The question requires us to find the missing algebraic terms A, B, and C in the provided structure based on the relationships indicated by the other terms and their sums.
Answer
The missing terms are $A = 5p$, $B = -2p$, and $C = 6p$.
Answer for screen readers
The missing terms are:
- $A = 5p$
- $B = -2p$
- $C = 6p$
Steps to Solve
- Identify Terms and Relationships
From the diagram, we have the terms $p$, $4p$, and $5p$. These terms need to relate to $A$ and $C$. The structure indicates that $A = p + 4p$ and $C = p + 5p$.
- Simplify the Expression for A
To find $A$, we will add $p$ and $4p$ together:
$$ A = p + 4p = 5p $$
- Simplify the Expression for C
Next, to find $C$, we will add $p$ and $5p$ together:
$$ C = p + 5p = 6p $$
- Identify the Value of B
Now we need to relate the values of $A$ and $C$ to find $B$. From the structure above, we see that:
$$ A + B + C = 4p + 5p $$
So combining $A$ and $C$:
$$ 5p + B + 6p = 9p $$
This implies:
$$ B = 9p - (5p + 6p) = 9p - 11p = -2p $$
- Final Summary of Values
Thus, we now have:
- $A = 5p$
- $B = -2p$
- $C = 6p$
The missing terms are:
- $A = 5p$
- $B = -2p$
- $C = 6p$
More Information
This problem illustrates how to manipulate algebraic terms using basic addition. It’s an example of how relations between terms can lead to the discovery of unknown values.
Tips
- Forgetting to combine like terms correctly can lead to errors.
- Neglecting to check the overall equation after solving for the unknowns can also result in mistakes.
AI-generated content may contain errors. Please verify critical information